Proof Of Derivative Of Inverse Trig Functions
News Co
Apr 07, 2025 · 5 min read
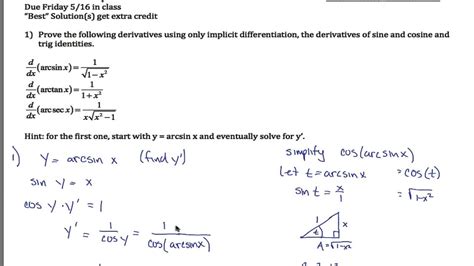
Table of Contents
Proof of Derivatives of Inverse Trigonometric Functions
The inverse trigonometric functions, also known as arcus functions or cyclometric functions, are the inverse functions of the trigonometric functions. Understanding their derivatives is crucial in various fields, including calculus, physics, and engineering. This article provides a comprehensive guide to proving the derivatives of the six inverse trigonometric functions: arcsin x, arccos x, arctan x, arccot x, arcsec x, and arccsc x. We will utilize implicit differentiation, a powerful technique for finding derivatives of implicitly defined functions.
1. Derivative of arcsin x
Let y = arcsin x. This means that sin y = x. We'll use implicit differentiation to find dy/dx.
Step 1: Implicit Differentiation
Differentiating both sides of sin y = x with respect to x, we get:
cos y (dy/dx) = 1
Step 2: Solving for dy/dx
Solving for dy/dx, we obtain:
dy/dx = 1 / cos y
Step 3: Expressing in terms of x
Since sin y = x, we can use the Pythagorean identity sin²y + cos²y = 1 to express cos y in terms of x:
cos²y = 1 - sin²y = 1 - x²
Therefore, cos y = ±√(1 - x²)
Step 4: Determining the sign
The range of arcsin x is [-π/2, π/2]. In this interval, cos y is always non-negative. Thus, we choose the positive square root:
cos y = √(1 - x²)
Step 5: Final Result
Substituting this back into our expression for dy/dx, we get the derivative of arcsin x:
d(arcsin x)/dx = 1 / √(1 - x²)
2. Derivative of arccos x
Following a similar process, let y = arccos x, which implies cos y = x.
Step 1: Implicit Differentiation
Differentiating both sides with respect to x:
-sin y (dy/dx) = 1
Step 2: Solving for dy/dx
dy/dx = -1 / sin y
Step 3: Expressing in terms of x
Using the Pythagorean identity, sin²y = 1 - cos²y = 1 - x², we have sin y = ±√(1 - x²)
Step 4: Determining the sign
The range of arccos x is [0, π]. In this interval, sin y is always non-negative. Therefore, we choose the positive square root:
sin y = √(1 - x²)
Step 5: Final Result
Substituting into the expression for dy/dx, we find the derivative of arccos x:
d(arccos x)/dx = -1 / √(1 - x²)
3. Derivative of arctan x
Let y = arctan x, implying tan y = x.
Step 1: Implicit Differentiation
Differentiating both sides with respect to x:
sec²y (dy/dx) = 1
Step 2: Solving for dy/dx
dy/dx = 1 / sec²y
Step 3: Expressing in terms of x
Using the identity sec²y = 1 + tan²y = 1 + x², we get:
dy/dx = 1 / (1 + x²)
Step 4: Final Result
The derivative of arctan x is:
d(arctan x)/dx = 1 / (1 + x²)
4. Derivative of arccot x
Let y = arccot x, so cot y = x.
Step 1: Implicit Differentiation
Differentiating with respect to x:
-csc²y (dy/dx) = 1
Step 2: Solving for dy/dx
dy/dx = -1 / csc²y
Step 3: Expressing in terms of x
Using the identity csc²y = 1 + cot²y = 1 + x², we have:
dy/dx = -1 / (1 + x²)
Step 4: Final Result
The derivative of arccot x is:
d(arccot x)/dx = -1 / (1 + x²)
5. Derivative of arcsec x
Let y = arcsec x, meaning sec y = x.
Step 1: Implicit Differentiation
Differentiating with respect to x:
sec y tan y (dy/dx) = 1
Step 2: Solving for dy/dx
dy/dx = 1 / (sec y tan y)
Step 3: Expressing in terms of x
We know sec y = x. Using the identity tan²y = sec²y - 1 = x² - 1, we get tan y = ±√(x² - 1).
Step 4: Determining the sign
The range of arcsec x is [0, π], excluding π/2. For x > 1, tan y is positive, and for x < -1, tan y is negative. Therefore:
tan y = √(x² - 1) for x > 1 tan y = -√(x² - 1) for x < -1
Step 5: Final Result
Combining this with sec y = x, we get the derivative of arcsec x:
d(arcsec x)/dx = 1 / (|x|√(x² - 1))
6. Derivative of arccsc x
Let y = arccsc x, which implies csc y = x.
Step 1: Implicit Differentiation
Differentiating with respect to x:
-csc y cot y (dy/dx) = 1
Step 2: Solving for dy/dx
dy/dx = -1 / (csc y cot y)
Step 3: Expressing in terms of x
We have csc y = x. Using the identity cot²y = csc²y - 1 = x² - 1, we get cot y = ±√(x² - 1).
Step 4: Determining the sign
The range of arccsc x is [-π/2, π/2], excluding 0. For x > 1, cot y is positive, and for x < -1, cot y is negative. Therefore:
cot y = √(x² - 1) for x > 1 cot y = -√(x² - 1) for x < -1
Step 5: Final Result
Combining this with csc y = x, we obtain the derivative of arccsc x:
d(arccsc x)/dx = -1 / (|x|√(x² - 1))
Conclusion
This article comprehensively detailed the derivations of all six inverse trigonometric functions using implicit differentiation. Understanding these derivatives is fundamental for advanced calculus and its applications. Remember to always consider the range of each inverse trigonometric function to correctly determine the sign of trigonometric expressions when expressing them in terms of x. This ensures accuracy and avoids errors in your calculations. Mastering these derivations solidifies your foundation in calculus and opens doors to tackling more complex problems involving inverse trigonometric functions. By understanding the underlying principles and steps involved, you can confidently apply these derivatives in diverse mathematical and scientific contexts. Consistent practice and a strong understanding of trigonometric identities are key to mastering these concepts.
Latest Posts
Related Post
Thank you for visiting our website which covers about Proof Of Derivative Of Inverse Trig Functions . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.