What Are The Factors For 46
News Co
Apr 05, 2025 · 5 min read
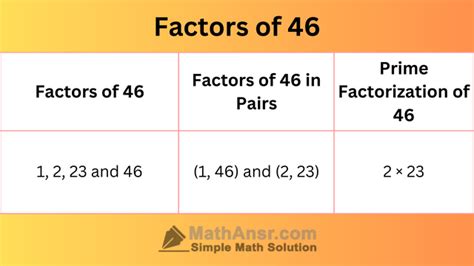
Table of Contents
Decoding the Factors of 46: A Deep Dive into Number Theory
The seemingly simple question, "What are the factors of 46?" opens a door to a fascinating exploration of number theory, a branch of mathematics that delves into the properties of numbers. While finding the factors of 46 might seem trivial, understanding the underlying concepts and their applications broadens our mathematical understanding significantly. This article will not only answer the question directly but also delve into the broader context of factors, prime factorization, and their importance in various mathematical fields.
What are Factors?
Before we tackle the factors of 46, let's define what a factor is. A factor (or divisor) of a number is a whole number that divides the number exactly without leaving a remainder. In simpler terms, if we can divide a number by another number and get a whole number result, then the second number is a factor of the first. For example, the factors of 12 are 1, 2, 3, 4, 6, and 12, because each of these numbers divides 12 evenly.
Finding the Factors of 46: A Step-by-Step Approach
Now, let's find the factors of 46. We can systematically approach this by checking each whole number from 1 up to 46 to see if it divides 46 without a remainder.
- 1: 46 ÷ 1 = 46 (1 is a factor)
- 2: 46 ÷ 2 = 23 (2 is a factor)
- 23: 46 ÷ 23 = 2 (23 is a factor)
- 46: 46 ÷ 46 = 1 (46 is a factor)
Therefore, the factors of 46 are 1, 2, 23, and 46. Notice that factors always come in pairs. This is because if 'a' is a factor of 'n', then 'n/a' is also a factor.
Prime Factorization: Unveiling the Building Blocks
The concept of prime factorization is closely related to finding factors. A prime number is a whole number greater than 1 that has only two factors: 1 and itself. Examples of prime numbers include 2, 3, 5, 7, 11, and so on. Prime factorization is the process of expressing a number as a product of its prime factors. This is a unique representation for every number (excluding 1).
To find the prime factorization of 46, we can use a factor tree:
46
/ \
2 23
Since 2 and 23 are both prime numbers, the prime factorization of 46 is 2 x 23. This means that 46 can be built solely from the prime numbers 2 and 23. This factorization is unique to 46; no other combination of prime numbers will multiply to give 46.
The Significance of Factors and Prime Factorization
Understanding factors and prime factorization is crucial in various areas of mathematics and beyond:
-
Simplifying Fractions: Finding the greatest common factor (GCF) of the numerator and denominator allows us to simplify fractions to their lowest terms. For instance, simplifying 46/92 requires finding the GCF of 46 and 92, which is 46. Therefore, 46/92 simplifies to 1/2.
-
Solving Algebraic Equations: Factorization plays a crucial role in solving quadratic and other polynomial equations. Factoring an equation helps us find its roots (the values that make the equation equal to zero).
-
Cryptography: Prime numbers and their properties are fundamental to modern cryptography, which secures online communication and transactions. The difficulty of factoring very large numbers into their prime components forms the basis of many encryption algorithms.
-
Number Theory Research: Factors and prime factorization are central concepts in number theory, leading to advanced research in areas like prime number distribution, perfect numbers, and the Riemann hypothesis.
-
Computer Science: Algorithms for finding prime factors are used in various computer science applications, including data compression, hashing, and random number generation.
Beyond 46: Exploring Other Numbers and Their Factors
Let's extend our understanding by exploring the factors of other numbers:
- Factors of 100: 1, 2, 4, 5, 10, 20, 25, 50, 100. Prime factorization: 2² x 5².
- Factors of 120: 1, 2, 3, 4, 5, 6, 8, 10, 12, 15, 20, 24, 30, 40, 60, 120. Prime factorization: 2³ x 3 x 5.
- Factors of 252: 1, 2, 3, 4, 6, 7, 9, 12, 14, 18, 21, 28, 36, 42, 63, 84, 126, 252. Prime factorization: 2² x 3² x 7.
Notice the patterns and how the prime factorization provides a concise representation of a number's structure.
Advanced Concepts Related to Factors
Several advanced concepts build upon the foundational understanding of factors:
- Greatest Common Factor (GCF): The largest number that divides two or more numbers without leaving a remainder.
- Least Common Multiple (LCM): The smallest number that is a multiple of two or more numbers.
- Divisibility Rules: Shortcuts for determining whether a number is divisible by certain integers (e.g., a number is divisible by 3 if the sum of its digits is divisible by 3).
- Perfect Numbers: A number that is equal to the sum of its proper divisors (divisors excluding the number itself). 6 is the first perfect number (1 + 2 + 3 = 6).
Conclusion: The Enduring Importance of Factors
The seemingly simple task of finding the factors of 46 opens a gateway to a vast and rich mathematical landscape. Understanding factors and prime factorization is not just about finding numbers; it's about understanding the fundamental building blocks of numbers, their relationships, and their profound implications across diverse fields. From simplifying fractions to securing online transactions, the concepts explored here lay the groundwork for many advanced mathematical concepts and real-world applications. The journey of exploring numbers and their properties is an ongoing adventure, and 46, with its simple factorization, serves as a perfect starting point for this exciting journey.
Latest Posts
Related Post
Thank you for visiting our website which covers about What Are The Factors For 46 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.