What Are The Prime Factors Of 175
News Co
May 07, 2025 · 5 min read
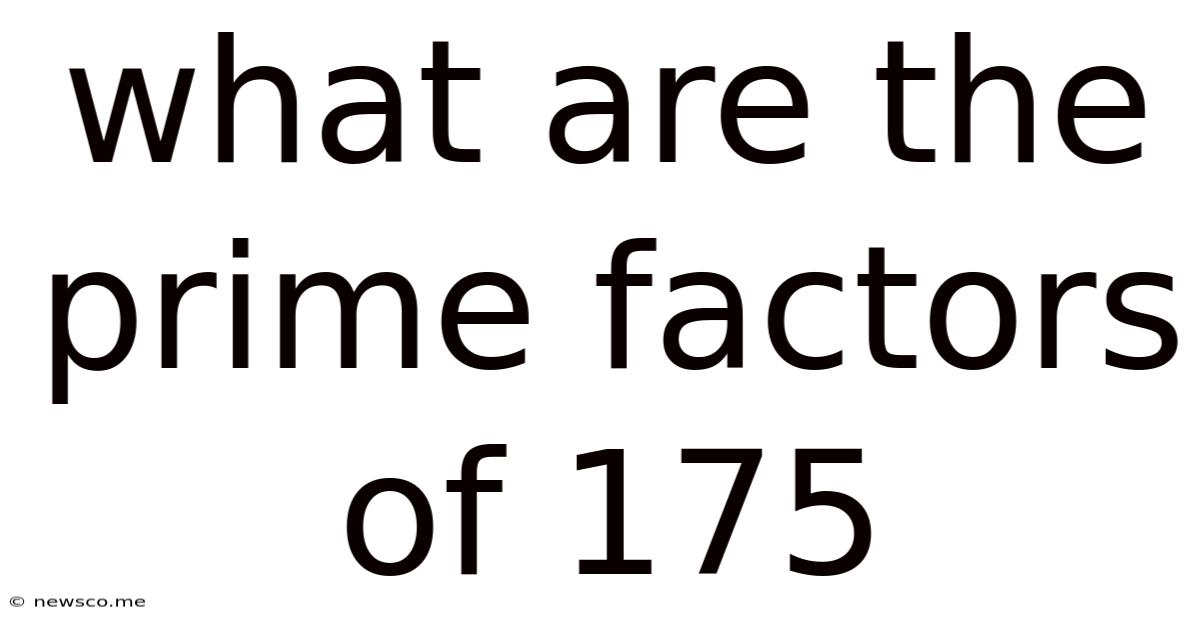
Table of Contents
What are the Prime Factors of 175? A Deep Dive into Prime Factorization
Finding the prime factors of a number might seem like a simple math problem, but understanding the process unlocks a deeper understanding of number theory and has practical applications in various fields, from cryptography to computer science. This article will not only answer the question, "What are the prime factors of 175?" but also explore the underlying concepts and techniques involved in prime factorization, providing a comprehensive guide suitable for both beginners and those seeking a refresher.
Understanding Prime Numbers and Prime Factorization
Before we delve into the prime factors of 175, let's establish a solid foundation.
What are Prime Numbers?
A prime number is a natural number greater than 1 that has no positive divisors other than 1 and itself. This means it's only divisible by 1 and itself without leaving a remainder. The first few prime numbers are 2, 3, 5, 7, 11, 13, and so on. The number 1 is not considered a prime number.
What is Prime Factorization?
Prime factorization is the process of expressing a composite number (a number that is not prime) as a product of its prime factors. Every composite number can be uniquely expressed as a product of prime numbers. This unique representation is known as the fundamental theorem of arithmetic. This theorem is crucial in number theory and forms the basis for many mathematical concepts.
Finding the Prime Factors of 175: A Step-by-Step Approach
Now, let's tackle the main question: What are the prime factors of 175? We'll use a systematic approach to find the answer.
Step 1: Identify the Smallest Prime Factor
We start by identifying the smallest prime number that divides 175 without leaving a remainder. The smallest prime number is 2. However, 175 is an odd number, so it's not divisible by 2. The next prime number is 3. Adding the digits of 175 (1 + 7 + 5 = 13), which is not divisible by 3, indicates that 175 is not divisible by 3. The next prime number is 5. Since 175 ends in 5, it's clearly divisible by 5.
Step 2: Perform the Division
Dividing 175 by 5, we get:
175 ÷ 5 = 35
Step 3: Continue the Process
Now we need to find the prime factors of 35. 35 is also divisible by 5:
35 ÷ 5 = 7
Step 4: Identify the Remaining Factor
The result is 7, which is a prime number. We've reached a prime number, indicating that we've completed the factorization.
Step 5: Express the Prime Factorization
Therefore, the prime factorization of 175 is 5 x 5 x 7, or 5² x 7.
Visualizing Prime Factorization: The Factor Tree
A factor tree is a visual aid that helps in understanding the prime factorization process. Let's create a factor tree for 175:
175
/ \
5 35
/ \
5 7
The tree starts with the original number (175). Each branch represents a division by a prime factor, leading to smaller numbers until all the factors are prime. The prime factors are found at the end of each branch.
Applications of Prime Factorization
Prime factorization, despite its seemingly simple nature, has significant applications in various fields:
-
Cryptography: The security of many encryption algorithms, including RSA, relies heavily on the difficulty of factoring large numbers into their prime factors. The computational complexity of this process makes it computationally infeasible to crack these encryption systems.
-
Computer Science: Prime factorization is used in various algorithms and data structures. For example, it plays a role in efficient hash table implementations and certain types of sorting algorithms.
-
Number Theory: Prime factorization is a fundamental concept in number theory, forming the basis for many advanced theorems and concepts. It helps in understanding the properties of numbers and their relationships.
-
Mathematics Education: Understanding prime factorization helps build a strong foundation in mathematics, developing critical thinking and problem-solving skills.
Advanced Techniques for Prime Factorization
For larger numbers, the trial division method (as used above) can be time-consuming. More advanced algorithms exist to efficiently factor larger numbers:
-
Sieve of Eratosthenes: This algorithm is used to efficiently find all prime numbers up to a specified limit. While not directly used for factorization, it can assist in identifying potential prime factors.
-
Pollard's rho algorithm: This probabilistic algorithm is relatively efficient for finding small prime factors.
-
General Number Field Sieve (GNFS): This is the most efficient known algorithm for factoring very large numbers. It's used in tackling numbers with hundreds of digits, crucial in the context of cryptography.
Beyond 175: Practicing Prime Factorization
To solidify your understanding, try factoring other numbers using the techniques described above. Start with smaller numbers and gradually increase the complexity. Practice will build your proficiency and improve your ability to identify prime factors quickly. Remember to always check for divisibility by the smallest prime numbers first (2, 3, 5, 7, 11, etc.).
Conclusion: The Power of Prime Numbers
The seemingly simple question, "What are the prime factors of 175?" opens the door to a fascinating world of number theory and its applications. Understanding prime factorization is not just about finding the components of a number; it's about appreciating the fundamental building blocks of arithmetic and their significant role in various fields. Mastering this concept provides a solid foundation for more advanced mathematical concepts and problem-solving skills. The prime factors of 175 – 5² x 7 – represent a small piece of a much larger and intricate mathematical puzzle.
Latest Posts
Latest Posts
-
How To Calculate Loss Of Profit
May 08, 2025
-
What Is The Approximate Value Of X
May 08, 2025
-
How To Find An Original Price
May 08, 2025
-
Example Of Inverse Operations In Math
May 08, 2025
-
Simplify The Square Root Of 30
May 08, 2025
Related Post
Thank you for visiting our website which covers about What Are The Prime Factors Of 175 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.