What Does 1/3 + 1/3 Equal
News Co
May 08, 2025 · 5 min read
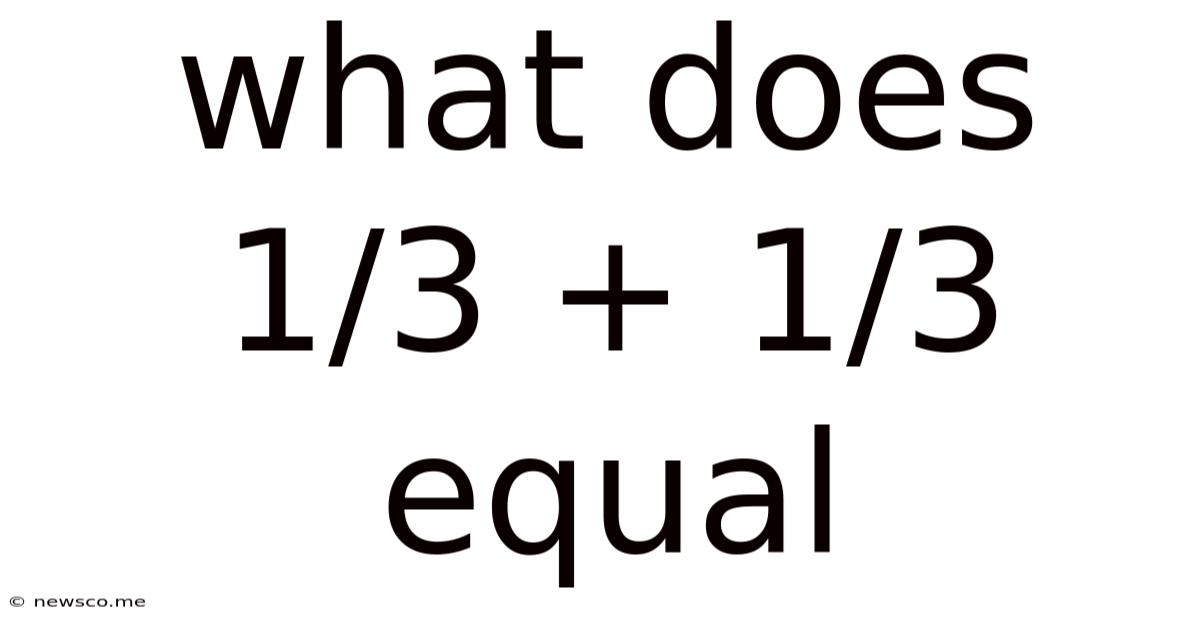
Table of Contents
What Does 1/3 + 1/3 Equal? A Deep Dive into Fraction Addition
The seemingly simple question, "What does 1/3 + 1/3 equal?" opens the door to a fascinating exploration of fundamental mathematical concepts. While the answer itself is straightforward for those familiar with fractions, the underlying principles and their applications extend far beyond this basic addition problem. This article will delve into not only the solution but also the broader significance of understanding fraction addition and its relevance in various fields.
Understanding Fractions: The Building Blocks
Before tackling the addition problem, let's solidify our understanding of fractions. A fraction represents a part of a whole. It's expressed as a ratio of two numbers: the numerator (the top number) and the denominator (the bottom number). The denominator indicates the number of equal parts the whole is divided into, while the numerator indicates how many of those parts are being considered.
In our example, 1/3 represents one part out of three equal parts. Visualizing this can be helpful. Imagine a pizza cut into three equal slices. 1/3 represents one of those slices.
Key Fraction Terminology: A Quick Review
- Numerator: The top number in a fraction, indicating the number of parts.
- Denominator: The bottom number in a fraction, indicating the total number of equal parts.
- Proper Fraction: A fraction where the numerator is smaller than the denominator (e.g., 1/3, 2/5).
- Improper Fraction: A fraction where the numerator is equal to or greater than the denominator (e.g., 5/3, 7/4).
- Mixed Number: A number consisting of a whole number and a proper fraction (e.g., 1 2/3).
Adding Fractions: A Step-by-Step Guide
Adding fractions with the same denominator is remarkably simple. The process involves adding the numerators while keeping the denominator unchanged. Let's apply this to our problem: 1/3 + 1/3.
-
Check the denominators: Both fractions have the same denominator, 3. This is crucial for direct addition.
-
Add the numerators: Add the numerators: 1 + 1 = 2.
-
Keep the denominator the same: The denominator remains 3.
-
The result: Therefore, 1/3 + 1/3 = 2/3.
This means that if you have one slice of pizza out of three and you add another slice, you now have two slices out of three.
Visualizing Fraction Addition
Visual aids can greatly enhance understanding, especially when dealing with abstract concepts like fractions. Let's visualize 1/3 + 1/3 using a simple diagram:
Imagine two circles, each divided into three equal parts. Shade one part in the first circle (representing 1/3) and one part in the second circle (representing another 1/3). Combining the shaded parts shows that you have two parts out of a total of three, illustrating 2/3.
Adding Fractions with Different Denominators
While adding fractions with the same denominator is straightforward, adding fractions with different denominators requires an extra step: finding a common denominator. This is the smallest number that both denominators can divide into evenly.
Let's consider the example of 1/2 + 1/4.
-
Find the least common denominator (LCD): The LCD of 2 and 4 is 4.
-
Convert fractions to equivalent fractions with the LCD: 1/2 is equivalent to 2/4 (multiply both numerator and denominator by 2).
-
Add the numerators: 2/4 + 1/4 = 3/4.
The key is that converting fractions to equivalent fractions with the same denominator doesn't change their value. It simply represents the same portion of a whole in a different way.
Applications of Fraction Addition in Real Life
Understanding fraction addition is not merely an academic exercise; it's a practical skill applicable in numerous everyday situations:
-
Cooking and Baking: Recipes often require fractional amounts of ingredients. Adding fractions helps determine the total quantity of ingredients needed.
-
Construction and Engineering: Precise measurements are critical in construction and engineering projects. Adding fractions helps ensure accurate calculations for dimensions and materials.
-
Finance and Budgeting: Managing finances involves working with percentages and fractions. Adding fractions helps track expenses and savings accurately.
-
Data Analysis: In data analysis and statistics, understanding fractions is essential for interpreting proportions and probabilities.
Beyond Basic Addition: Extending the Concepts
The foundation of fraction addition extends to more complex mathematical concepts:
-
Subtraction of Fractions: The principles of finding common denominators also apply to subtracting fractions.
-
Multiplication and Division of Fractions: These operations involve different rules but build upon the fundamental understanding of fractions and their representation.
-
Algebra and Calculus: Fractions are fundamental building blocks in advanced mathematical fields like algebra and calculus, playing a crucial role in solving equations and performing calculations.
Mastering Fractions: Tips and Resources
Mastering fractions takes practice and consistent effort. Here are some helpful tips:
-
Visualizations: Use diagrams, charts, and real-world objects to visualize fractions and their operations.
-
Practice Regularly: Solving numerous problems helps solidify understanding and develop fluency.
-
Utilize Online Resources: Many websites and educational platforms offer interactive exercises and tutorials on fractions.
-
Seek Help When Needed: Don't hesitate to ask teachers, tutors, or peers for assistance when facing difficulties.
Conclusion: The Significance of Understanding Fractions
The seemingly simple question, "What does 1/3 + 1/3 equal?" serves as a gateway to a deeper appreciation of the importance of fractions in mathematics and their widespread applications in various aspects of life. Understanding fractions is not just about solving equations; it's about developing a fundamental understanding of proportions, ratios, and the ability to represent parts of a whole. By mastering fraction addition and related concepts, we equip ourselves with essential skills applicable in diverse fields, paving the way for further mathematical exploration and problem-solving capabilities. The seemingly simple 2/3 is a testament to the power and elegance of mathematical principles.
Latest Posts
Related Post
Thank you for visiting our website which covers about What Does 1/3 + 1/3 Equal . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.