What Is 1 3 X 3
News Co
Mar 20, 2025 · 5 min read
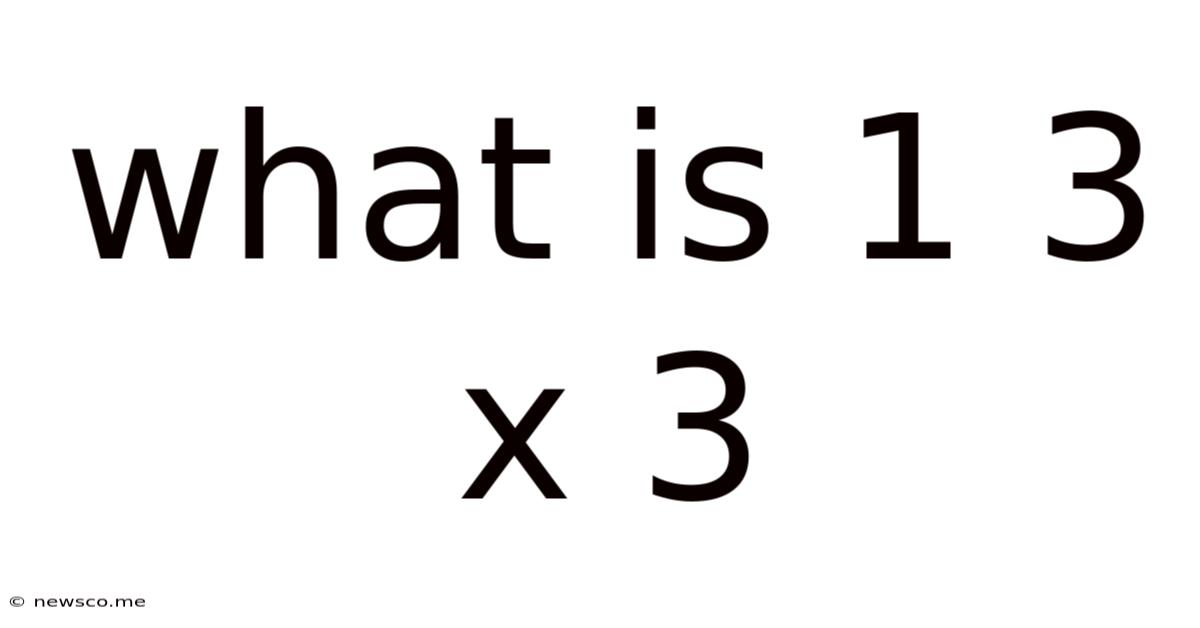
Table of Contents
What is 1/3 x 3? Understanding Fractions and Multiplication
This seemingly simple question, "What is 1/3 x 3?", actually opens the door to understanding fundamental concepts in mathematics, specifically fractions and multiplication. While the answer might seem immediately obvious to some, delving deeper reveals valuable insights into how fractions behave within mathematical operations and lays a solid foundation for more complex calculations. This article will not only answer the question but also explore the underlying principles, providing a comprehensive understanding for both beginners and those looking for a refresher.
Understanding Fractions: The Building Blocks
Before tackling the multiplication problem, let's solidify our grasp of fractions. A fraction represents a part of a whole. It's composed of two key components:
- Numerator: The top number, indicating how many parts we have.
- Denominator: The bottom number, showing the total number of equal parts the whole is divided into.
In our case, 1/3 signifies one part out of a total of three equal parts. Imagine a pizza cut into three slices; 1/3 represents one of those slices.
Visualizing 1/3 x 3
Visual representation is a powerful tool for understanding mathematical concepts. Let's visualize 1/3 x 3:
Imagine we have three pizzas, each cut into three equal slices. 1/3 x 3 means we're taking one slice from each of the three pizzas.
- Pizza 1: We take 1/3 (one slice).
- Pizza 2: We take 1/3 (one slice).
- Pizza 3: We take 1/3 (one slice).
In total, we have collected three slices. Since each pizza had three slices, we've essentially accumulated one whole pizza. Therefore, 1/3 x 3 = 1.
The Mathematical Process: Step-by-Step
While visualization helps intuition, understanding the mathematical process is crucial for tackling more complex problems. Here's a step-by-step breakdown of calculating 1/3 x 3:
-
Multiply the numerators: In this case, the numerator of the fraction is 1, and we're multiplying by 3. So, 1 x 3 = 3.
-
Multiply the denominators: The denominator of the fraction is 3, and we are multiplying it by 1 (since 3 can be written as 3/1). Thus, 3 x 1 = 3.
-
Form the new fraction: This gives us a new fraction: 3/3.
-
Simplify the fraction: 3/3 means 3 parts out of 3 equal parts, which simplifies to 1 (a whole).
Therefore, the mathematical calculation confirms our visual understanding: 1/3 x 3 = 1.
Expanding the Concept: Fractions and Multiplication in General
The principles applied to 1/3 x 3 can be generalized to any fraction multiplication problem:
a/b x c = (a x c) / b
where 'a', 'b', and 'c' are integers (whole numbers), and 'b' is not equal to zero (division by zero is undefined).
This formula highlights the core process: multiply the numerators and then the denominators. Simplify the resulting fraction if possible.
Let's look at another example: 2/5 x 4
- Multiply numerators: 2 x 4 = 8
- Multiply denominators: 5 x 1 = 5
- Resulting fraction: 8/5
- Simplification: This fraction is an improper fraction (numerator is larger than the denominator). We can simplify it to a mixed number: 1 3/5.
Real-World Applications: Where Fractions and Multiplication Matter
Understanding fractions and multiplication is crucial in countless real-world scenarios:
-
Cooking and Baking: Recipes often involve fractional measurements. Calculating the correct amounts when scaling a recipe up or down relies on understanding fraction multiplication. For instance, if a recipe calls for 1/2 cup of sugar and you want to double the recipe, you need to calculate 1/2 x 2.
-
Construction and Engineering: Accurate measurements are essential. Fractions are used extensively in blueprints and specifications, requiring precise calculations involving multiplication and division.
-
Finance and Budgeting: Managing personal finances often involves working with fractions of amounts (e.g., calculating interest rates, discounts, or portions of expenses).
-
Data Analysis: In various fields, data analysis involves working with proportions and percentages, which are fundamentally related to fractions.
Common Mistakes to Avoid
While seemingly straightforward, fraction multiplication can sometimes lead to errors. Here are some common mistakes to watch out for:
-
Incorrect multiplication of numerators and denominators: Always remember to multiply the numerators together and the denominators together separately.
-
Forgetting to simplify: Ensure you simplify your answer to its lowest terms. An improper fraction should be converted to a mixed number.
-
Improper cancellation: While you can sometimes cancel common factors before multiplying (e.g., simplifying 2/4 x 1/2 to 1/4 by canceling the 2s), this requires careful attention to ensure accuracy.
Beyond the Basics: Further Exploration
Understanding 1/3 x 3 is a stepping stone to more complex mathematical concepts. From here, you can progress to:
-
Multiplication of mixed numbers: This involves converting mixed numbers into improper fractions before applying the standard multiplication rules.
-
Division of fractions: This involves inverting the second fraction (reciprocal) and then multiplying.
-
Working with decimals and fractions: Converting between decimals and fractions is a valuable skill for diverse calculations.
-
Algebraic manipulation of fractions: Fractions play a critical role in algebraic equations and manipulations.
Conclusion: Mastering the Fundamentals
The question "What is 1/3 x 3?" might seem trivial at first glance, but it provides a solid foundation for understanding fundamental mathematical concepts. By visualizing the problem, working through the mathematical steps, and recognizing common pitfalls, you gain a deeper understanding of fraction multiplication and its extensive applications in various fields. Mastering these fundamentals lays a strong base for tackling more advanced mathematical challenges and successfully applying these skills in various aspects of life. The journey from understanding a simple fraction multiplication problem to mastering more complex concepts is a rewarding one, empowering you with the analytical skills needed to navigate a world filled with numerical challenges.
Latest Posts
Latest Posts
-
Find The Point On The Y Axis Which Is Equidistant From
May 09, 2025
-
Is 3 4 Bigger Than 7 8
May 09, 2025
-
Which Of These Is Not A Prime Number
May 09, 2025
-
What Is 30 Percent Off Of 80 Dollars
May 09, 2025
-
Are Alternate Exterior Angles Always Congruent
May 09, 2025
Related Post
Thank you for visiting our website which covers about What Is 1 3 X 3 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.