What Is 1 To The Power Of 5
News Co
Apr 07, 2025 · 5 min read
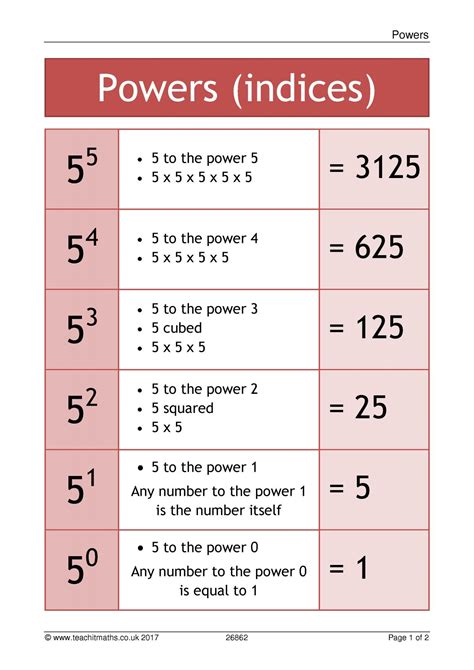
Table of Contents
What is 1 to the Power of 5? A Deep Dive into Exponents and Their Applications
The seemingly simple question, "What is 1 to the power of 5?", opens a door to a fascinating exploration of exponents, their properties, and their wide-ranging applications in mathematics, science, and computer science. While the answer itself is straightforward – 1 – the journey to understanding why it's 1 reveals fundamental concepts crucial for more advanced mathematical studies. This article will not only answer the question but delve deep into the underlying principles, exploring practical examples and highlighting the significance of exponential notation in various fields.
Understanding Exponents: The Power of Repeated Multiplication
Before tackling 1<sup>5</sup>, let's establish a solid understanding of exponents. An exponent, also known as a power or index, indicates how many times a base number is multiplied by itself. The general form is:
b<sup>n</sup>
where:
- b is the base (the number being multiplied)
- n is the exponent (the number of times the base is multiplied by itself)
For instance, 2<sup>3</sup> (2 to the power of 3) means 2 × 2 × 2 = 8. The exponent 3 indicates that the base 2 is multiplied by itself three times.
The Special Case of Exponent 0
A noteworthy point is the case when the exponent is 0. Any non-zero number raised to the power of 0 is always 1. This is a fundamental rule in mathematics:
b<sup>0</sup> = 1 (where b ≠ 0)
This rule might seem counterintuitive at first, but it's consistent with the properties of exponents and necessary for mathematical consistency. We can derive this rule by considering the pattern of decreasing exponents:
- 2<sup>3</sup> = 8
- 2<sup>2</sup> = 4 (8 / 2)
- 2<sup>1</sup> = 2 (4 / 2)
- 2<sup>0</sup> = 1 (2 / 2)
This pattern logically leads to 2<sup>0</sup> = 1.
Negative Exponents: The Reciprocal
Another essential concept is negative exponents. A negative exponent indicates the reciprocal of the base raised to the positive exponent:
b<sup>-n</sup> = 1/b<sup>n</sup>
For example, 2<sup>-2</sup> = 1/2<sup>2</sup> = 1/4 = 0.25
Calculating 1 to the Power of 5
Now, let's return to our original question: What is 1<sup>5</sup>? Applying the definition of exponents, we have:
1<sup>5</sup> = 1 × 1 × 1 × 1 × 1 = 1
Regardless of the exponent's value (positive, negative, or zero), any power of 1 will always equal 1. This stems from the multiplicative identity property of 1: any number multiplied by 1 remains unchanged.
The Significance of Exponents Beyond Simple Calculations
The concept of exponents extends far beyond simple calculations like 1<sup>5</sup>. Its importance is evident across various fields:
1. Scientific Notation: Representing Extremely Large and Small Numbers
Scientists frequently deal with extremely large or small numbers. Exponents provide a concise and efficient way to represent these numbers using scientific notation. For example, the speed of light is approximately 3 × 10<sup>8</sup> meters per second, meaning 3 multiplied by 10 eight times.
2. Compound Interest: Growth and Decay Calculations
In finance, compound interest calculations rely heavily on exponents. The formula for compound interest involves an exponential term that demonstrates the growth of an investment over time. Similarly, exponential decay models are used to describe phenomena such as radioactive decay.
3. Computer Science: Algorithms and Data Structures
Exponents play a vital role in analyzing the efficiency of algorithms. The time complexity of many algorithms is expressed using exponential notation (e.g., O(2<sup>n</sup>), indicating exponential growth in computation time with increasing input size). Data structures like binary trees also utilize exponential relationships.
4. Biology: Population Growth and Modeling
Exponential growth is frequently observed in biological systems, such as bacterial populations. Exponential models are used to predict population size over time, considering factors like birth rates and death rates.
5. Physics: Describing Physical Phenomena
Exponential functions describe a variety of physical phenomena, from radioactive decay and the cooling of objects to the intensity of light traveling through a medium. These models provide crucial insights into the underlying physical processes.
6. Mathematics: Polynomials and Series
Exponents are fundamental in the study of polynomials (expressions involving variables raised to various powers) and infinite series (sums of infinite sequences). Many important mathematical functions, such as the exponential function (e<sup>x</sup>), are defined using infinite series involving exponents.
Beyond the Basics: Exploring More Complex Exponential Concepts
While 1<sup>5</sup> provides a simple starting point, exploring more complex aspects of exponents deepens our understanding of their significance.
Fractional Exponents: Radicals and Roots
Fractional exponents represent roots. For example, 8<sup>1/3</sup> means the cube root of 8, which is 2. This extends the concept of exponents to include non-integer values.
Irrational Exponents: Transcendentals
Exponents can also be irrational numbers (numbers that cannot be expressed as a fraction of two integers), such as π (pi) or e (Euler's number). These lead to transcendental numbers, which are numbers that are not roots of any non-zero polynomial with rational coefficients.
Complex Exponents: Extending into the Complex Plane
The concept of exponents can be extended even further into the realm of complex numbers (numbers involving the imaginary unit 'i', where i<sup>2</sup> = -1). Euler's formula, e<sup>ix</sup> = cos(x) + i sin(x), connects exponential functions with trigonometric functions in a profound and elegant way. This has far-reaching consequences in various areas of mathematics and physics.
Conclusion: The Power of Understanding Exponents
The seemingly simple problem of calculating 1<sup>5</sup> serves as a gateway to understanding the broader significance of exponents in mathematics and its applications. While the answer is simply 1, the journey to arrive at this answer highlights fundamental mathematical principles and opens doors to a wealth of more complex and fascinating concepts. The power of exponents extends far beyond simple calculations; it forms the backbone of numerous models and algorithms used across various scientific and computational disciplines. A solid grasp of exponents is essential for anyone pursuing studies in mathematics, science, engineering, or computer science. Understanding its nuances allows one to tackle complex problems and contribute to advancements across a broad spectrum of fields.
Latest Posts
Related Post
Thank you for visiting our website which covers about What Is 1 To The Power Of 5 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.