What Is 2/3 Divided By 2 As A Fraction
News Co
May 08, 2025 · 5 min read
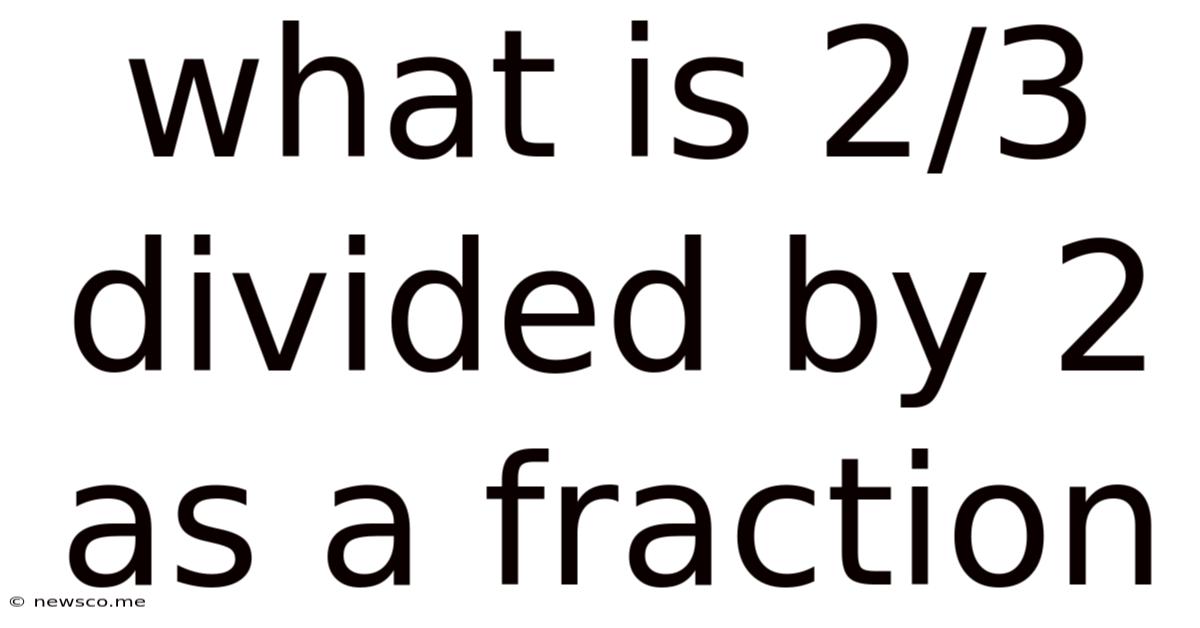
Table of Contents
What is 2/3 Divided by 2 as a Fraction? A Comprehensive Guide
Understanding fractions and how to manipulate them is a fundamental skill in mathematics. This seemingly simple question – "What is 2/3 divided by 2 as a fraction?" – offers a great opportunity to explore core concepts and build a strong foundation in fractional arithmetic. This guide will not only answer the question but also delve into the underlying principles, providing you with a comprehensive understanding of division with fractions.
Understanding Fraction Division
Before we tackle the specific problem, let's review the basics of fraction division. Dividing by a fraction is essentially the same as multiplying by its reciprocal. The reciprocal of a fraction is simply the fraction flipped upside down. For example, the reciprocal of 2/3 is 3/2.
Key Concept: Dividing by a number is the same as multiplying by its multiplicative inverse (reciprocal).
This concept is crucial because it transforms a division problem into a multiplication problem, which is often easier to solve.
Solving 2/3 Divided by 2
Now, let's apply this concept to our problem: 2/3 divided by 2. First, we need to express the whole number 2 as a fraction. Any whole number can be written as a fraction by placing it over 1. Therefore, 2 can be written as 2/1.
Our problem now becomes: (2/3) ÷ (2/1)
Applying the rule of fraction division, we change the division sign to a multiplication sign and flip the second fraction (find its reciprocal):
(2/3) x (1/2)
Now we perform the multiplication:
Numerator: 2 x 1 = 2 Denominator: 3 x 2 = 6
This gives us the fraction 2/6.
Simplifying Fractions
The fraction 2/6 is not in its simplest form. To simplify a fraction, we find the greatest common divisor (GCD) of the numerator and the denominator and divide both by it. The GCD of 2 and 6 is 2.
Dividing both the numerator and denominator by 2, we get:
2 ÷ 2 = 1 6 ÷ 2 = 3
Therefore, the simplified answer is 1/3.
Alternative Methods for Solving Fraction Division Problems
While the reciprocal method is generally preferred, there are other ways to approach this type of problem. Understanding these alternative methods can provide a deeper understanding of the underlying mathematical principles.
Method 2: Using the Concept of Sharing
Consider the problem as sharing 2/3 of something among 2 people. If you have 2/3 of a pizza and you want to divide it equally between 2 people, each person gets half of 2/3. This can be represented as:
(2/3) x (1/2)
This leads to the same result as before: 2/6, which simplifies to 1/3. This method offers a visual and intuitive approach to solving the problem.
Method 3: Converting to Decimal and Back
You could also convert the fraction 2/3 to its decimal equivalent (approximately 0.6667), divide by 2 (resulting in approximately 0.3333), and then convert the decimal back into a fraction. However, this method can introduce rounding errors and is generally less efficient and precise than the reciprocal method. It's also more prone to mistakes, particularly when dealing with fractions that don't have exact decimal equivalents.
Expanding on Fraction Division: More Complex Scenarios
Now that we've tackled the basic problem, let's explore more complex scenarios involving fraction division. Understanding these scenarios will further solidify your grasp of the concept.
Scenario 1: Dividing a Fraction by a Mixed Number
A mixed number is a combination of a whole number and a fraction (e.g., 1 1/2). To divide a fraction by a mixed number, first convert the mixed number into an improper fraction. An improper fraction is a fraction where the numerator is larger than or equal to the denominator.
Example: (2/5) ÷ (1 1/2)
-
Convert 1 1/2 to an improper fraction: (1 x 2) + 1 = 3/2
-
Apply the reciprocal rule: (2/5) x (2/3)
-
Multiply: (2 x 2) / (5 x 3) = 4/15
Scenario 2: Dividing a Mixed Number by a Fraction
Similarly, if you have a mixed number divided by a fraction, first convert the mixed number into an improper fraction before applying the reciprocal rule.
Example: (1 2/3) ÷ (1/4)
-
Convert 1 2/3 to an improper fraction: (1 x 3) + 2 = 5/3
-
Apply the reciprocal rule: (5/3) x (4/1)
-
Multiply: (5 x 4) / (3 x 1) = 20/3
Scenario 3: Dividing a Mixed Number by a Mixed Number
The most complex scenario involves dividing a mixed number by another mixed number. The process remains the same: convert both mixed numbers to improper fractions, apply the reciprocal rule, and simplify the result.
Example: (2 1/4) ÷ (1 1/2)
- Convert 2 1/4 to an improper fraction: (2 x 4) + 1 = 9/4
- Convert 1 1/2 to an improper fraction: (1 x 2) + 1 = 3/2
- Apply the reciprocal rule: (9/4) x (2/3)
- Multiply: (9 x 2) / (4 x 3) = 18/12
- Simplify: 18/12 = 3/2 or 1 1/2
Practical Applications of Fraction Division
Fraction division is not just an abstract mathematical concept; it has numerous practical applications in everyday life and various professional fields. Here are a few examples:
- Cooking and Baking: Scaling recipes up or down often requires dividing fractions. If a recipe calls for 1/2 cup of flour and you want to make half the recipe, you would need to divide 1/2 by 2.
- Construction and Engineering: Precise measurements are crucial, and often these involve fractions. Dividing fractions is essential for accurate calculations.
- Sewing and Tailoring: Cutting fabric requires precise measurements, and fractions are frequently involved in calculations.
- Finance: Calculating interest rates, proportions of investments, and shares often involves fraction division.
Conclusion: Mastering Fraction Division
Understanding fraction division is a critical skill with widespread applications. By mastering the reciprocal method and understanding the underlying principles, you can confidently tackle various problems, from simple calculations to more complex scenarios involving mixed numbers. Practice is key to solidifying your understanding and building fluency. Remember to always simplify your final answer to its lowest terms for a complete and accurate solution. Through consistent practice and application, you will develop a strong foundation in fraction arithmetic, opening doors to a deeper understanding of mathematics and its applications in the real world.
Latest Posts
Latest Posts
-
What Is 1 3 8 In Fraction
May 08, 2025
-
Lcm Of 2 3 And 11
May 08, 2025
-
Are Diagonals Perpendicular In A Parallelogram
May 08, 2025
-
Is Every Integer A Whole Number
May 08, 2025
-
The Greatest Prime Factor Of 36x
May 08, 2025
Related Post
Thank you for visiting our website which covers about What Is 2/3 Divided By 2 As A Fraction . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.