What Is 2 To The Negative 1 Power
News Co
May 08, 2025 · 5 min read
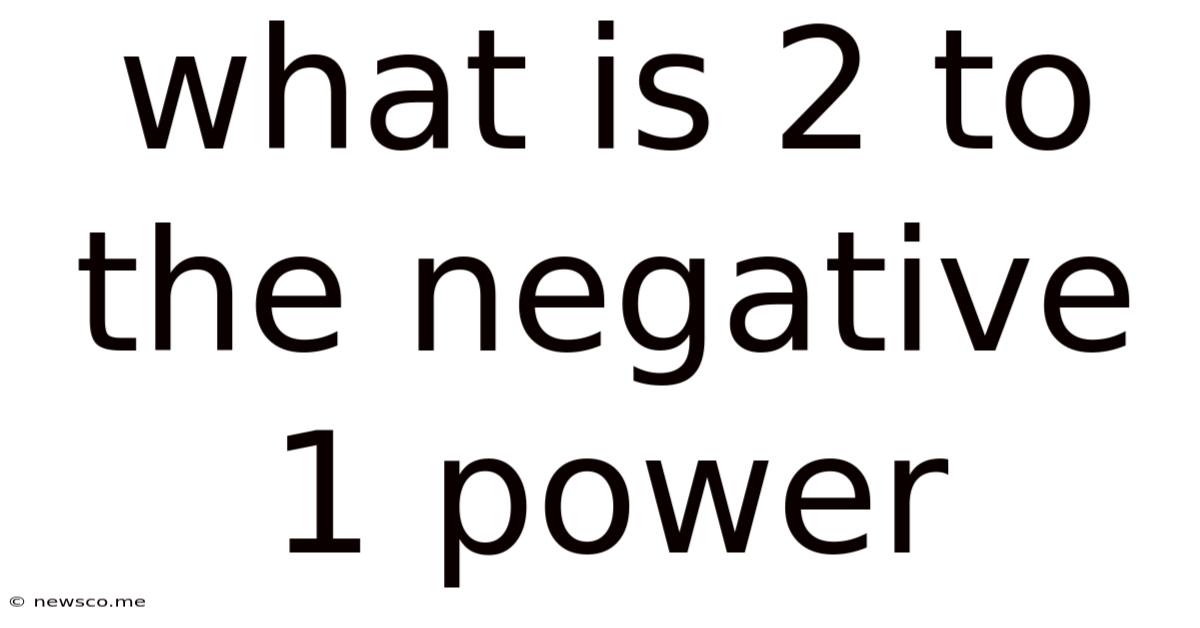
Table of Contents
What is 2 to the Negative 1 Power? A Comprehensive Guide
Understanding exponents, especially negative exponents, can be a stumbling block for many. This comprehensive guide will delve deep into the meaning of 2 to the negative 1 power (2⁻¹), explaining its calculation, its relationship to other mathematical concepts, and its practical applications. We'll explore this seemingly simple expression to reveal its fundamental importance in mathematics and beyond.
Understanding Exponents: A Quick Refresher
Before tackling negative exponents, let's refresh our understanding of positive exponents. An exponent (also called a power or index) indicates how many times a base number is multiplied by itself. For example:
- 2³ = 2 × 2 × 2 = 8 (2 to the power of 3 is 2 multiplied by itself three times)
- 5² = 5 × 5 = 25 (5 to the power of 2 is 5 multiplied by itself twice)
- 10¹ = 10 (10 to the power of 1 is simply 10)
These are all examples of positive integer exponents. But what happens when the exponent is negative? That's where things get interesting.
Deciphering Negative Exponents: The Reciprocal Rule
The key to understanding negative exponents lies in the concept of the reciprocal. The reciprocal of a number is simply 1 divided by that number. For example:
- The reciprocal of 2 is 1/2
- The reciprocal of 5 is 1/5
- The reciprocal of 10 is 1/10
The rule for negative exponents states: a⁻ⁿ = 1/aⁿ
This means that a number raised to a negative power is equal to 1 divided by that number raised to the positive power. Let's apply this to our example:
2⁻¹ = 1/2¹ = 1/2 = 0.5
Therefore, 2 to the negative 1 power is equal to 1/2, or 0.5.
Expanding the Understanding: Different Bases and Exponents
The rule for negative exponents applies to any base, not just 2. Let's explore a few more examples:
- 3⁻² = 1/3² = 1/9
- 10⁻³ = 1/10³ = 1/1000 = 0.001
- (1/4)⁻¹ = 1/(1/4)¹ = 4 (Note that the reciprocal of a fraction flips the numerator and denominator)
Notice that a negative exponent doesn't inherently mean a negative result. The result depends on the base number. However, the result will always be a fraction or a decimal less than 1 (unless the base itself is a fraction less than 1).
Connecting Negative Exponents to Scientific Notation
Negative exponents play a crucial role in scientific notation, a way of representing very large or very small numbers concisely. Scientific notation expresses numbers in the form a × 10ⁿ, where 'a' is a number between 1 and 10, and 'n' is an integer exponent.
For example, the speed of light is approximately 3 × 10⁸ meters per second. This means 3 multiplied by 10 to the power of 8. Conversely, extremely small numbers, like the size of an atom, are often represented using negative exponents. For instance, 0.0000000001 meters could be written as 1 × 10⁻¹⁰ meters. This highlights the utility of negative exponents in representing a wide range of magnitudes.
Negative Exponents in Algebra and Calculus
Negative exponents are not just confined to simple numerical calculations. They are fundamental in more advanced mathematical areas such as algebra and calculus.
In algebra, negative exponents appear in various equations and manipulations, often requiring simplification using the rules of exponents. For example, consider simplifying the expression: x³ * x⁻². Using the rule of adding exponents when multiplying with the same base, we get x³⁻² = x¹.
In calculus, negative exponents are essential in differentiation and integration, appearing in power rules and other key formulas. For example, the derivative of x⁻² is -2x⁻³. Mastering negative exponents is therefore critical for success in these advanced mathematical fields.
Real-World Applications of Negative Exponents
Beyond the realm of pure mathematics, negative exponents find numerous real-world applications across various disciplines:
- Physics: Describing decay rates in radioactive materials or the inverse square law for gravity and light intensity.
- Chemistry: Representing concentrations of dilute solutions or reaction rates.
- Engineering: Modeling signal attenuation in electrical circuits or calculating the inverse relationship between pressure and volume in gases (Boyle's Law).
- Finance: Compound interest calculations often involve negative exponents when dealing with present value discounting.
- Computer Science: Representing memory sizes, particularly in binary systems.
Common Mistakes to Avoid
While the concept of negative exponents seems straightforward, certain pitfalls can trap beginners:
- Confusing negative exponents with negative numbers: A negative exponent does not make the entire expression negative. It indicates a reciprocal operation.
- Incorrectly applying exponent rules: Ensure you understand the rules for adding, subtracting, multiplying, and dividing exponents. Pay close attention to the order of operations (PEMDAS/BODMAS).
- Forgetting to apply the reciprocal correctly: Remember that a⁻ⁿ = 1/aⁿ, not a/1ⁿ.
Mastering Negative Exponents: Tips and Practice
To develop a strong understanding of negative exponents, consider the following:
- Practice regularly: Work through various problems with different bases and exponents.
- Visualize the concept: Imagine the reciprocal operation to reinforce your understanding.
- Use online resources: Many websites and educational platforms offer interactive exercises and explanations.
- Seek help when needed: Don't hesitate to ask for assistance from teachers, tutors, or online communities.
Conclusion: The Significance of 2⁻¹ and Beyond
While seemingly simple, 2⁻¹ (or 1/2) represents a foundational concept within mathematics, encompassing the powerful and versatile idea of negative exponents. Understanding this seemingly small concept unlocks a gateway to a deeper appreciation of exponential functions, scientific notation, and advanced mathematical and scientific concepts. Its diverse applications demonstrate its significance beyond the classroom, highlighting the pervasive influence of this fundamental mathematical principle in various facets of our world. Mastering negative exponents significantly enhances your quantitative reasoning skills and expands your capabilities to tackle complex mathematical problems in academic and professional settings alike.
Latest Posts
Latest Posts
-
How To Find A1 In Arithmetic Sequence
May 09, 2025
-
Subtracting A Positive Integer From A Negative Integer
May 09, 2025
-
The Diagonals Of A Parallelogram Are Equal
May 09, 2025
-
Angle Pairs Worksheet Geometry Answer Key
May 09, 2025
-
What Is The Perimeter Of A Regular Pentagon
May 09, 2025
Related Post
Thank you for visiting our website which covers about What Is 2 To The Negative 1 Power . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.