What Is 20 Percent Of 90
News Co
Mar 15, 2025 · 5 min read
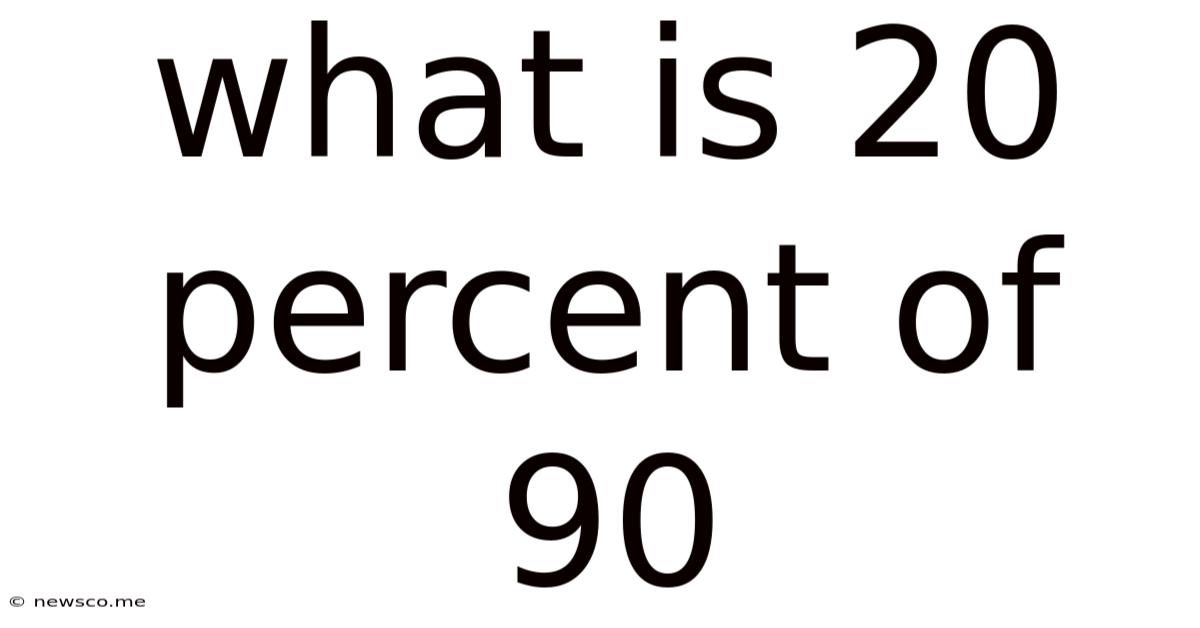
Table of Contents
What is 20 Percent of 90? A Deep Dive into Percentages and Their Applications
Finding 20 percent of 90 might seem like a simple arithmetic problem, solvable with a quick calculator search. However, understanding the underlying principles of percentages and their broad applications goes far beyond this single calculation. This article will not only answer the question "What is 20 percent of 90?" but also delve into the broader context of percentages, exploring different calculation methods, practical examples, and real-world applications across various fields.
Understanding Percentages: The Foundation
A percentage is a way of expressing a number as a fraction of 100. The word "percent" itself comes from the Latin "per centum," meaning "out of a hundred." Therefore, 20 percent (often written as 20%) means 20 out of 100, or 20/100, which simplifies to 1/5. This fundamental understanding is crucial for tackling any percentage problem.
Methods for Calculating 20 Percent of 90
There are several ways to calculate 20% of 90. Let's explore the most common and effective approaches:
Method 1: Using the Decimal Equivalent
This is arguably the most straightforward method. We convert the percentage to its decimal equivalent by dividing it by 100. 20% divided by 100 is 0.20 (or simply 0.2). Then, we multiply this decimal by the number we want to find the percentage of:
90 * 0.2 = 18
Therefore, 20% of 90 is 18.
Method 2: Using Fractions
As mentioned earlier, 20% is equivalent to the fraction 1/5. Therefore, we can calculate 20% of 90 by multiplying 90 by 1/5:
90 * (1/5) = 90/5 = 18
This method provides an alternative perspective and reinforces the fractional representation of percentages.
Method 3: Proportion Method
This method uses proportions to solve for the unknown value. We set up a proportion:
20/100 = x/90
Where 'x' represents 20% of 90. To solve for x, we cross-multiply:
20 * 90 = 100 * x
1800 = 100x
x = 1800/100 = 18
Again, we find that 20% of 90 is 18.
Real-World Applications of Percentage Calculations
The ability to calculate percentages is a vital skill applicable across numerous aspects of daily life and various professions. Here are some examples:
1. Finance and Budgeting:
- Calculating discounts: Stores frequently offer discounts on products. Understanding percentage calculations allows you to quickly determine the final price after a discount. For example, a 20% discount on a $90 item leads to a saving of $18.
- Interest rates: Interest rates on loans, savings accounts, and investments are expressed as percentages. Accurate calculation is crucial for understanding the cost of borrowing or the return on investment.
- Tax calculations: Sales taxes, income taxes, and other forms of taxation are often based on percentages of income or purchase price.
2. Sales and Marketing:
- Sales commission: Sales representatives frequently earn a commission based on a percentage of their sales. Calculating the commission accurately is essential for fair compensation.
- Market share analysis: Companies use percentages to analyze their market share relative to competitors. This helps in strategic planning and decision-making.
- Conversion rates: In online marketing, conversion rates (e.g., the percentage of website visitors who make a purchase) are vital metrics for evaluating campaign effectiveness.
3. Science and Statistics:
- Data analysis: Percentages are widely used to represent data in graphs, charts, and tables. They provide a clear and concise way to visualize proportions and trends.
- Probability and statistics: Percentages are fundamental in expressing probabilities and statistical significance.
- Scientific measurements: Many scientific measurements and experiments involve percentage calculations, such as error analysis and concentration calculations.
4. Everyday Life:
- Tip calculations: Calculating a tip in a restaurant often involves a percentage of the total bill.
- Recipe scaling: Adjusting recipe quantities requires understanding percentages to maintain the correct proportions of ingredients.
- Grading and assessment: Percentage scores are widely used in educational settings to assess performance.
Beyond the Basics: Advanced Percentage Calculations
While finding 20% of 90 is a relatively simple calculation, percentage problems can become more complex. Here are some advanced concepts:
1. Calculating Percentage Increase/Decrease:
These calculations involve finding the percentage change between two values. For example, if a product's price increases from $90 to $108, the percentage increase is calculated as follows:
(108 - 90) / 90 * 100% = 20%
2. Calculating the Original Value:
If you know the final value after a percentage increase or decrease, you can work backward to find the original value. For example, if a price is $108 after a 20% increase, the original price can be calculated using algebra.
Let x be the original price. Then:
x + 0.2x = 108
1.2x = 108
x = 108 / 1.2 = 90
3. Compound Interest:
Compound interest involves earning interest on both the principal amount and accumulated interest. These calculations require more advanced mathematical formulas.
The Importance of Accuracy in Percentage Calculations
Accuracy in percentage calculations is paramount in many contexts. In financial transactions, for instance, an inaccurate calculation could lead to significant financial losses. In scientific research, inaccurate percentages can invalidate experimental results. Therefore, double-checking your work and using reliable calculation methods is crucial.
Tools and Resources for Percentage Calculations
While manual calculations are valuable for understanding the underlying principles, various tools can simplify complex percentage calculations:
- Calculators: Both standard and scientific calculators have built-in functions for percentage calculations.
- Spreadsheet software: Programs like Microsoft Excel and Google Sheets provide numerous functions for handling percentage calculations, including advanced formulas for compound interest and other complex scenarios.
- Online percentage calculators: Many websites offer free online percentage calculators that can handle various percentage-related problems.
Conclusion: Mastering Percentages for Success
Understanding and applying percentage calculations is a fundamental skill with broad applications across various fields. While the simple problem of finding 20% of 90 serves as a starting point, exploring the underlying concepts, different calculation methods, and real-world applications provides a comprehensive understanding of this crucial mathematical concept. Mastering percentages empowers individuals and professionals to make informed decisions, analyze data effectively, and succeed in various aspects of life. From budgeting and finance to scientific research and marketing, the ability to accurately and efficiently handle percentages is an invaluable asset. Therefore, continue practicing these calculations and explore further applications to enhance your numeracy and problem-solving skills.
Latest Posts
Latest Posts
-
Find The Point On The Y Axis Which Is Equidistant From
May 09, 2025
-
Is 3 4 Bigger Than 7 8
May 09, 2025
-
Which Of These Is Not A Prime Number
May 09, 2025
-
What Is 30 Percent Off Of 80 Dollars
May 09, 2025
-
Are Alternate Exterior Angles Always Congruent
May 09, 2025
Related Post
Thank you for visiting our website which covers about What Is 20 Percent Of 90 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.