What Is 3 Times 3 Times 3
News Co
May 07, 2025 · 4 min read
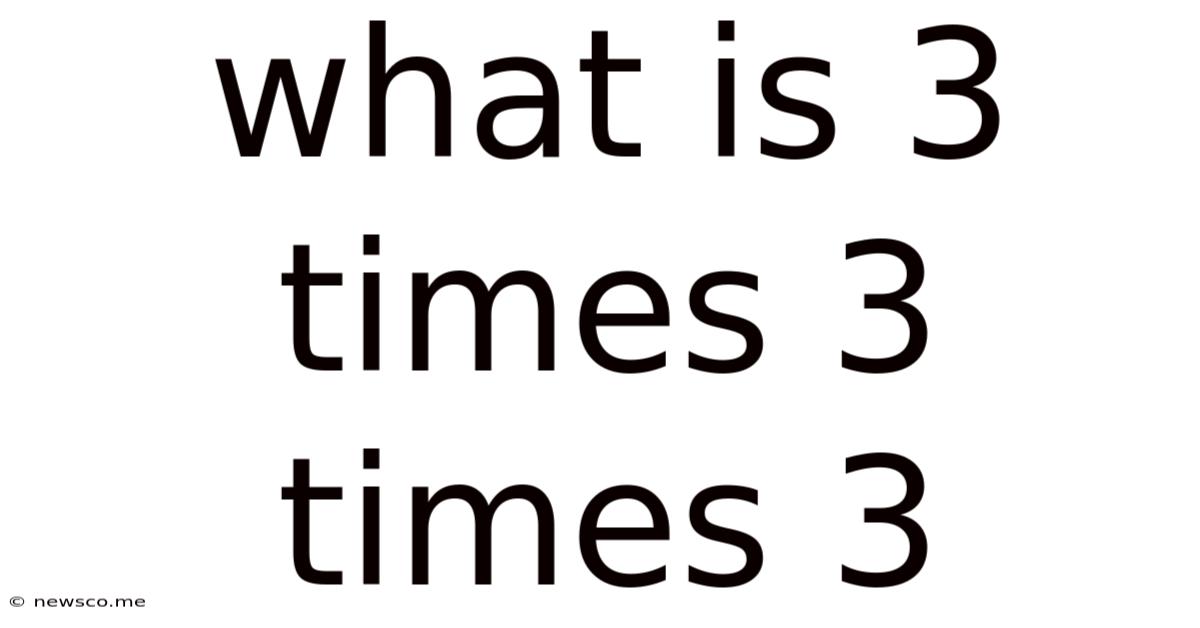
Table of Contents
What is 3 Times 3 Times 3? Exploring Multiplication and its Applications
The seemingly simple question, "What is 3 times 3 times 3?" opens a door to a fascinating exploration of multiplication, its properties, and its widespread applications in various fields. While the immediate answer is straightforward, understanding the underlying concepts allows us to appreciate the power and versatility of this fundamental mathematical operation. This comprehensive guide will delve into the solution, explore the principles of multiplication, and examine its significance in diverse contexts.
Understanding the Basics: Multiplication as Repeated Addition
At its core, multiplication is a shorthand method for repeated addition. When we say "3 times 3," we're essentially saying "3 added to itself 3 times." This translates to 3 + 3 + 3 = 9. Therefore, "3 times 3 times 3" signifies the repeated addition of 3, three separate times. This can be broken down as:
- Step 1: 3 x 3 = 9 (Three added to itself three times)
- Step 2: 9 x 3 = 27 (Nine added to itself three times)
Therefore, the answer to "What is 3 times 3 times 3?" is 27.
Beyond the Basics: Exponents and Exponential Growth
The expression "3 times 3 times 3" can be more concisely represented using exponents. An exponent indicates how many times a number (the base) is multiplied by itself. In this case, the base is 3, and since it's multiplied by itself three times, the exponent is 3. This is written as 3³.
Understanding exponents is crucial because they are fundamental to various mathematical concepts and applications, particularly in:
-
Exponential Growth: This describes a situation where a quantity increases at a rate proportional to its current value. Examples include population growth, compound interest, and the spread of viruses. In these scenarios, the exponent reflects the number of periods of growth.
-
Exponential Decay: This is the opposite of exponential growth, representing a decrease at a rate proportional to the current value. Examples include radioactive decay, the depreciation of assets, and the cooling of an object.
-
Geometric Sequences: These are sequences where each term is found by multiplying the previous term by a constant value. The common ratio is raised to a power (exponent) to find a specific term in the sequence.
-
Scientific Notation: Used to represent very large or very small numbers concisely, often employing exponents to manage the magnitude of the numbers.
Applying Multiplication: Real-World Examples
The principle of "3 times 3 times 3" is more prevalent in our daily lives than we might initially realize. Here are some examples:
-
Calculating Volume: Imagine a cube with sides of 3 units each (e.g., 3 centimeters, 3 inches, 3 meters). To find its volume, you multiply the length, width, and height: 3 x 3 x 3 = 27 cubic units. This principle applies to various volume calculations, from shipping containers to building materials.
-
Counting Combinations: If you have 3 types of shirts, 3 types of pants, and 3 types of shoes, you have 3 x 3 x 3 = 27 possible outfits. This illustrates the principle of counting combinations, which is useful in probability and statistics.
-
Data Storage: In computer science, data storage is often expressed in powers of 2 (e.g., kilobytes, megabytes, gigabytes). However, the principle of multiplying units remains central to understanding storage capacity and data transfer rates.
-
Investment Growth: Compound interest calculations frequently involve exponential growth, where the principal amount is multiplied repeatedly based on the interest rate and the number of compounding periods. Even though the interest rate might not be exactly 3, the underlying principle of repeated multiplication remains the same.
Exploring Further: Advanced Mathematical Concepts
The simple calculation of 3 x 3 x 3 opens doors to more advanced mathematical concepts:
-
Cubing a Number: Calculating 3³ is essentially finding the cube of 3. Cubing a number refers to raising it to the power of 3. This concept is related to cubic equations and geometric shapes (cubes).
-
Higher-Order Polynomials: Polynomials are mathematical expressions involving variables raised to various powers. The expression 3³ is a simple example of a polynomial, and understanding exponents is crucial to working with higher-order polynomials.
-
Factorials: Factorials (represented by !) involve multiplying a number by all positive integers less than it (e.g., 5! = 5 x 4 x 3 x 2 x 1). While not directly related to 3³, factorials are another way of expressing repeated multiplication and play a significant role in probability and combinatorics.
The Significance of Understanding Multiplication
The seemingly simple problem of "3 times 3 times 3" provides a gateway to understanding fundamental mathematical concepts that extend far beyond basic arithmetic. The ability to perform this calculation and grasp its underlying principles is crucial for success in various fields, from engineering and finance to computer science and data analysis. By understanding the concept of multiplication, exponents, and their applications, individuals are better equipped to tackle complex problems and make informed decisions in various aspects of life. From calculating volumes and combinations to understanding exponential growth and decay, the ability to handle repeated multiplication is a cornerstone of mathematical literacy and practical problem-solving. Mastering this fundamental concept equips us with the tools necessary to navigate a world increasingly reliant on quantitative analysis and data-driven decision-making.
Latest Posts
Latest Posts
-
What Is The Prime Factorization Of 650
May 08, 2025
-
18 8 As A Mixed Number
May 08, 2025
-
Draw All Lines Of Symmetry For The Following Figure
May 08, 2025
-
Where Is 3 5 On A Number Line
May 08, 2025
-
How To Find Domain And Range In A Table
May 08, 2025
Related Post
Thank you for visiting our website which covers about What Is 3 Times 3 Times 3 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.