What Is 3 To The 2nd Power
News Co
Apr 06, 2025 · 5 min read
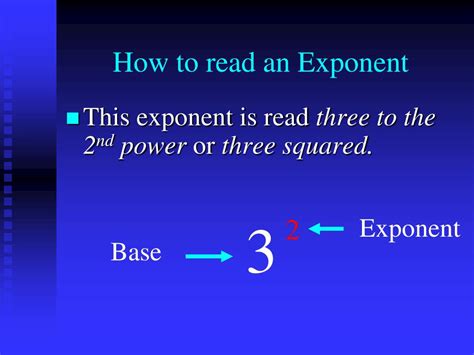
Table of Contents
What is 3 to the 2nd Power? A Comprehensive Exploration of Exponents
Understanding exponents is fundamental to mathematics, forming the bedrock for numerous advanced concepts. This article delves deep into the seemingly simple question: "What is 3 to the 2nd power?" We will not only answer this directly but also explore the broader concept of exponents, their applications, and how to solve related problems. We will use various examples and explain the underlying principles in an accessible and engaging way.
Understanding Exponents: The Basics
Before we tackle 3 to the 2nd power, let's establish a firm understanding of exponents. An exponent, also known as a power or index, is a small number written slightly above and to the right of a base number. It indicates how many times the base number is multiplied by itself. The general form is:
b<sup>n</sup>
Where:
- b represents the base number (the number being multiplied).
- n represents the exponent (the number of times the base is multiplied by itself).
For example, in the expression 2³, '2' is the base and '3' is the exponent. This means 2 multiplied by itself three times: 2 x 2 x 2 = 8.
Calculating 3 to the 2nd Power (3²)
Now, let's address the core question: What is 3 to the 2nd power, or 3²?
Using the definition above, this means 3 multiplied by itself two times:
3² = 3 x 3 = 9
Therefore, 3 to the 2nd power is equal to 9. This is a relatively simple calculation, but understanding the underlying principle is crucial for tackling more complex exponential problems.
Expanding on Exponents: Different Scenarios
While 3² is straightforward, let's explore different scenarios involving exponents to build a more comprehensive understanding.
Exponent of 1:
Any number raised to the power of 1 is simply the number itself. For instance:
- 5¹ = 5
- 10¹ = 10
- x¹ = x
Exponent of 0:
Any non-zero number raised to the power of 0 is always equal to 1. This is a key rule in algebra:
- 7⁰ = 1
- 100⁰ = 1
- (xyz)⁰ = 1 (provided x, y, and z are not zero)
The exception is 0⁰, which is undefined.
Negative Exponents:
A negative exponent indicates the reciprocal of the base raised to the positive power. For example:
- 2⁻² = 1 / 2² = 1 / (2 x 2) = 1/4
- 5⁻¹ = 1/5
- x⁻ⁿ = 1/xⁿ
Fractional Exponents:
Fractional exponents involve roots. For instance, a fractional exponent of 1/2 represents the square root, 1/3 represents the cube root, and so on.
- 9<sup>1/2</sup> = √9 = 3
- 8<sup>1/3</sup> = ∛8 = 2
- x<sup>1/n</sup> = ⁿ√x
Larger Exponents:
As exponents increase, the calculations become more complex, often requiring calculators or computational tools for larger numbers. For instance, calculating 3¹⁰ would involve multiplying 3 by itself ten times:
3¹⁰ = 3 x 3 x 3 x 3 x 3 x 3 x 3 x 3 x 3 x 3 = 59049
Applications of Exponents in Real Life
Exponents are not just abstract mathematical concepts; they have numerous real-world applications across various fields:
Science:
-
Exponential Growth and Decay: Many natural processes, like population growth, radioactive decay, and compound interest, follow exponential patterns. Understanding exponents is essential for modeling and predicting these phenomena. For example, the half-life of a radioactive substance is calculated using exponential functions.
-
Physics: Exponents are integral to formulas in physics, including those related to velocity, acceleration, and energy calculations.
-
Chemistry: In chemistry, exponents are used in stoichiometry calculations and in describing the concentration of substances in chemical reactions.
Finance:
-
Compound Interest: The power of compounding interest relies heavily on exponential growth. Understanding exponents allows for accurate calculation of future values of investments.
-
Financial Modeling: Financial models used to forecast market trends and assess risk often use exponential functions.
Computer Science:
-
Big O Notation: Exponents are used in Big O notation to describe the efficiency of algorithms. It's a crucial tool for comparing algorithm performance in terms of time and space complexity.
-
Data Structures: Understanding exponents is essential when dealing with data structures such as trees and graphs whose complexities are often described using exponential or logarithmic functions.
Solving Problems Involving Exponents
Let's look at a few examples to solidify our understanding of exponents:
Example 1: Simplify the expression: (2³ x 2²)
When multiplying numbers with the same base, we add the exponents:
(2³ x 2²) = 2⁽³⁺²⁾ = 2⁵ = 32
Example 2: Simplify the expression: (4⁵ / 4²)
When dividing numbers with the same base, we subtract the exponents:
(4⁵ / 4²) = 4⁽⁵⁻²⁾ = 4³ = 64
Example 3: Evaluate: (5²)³
When raising a power to another power, we multiply the exponents:
(5²)³ = 5⁽²ˣ³⁾ = 5⁶ = 15625
Beyond the Basics: Advanced Exponential Concepts
This section briefly touches upon more advanced topics related to exponents. Further exploration of these topics would require a dedicated study.
Logarithms:
Logarithms are the inverse of exponents. They answer the question: "To what power must we raise the base to get a specific number?" For example, the logarithm base 10 of 100 (log₁₀100) is 2, because 10² = 100.
Exponential Functions:
Exponential functions are functions where the variable appears in the exponent. They are used to model exponential growth and decay phenomena. The general form is y = abˣ, where 'a' and 'b' are constants.
Exponential Equations:
These equations involve exponents and often require techniques like logarithms to solve for the unknown variable.
Conclusion: Mastering the Power of Exponents
Understanding exponents, starting from the simple concept of 3 to the 2nd power, is essential for success in mathematics and its numerous applications across various fields. While 3² = 9 is a basic calculation, the broader understanding of exponential principles, including different exponent types, applications, and problem-solving techniques, is crucial for building a strong foundation in mathematics and related disciplines. We encourage you to practice these concepts to master the 'power' of exponents and their multifaceted utility. This deep dive has provided a comprehensive overview; further independent study can unlock even deeper insights into the intricacies of this powerful mathematical concept.
Latest Posts
Related Post
Thank you for visiting our website which covers about What Is 3 To The 2nd Power . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.