What Is 30 Percent Of 200
News Co
Mar 20, 2025 · 5 min read
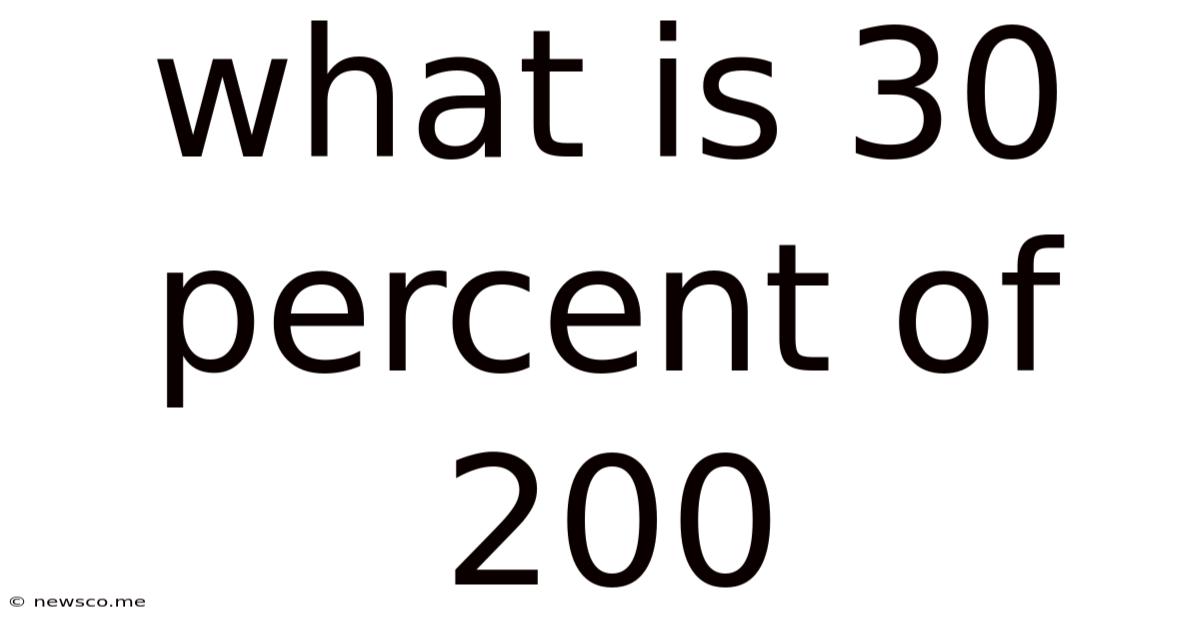
Table of Contents
What is 30 Percent of 200? A Deep Dive into Percentages and Their Applications
Calculating percentages is a fundamental skill with widespread applications in various aspects of life, from everyday finances to complex scientific analyses. This article explores the simple calculation of "What is 30 percent of 200?" and then delves deeper into the concept of percentages, their practical uses, and how to master percentage calculations.
Understanding Percentages: The Foundation
A percentage is a fraction or ratio expressed as a number out of 100. The term "percent" is derived from the Latin words "per centum," meaning "out of one hundred." Therefore, 30 percent means 30 out of 100, or 30/100, which simplifies to 3/10.
This understanding forms the basis of all percentage calculations. To find a percentage of a number, we essentially find that fraction of the number.
Calculating 30 Percent of 200: The Simple Method
The most straightforward way to calculate 30 percent of 200 is to use the following formula:
Percentage × Number = Result
Substituting our values:
0.30 × 200 = 60
Therefore, 30 percent of 200 is 60.
This simple multiplication shows the core principle: converting the percentage to a decimal (30% becomes 0.30) and then multiplying it by the number.
Alternative Methods: Different Approaches, Same Result
While the above method is the most common and efficient, several alternative methods can achieve the same outcome:
-
Fraction Method: As mentioned earlier, 30% is equivalent to 3/10. Therefore, we can calculate (3/10) × 200. This simplifies to 600/10, which equals 60.
-
Proportion Method: Set up a proportion: 30/100 = x/200. Cross-multiplying gives 100x = 6000. Solving for x (by dividing both sides by 100) yields x = 60.
These alternative approaches demonstrate the flexibility of percentage calculations and help reinforce the understanding of the underlying mathematical principles.
Real-World Applications of Percentage Calculations
Understanding percentages is crucial in a multitude of real-life scenarios:
-
Finance: Calculating interest rates, discounts, taxes, tips, and profit margins all rely heavily on percentage calculations. For instance, figuring out the sale price of an item discounted by 30% or determining the total cost after adding a sales tax involves percentage calculations. Understanding these calculations empowers consumers to make informed financial decisions.
-
Science and Statistics: Percentages are essential in expressing probabilities, statistical significance, and data representation. Scientists use percentages to represent experimental results, while statisticians use them to analyze and interpret data, making percentages integral to scientific research and data-driven decision-making.
-
Business and Economics: Businesses use percentages to analyze sales figures, market share, growth rates, and profit margins. Investors use percentages to track returns on investment, while economists use percentages to analyze economic indicators like inflation and unemployment. A firm grasp of percentages is vital for understanding and interpreting economic trends and business performance.
-
Everyday Life: From calculating the percentage of a task completed to understanding nutritional information on food labels (e.g., percentage of daily recommended intake of vitamins), percentages are woven into the fabric of our daily lives. Knowing how to calculate percentages helps us manage our time and make informed choices.
Mastering Percentage Calculations: Tips and Tricks
While calculating simple percentages like 30% of 200 is straightforward, mastering percentage calculations requires understanding and applying several techniques:
-
Converting Percentages to Decimals: Remember, to convert a percentage to a decimal, simply divide the percentage by 100. For example, 75% becomes 0.75.
-
Converting Decimals to Percentages: To convert a decimal to a percentage, multiply the decimal by 100 and add the percent sign. For example, 0.25 becomes 25%.
-
Calculating Percentage Increase/Decrease: To calculate a percentage increase, find the difference between the new value and the original value, divide the difference by the original value, and multiply by 100. Similarly, for percentage decrease, follow the same steps, but the result will be negative.
-
Finding the Original Value: If you know a percentage and the resulting value, you can work backward to find the original value. This often involves setting up a proportion or using algebraic equations.
Advanced Percentage Calculations: Beyond the Basics
Beyond the fundamental calculations, more advanced techniques exist for solving more complex percentage problems. These often involve combining different percentage operations, such as calculating compound interest or finding the percentage change between multiple values.
-
Compound Interest: This involves calculating interest not only on the principal amount but also on the accumulated interest from previous periods. Understanding compound interest is crucial for long-term financial planning, such as investments and loan repayments.
-
Percentage Change: This involves calculating the percentage difference between two values over time, indicating growth or decline. This is widely used in analyzing economic data, business trends, and scientific measurements.
Practical Exercises to Enhance Your Skills
To solidify your understanding and improve your proficiency in percentage calculations, try these exercises:
- What is 15% of 500?
- If an item costs $100 and is discounted by 20%, what is the final price?
- A student scored 80 out of 100 on a test. What is their percentage score?
- A population increased from 1000 to 1200. What is the percentage increase?
- An investment of $5000 earned $500 in interest. What is the percentage return on investment?
By practicing these examples and exploring other percentage-based problems, you'll steadily develop your skills and confidence in handling various percentage calculations.
Conclusion: The Power of Percentages
Understanding and mastering percentage calculations is a valuable skill applicable across numerous domains. From everyday budgeting to complex scientific analyses, the ability to work confidently with percentages provides a significant advantage. By grasping the fundamental principles and practicing various techniques, you can unlock the power of percentages and use them effectively in various aspects of your life. This comprehensive guide not only answers "What is 30 percent of 200?" but also equips you with the knowledge and tools to confidently tackle any percentage-related challenge. Remember, consistent practice is key to mastery. So keep practicing, and you will find that percentage calculations become second nature.
Latest Posts
Latest Posts
-
Find The Point On The Y Axis Which Is Equidistant From
May 09, 2025
-
Is 3 4 Bigger Than 7 8
May 09, 2025
-
Which Of These Is Not A Prime Number
May 09, 2025
-
What Is 30 Percent Off Of 80 Dollars
May 09, 2025
-
Are Alternate Exterior Angles Always Congruent
May 09, 2025
Related Post
Thank you for visiting our website which covers about What Is 30 Percent Of 200 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.