What Is 66 2 3 As A Decimal
News Co
May 08, 2025 · 4 min read
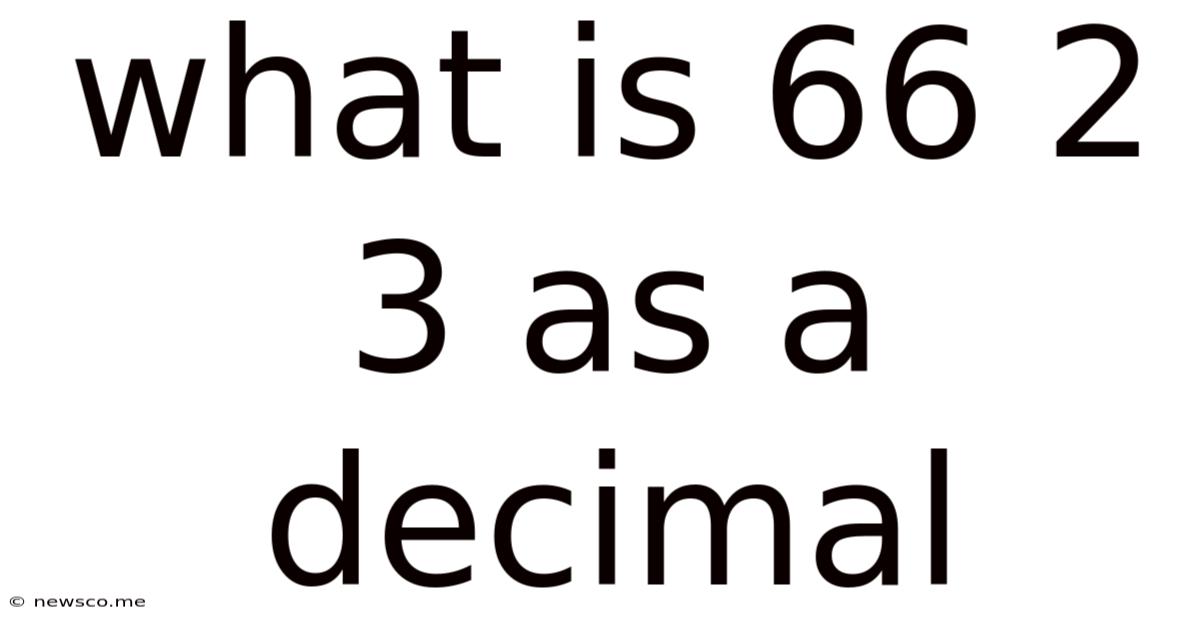
Table of Contents
What is 66 2/3 as a Decimal? A Comprehensive Guide
Understanding how to convert fractions to decimals is a fundamental skill in mathematics. This comprehensive guide will delve into the process of converting the mixed number 66 2/3 into its decimal equivalent, exploring various methods and providing a clear understanding of the underlying concepts. We'll also touch upon the practical applications of this conversion and offer additional resources for further learning.
Understanding Mixed Numbers and Decimals
Before diving into the conversion, let's briefly review the concepts of mixed numbers and decimals.
A mixed number combines a whole number and a fraction, such as 66 2/3. It represents a value greater than one.
A decimal is a number expressed in base-10, using a decimal point to separate the whole number part from the fractional part. For example, 66.666... is a decimal.
Method 1: Converting the Fraction to a Decimal, then Adding the Whole Number
This is arguably the most straightforward method. We'll first convert the fraction 2/3 into a decimal and then add the whole number 66.
Step 1: Divide the Numerator by the Denominator
To convert the fraction 2/3 to a decimal, we perform the division: 2 ÷ 3.
This division results in a repeating decimal: 0.66666... This is often represented as 0.6̅, where the bar indicates that the digit 6 repeats infinitely.
Step 2: Add the Whole Number
Now, we add the whole number part (66) to the decimal equivalent of the fraction (0.66666...):
66 + 0.66666... = 66.66666...
Therefore, 66 2/3 as a decimal is 66.6̅ or approximately 66.67 when rounded to two decimal places.
Method 2: Converting the Mixed Number to an Improper Fraction, then to a Decimal
This method involves first converting the mixed number into an improper fraction, and then dividing the numerator by the denominator.
Step 1: Convert to an Improper Fraction
To convert 66 2/3 to an improper fraction, we multiply the whole number (66) by the denominator (3), add the numerator (2), and keep the same denominator (3):
(66 * 3) + 2 = 200
So, 66 2/3 becomes 200/3.
Step 2: Divide the Numerator by the Denominator
Now, we divide the numerator (200) by the denominator (3):
200 ÷ 3 = 66.66666...
This again gives us the repeating decimal 66.6̅.
Understanding Repeating Decimals
The result, 66.6̅, is a repeating decimal. This means the digit 6 repeats infinitely. It's crucial to understand the implications of this: We can never write the entire decimal representation because it goes on forever. However, for practical purposes, we often round the decimal to a specific number of decimal places. Rounding 66.6̅ to two decimal places gives us 66.67.
Practical Applications of Decimal Conversions
Converting fractions to decimals is essential in many real-world applications, including:
-
Finance: Calculating percentages, interest rates, and proportions of money. For example, if a discount is 2/3 off, knowing the decimal equivalent (0.666...) is crucial for quick calculations.
-
Engineering and Science: Precision measurements often require decimal representations. Engineering calculations frequently utilize decimal values for accuracy.
-
Cooking and Baking: Recipes sometimes use fractional measurements, which often need to be converted to decimals for more accurate measurements using digital scales.
-
Data Analysis and Statistics: Decimal representations are standard in data analysis and statistical computations, simplifying calculations and interpretations.
Rounding Repeating Decimals
As we've seen, converting 66 2/3 to a decimal results in a repeating decimal. In many situations, we need to round this decimal to a specific number of decimal places for practical use. The common rounding rules apply:
- Rounding to one decimal place: 66.7
- Rounding to two decimal places: 66.67
- Rounding to three decimal places: 66.667
Choosing the Right Method
Both methods described above – converting the fraction first or converting to an improper fraction first – yield the same result. Choose the method that you find more intuitive and comfortable. The key is to understand the underlying principles of fraction-to-decimal conversion.
Further Exploration and Resources
For those seeking to deepen their understanding of fractions, decimals, and other mathematical concepts, there are numerous resources available:
-
Online Math Tutorials: Many websites offer interactive tutorials and practice exercises on fraction-to-decimal conversion.
-
Math Textbooks: Comprehensive math textbooks provide detailed explanations and examples of fraction-to-decimal conversions and other mathematical concepts.
-
Khan Academy: This online learning platform offers free courses and videos on a wide range of mathematical topics, including fractions and decimals.
Conclusion
Converting the mixed number 66 2/3 to a decimal results in the repeating decimal 66.6̅. Understanding the process of converting fractions to decimals, including handling repeating decimals and rounding, is crucial for various applications across different fields. By mastering this fundamental skill, you'll enhance your mathematical abilities and improve your problem-solving skills in various real-world scenarios. Remember to practice regularly to build confidence and proficiency. Choosing the right method for you and understanding the practical implications are key to effectively working with these types of conversions.
Latest Posts
Latest Posts
-
Two Angles Whose Sides Are Opposite Rays Are Called
May 08, 2025
-
Surface Area Is Always Measured In Square Units True False
May 08, 2025
-
What Is The Prime Factorization Of 650
May 08, 2025
-
18 8 As A Mixed Number
May 08, 2025
-
Draw All Lines Of Symmetry For The Following Figure
May 08, 2025
Related Post
Thank you for visiting our website which covers about What Is 66 2 3 As A Decimal . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.