What Is A Common Multiple Of 5 And 9
News Co
Apr 07, 2025 · 5 min read
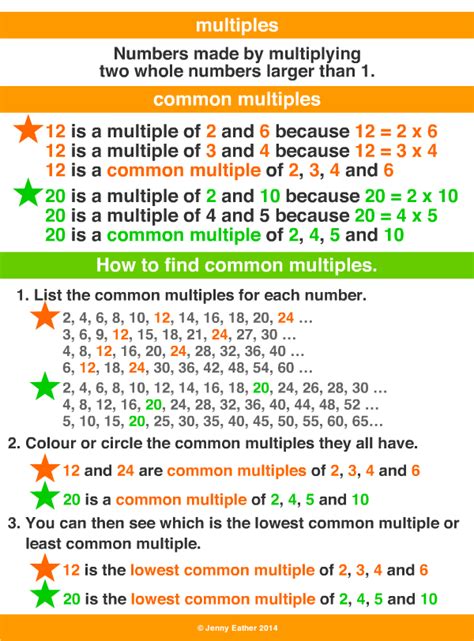
Table of Contents
What is a Common Multiple of 5 and 9? A Deep Dive into Number Theory
Finding the common multiples of two numbers might seem like a simple mathematical task, suitable only for elementary school students. However, understanding the concept of common multiples, particularly the least common multiple (LCM), delves into fundamental principles of number theory with surprisingly broad applications in various fields. This article will explore the concept of common multiples, focusing specifically on the common multiples of 5 and 9, and illustrating its relevance beyond simple arithmetic.
Understanding Multiples
Before we dive into common multiples, let's establish a clear understanding of what a multiple is. A multiple of a number is the product of that number and any whole number (including zero). For instance:
- Multiples of 5: 0, 5, 10, 15, 20, 25, 30, 35, 40, 45, 50… and so on, extending infinitely.
- Multiples of 9: 0, 9, 18, 27, 36, 45, 54, 63, 72, 81, 90… and so on, also extending infinitely.
Notice that both lists are infinite sequences. Any number multiplied by 5 will produce a multiple of 5, and similarly for 9.
Common Multiples: Where the Paths Converge
A common multiple of two or more numbers is a number that is a multiple of all those numbers. Looking at our lists of multiples above, we can identify numbers that appear in both:
- Common Multiples of 5 and 9: 0, 45, 90, 135, 180, 225, 270… and so on.
This list, like the individual lists of multiples, also extends infinitely. Any number that is a multiple of both 5 and 9 is a common multiple.
The Least Common Multiple (LCM): Finding the Smallest
Among the infinite set of common multiples, there's one that holds particular significance: the Least Common Multiple (LCM). As the name suggests, the LCM is the smallest positive common multiple of two or more numbers, excluding zero. In our case, the LCM of 5 and 9 is 45.
Methods for Finding the LCM
Several methods exist for calculating the LCM of two numbers. Let's examine a few:
1. Listing Multiples:
This is a straightforward method, suitable for smaller numbers. We simply list the multiples of each number until we find the smallest common multiple. As demonstrated above, this method reveals 45 as the LCM of 5 and 9. However, this approach becomes less practical with larger numbers.
2. Prime Factorization:
This method leverages the concept of prime factorization. First, we find the prime factorization of each number:
- 5: 5 (5 is a prime number)
- 9: 3 x 3 = 3²
Next, we identify the highest power of each prime factor present in either factorization. In this case, we have 3² and 5.
Finally, we multiply these highest powers together: 3² x 5 = 9 x 5 = 45. Therefore, the LCM of 5 and 9 is 45. This method is efficient even for larger numbers.
3. Using the Formula: LCM(a, b) = (|a x b|) / GCD(a, b)
This method utilizes the Greatest Common Divisor (GCD) of the two numbers. The GCD is the largest number that divides both numbers without leaving a remainder. The GCD of 5 and 9 is 1 (as they share no common factors other than 1).
Using the formula: LCM(5, 9) = (|5 x 9|) / GCD(5, 9) = 45 / 1 = 45.
Real-World Applications of LCM
While finding common multiples might seem abstract, the concept has practical applications in various scenarios:
1. Scheduling and Timing:
Imagine two buses that arrive at a bus stop at different intervals. One bus arrives every 5 minutes, and the other every 9 minutes. To find when both buses arrive simultaneously, you need to find the common multiples of 5 and 9. The LCM, 45 minutes, indicates the time interval when both buses arrive together.
2. Fraction Operations:
When adding or subtracting fractions with different denominators, finding the LCM of the denominators is crucial. The LCM becomes the least common denominator (LCD), allowing for a simplified calculation.
3. Gear Ratios and Mechanical Systems:
In engineering and mechanics, the LCM is used to calculate gear ratios and synchronize rotational speeds in various systems.
4. Cyclic Patterns and Repetition:
The LCM helps determine when repeating patterns or cycles will coincide. For example, in musical compositions with repeating melodic phrases of different lengths, the LCM determines when both phrases will simultaneously end.
5. Task Scheduling and Project Management:
In project management, the LCM can aid in scheduling tasks that must be completed at specific intervals, ensuring efficient resource allocation and project completion.
Beyond the Basics: Extending the Concept
The concept of common multiples and LCM extends beyond two numbers. You can find the LCM of three or more numbers using similar methods, especially the prime factorization method. The prime factorization method remains the most efficient way to find the LCM for larger numbers and multiple numbers.
Conclusion: The Power of Understanding Common Multiples
The seemingly simple concept of common multiples, particularly the LCM, reveals a surprising depth of mathematical understanding. Its applications extend far beyond elementary arithmetic, proving invaluable in various fields requiring precise timing, scheduling, and synchronization. Understanding how to find the LCM, whether through listing, prime factorization, or using the GCD formula, is a fundamental skill with practical and far-reaching implications. The exploration of common multiples serves as a potent example of how seemingly basic mathematical concepts underpin complex systems and processes. From bus schedules to engineering marvels, the LCM plays its quiet yet crucial role in making the world work. Remember that mastering this simple concept opens doors to a deeper understanding of number theory and its practical application in the real world. By using the prime factorization method, one can even find the LCM of very large numbers with efficiency. This underscores the importance of a strong foundation in basic arithmetic principles.
Latest Posts
Related Post
Thank you for visiting our website which covers about What Is A Common Multiple Of 5 And 9 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.