What Is A Factor Of 81
News Co
Apr 05, 2025 · 5 min read
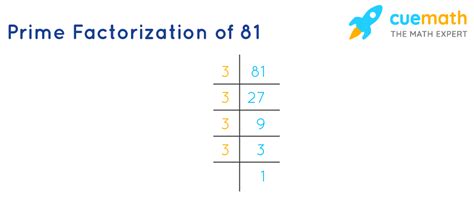
Table of Contents
What is a Factor of 81? A Deep Dive into Divisibility and Number Theory
Finding the factors of a number might seem like a simple arithmetic exercise, but it opens the door to a fascinating world of number theory and mathematical concepts. This comprehensive guide delves into the question, "What is a factor of 81?", exploring not only the answer but also the underlying principles and related mathematical ideas. We'll cover various methods for finding factors, discuss their significance in different areas of mathematics, and even touch upon some more advanced concepts.
Understanding Factors: The Building Blocks of Numbers
Before we tackle the factors of 81 specifically, let's establish a solid understanding of what a factor is. In simple terms, a factor (also known as a divisor) of a number is any integer that divides that number evenly, leaving no remainder. For example, 2 is a factor of 10 because 10 divided by 2 equals 5 with no remainder.
Key Characteristics of Factors
- Divisibility: The core characteristic of a factor is its ability to divide the number without leaving a remainder. This is often represented mathematically as
a | b, meaning 'a divides b'. - Pairs: Factors often come in pairs. If 'a' is a factor of 'b', then 'b/a' is also a factor of 'b'. For instance, since 3 is a factor of 12, then 12/3 = 4 is also a factor.
- One and the Number Itself: Every number has at least two factors: 1 and the number itself. These are considered trivial factors.
- Prime Factors: A prime factor is a factor that is also a prime number (a number greater than 1 that has only two factors: 1 and itself). The prime factorization of a number is the unique representation of that number as a product of its prime factors.
Finding the Factors of 81: Different Approaches
Now, let's determine the factors of 81. We can employ several methods:
Method 1: Systematic Division
The most straightforward approach is to systematically divide 81 by integers starting from 1 and increasing until we reach 81. We're looking for divisions that result in a whole number quotient.
- 81 ÷ 1 = 81
- 81 ÷ 3 = 27
- 81 ÷ 9 = 9
- 81 ÷ 27 = 3
- 81 ÷ 81 = 1
This reveals the factors of 81: 1, 3, 9, 27, and 81. Notice how they appear in pairs (1 and 81, 3 and 27), except for 9, which is paired with itself because 9 x 9 = 81.
Method 2: Prime Factorization
This method leverages the fundamental theorem of arithmetic, which states that every integer greater than 1 can be uniquely represented as a product of prime numbers.
-
Find the prime factorization of 81: 81 is divisible by 3, so we can write 81 = 3 x 27. Further, 27 is also divisible by 3 (27 = 3 x 9), and 9 is 3 x 3. Therefore, the prime factorization of 81 is 3 x 3 x 3 x 3 = 3⁴.
-
Generate factors from the prime factorization: To find all factors, we consider all possible combinations of the prime factors. Since we have four 3s, the combinations are:
- 3⁰ = 1
- 3¹ = 3
- 3² = 9
- 3³ = 27
- 3⁴ = 81
These combinations give us the same set of factors as the systematic division method: 1, 3, 9, 27, and 81.
Method 3: Factor Tree (Visual Representation of Prime Factorization)
A factor tree provides a visual way to represent the prime factorization process.
81
/ \
3 27
/ \
3 9
/ \
3 3
Following the branches down, we again arrive at the prime factorization 3⁴, leading to the same set of factors.
Significance of Factors in Mathematics and Beyond
Understanding factors is crucial in various mathematical fields and practical applications:
Number Theory:
- Greatest Common Divisor (GCD): Factors are fundamental to finding the GCD of two or more numbers. The GCD is the largest number that divides all the given numbers without leaving a remainder. For instance, the GCD of 81 and 54 can be determined by examining their factors.
- Least Common Multiple (LCM): Factors play a role in determining the LCM, which is the smallest number that is a multiple of all the given numbers.
- Modular Arithmetic: Factors are essential in understanding congruences and modular arithmetic, used extensively in cryptography and computer science.
Algebra:
- Factoring Polynomials: The concept of factors extends to algebraic expressions. Factoring polynomials involves expressing a polynomial as a product of simpler polynomials. This is a critical step in solving equations and simplifying expressions.
Real-world Applications:
- Dividing Resources: Factors help in fairly dividing resources or objects among a group of people. For example, dividing 81 candies among 9 children.
- Geometry: Factors are used in calculating areas and volumes of geometric shapes. Determining the dimensions of a square with an area of 81 square units involves finding the square root of 81 (which is a factor).
- Computer Science: Algorithms in computer science often rely on factoring to optimize processes or solve computational problems.
Advanced Concepts Related to Factors
While finding the factors of 81 is relatively simple, the concept expands into more sophisticated mathematical areas:
Perfect Squares and Cubes:
81 is a perfect square (9²) and a perfect sixth power (3⁶). Understanding perfect powers helps in quickly identifying some factors.
Divisibility Rules:
Divisibility rules provide shortcuts for determining whether a number is divisible by specific integers. For example, a number is divisible by 3 if the sum of its digits is divisible by 3 (8 + 1 = 9, which is divisible by 3, indicating 81 is divisible by 3).
Number of Factors:
A formula exists to calculate the number of factors a number possesses based on its prime factorization. For 81 (3⁴), the number of factors is (4+1) = 5.
Conclusion: The Richness of Factors
The seemingly simple question "What is a factor of 81?" unravels into a journey through fundamental mathematical concepts and their wide-ranging applications. Understanding factors is not just about simple division; it's a gateway to appreciating the intricate structure and beauty of numbers, paving the way for further exploration into number theory, algebra, and other mathematical disciplines. The factors of 81 – 1, 3, 9, 27, and 81 – are more than just numbers; they are building blocks that reveal much about the nature of mathematics itself. By mastering the concept of factors, we unlock a deeper understanding of the mathematical world around us.
Latest Posts
Related Post
Thank you for visiting our website which covers about What Is A Factor Of 81 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.