What Is Prime Factorization Of 225
News Co
Apr 06, 2025 · 5 min read
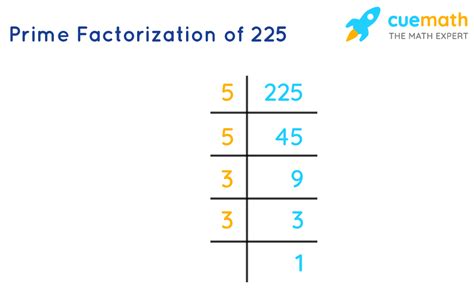
Table of Contents
What is Prime Factorization of 225? A Deep Dive into Number Theory
Prime factorization is a fundamental concept in number theory, forming the bedrock for various mathematical applications. Understanding prime factorization allows us to simplify complex mathematical operations and unlock deeper insights into the structure of numbers. This article delves into the prime factorization of 225, explaining the process, its significance, and related concepts. We'll explore the method step-by-step and discuss the broader implications of this seemingly simple factorization.
Understanding Prime Numbers and Factorization
Before we tackle the prime factorization of 225, let's define key terms:
-
Prime Number: A prime number is a natural number greater than 1 that has no positive divisors other than 1 and itself. Examples include 2, 3, 5, 7, 11, and so on. Prime numbers are the building blocks of all other integers.
-
Composite Number: A composite number is a positive integer that has at least one divisor other than 1 and itself. In essence, it's a number that can be factored into smaller positive integers.
-
Prime Factorization: Prime factorization (or integer factorization) is the process of expressing a composite number as a product of its prime factors. This representation is unique for every composite number (excluding the order of the factors).
Finding the Prime Factorization of 225
Now, let's find the prime factorization of 225. We can accomplish this using various methods, but a common and straightforward approach is through repeated division by prime numbers.
-
Start with the smallest prime number: The smallest prime number is 2. However, 225 is not divisible by 2 (it's an odd number).
-
Move to the next prime number: The next prime number is 3. Let's check if 225 is divisible by 3. The sum of the digits of 225 (2 + 2 + 5 = 9) is divisible by 3, indicating that 225 is also divisible by 3. Dividing 225 by 3 gives us 75.
-
Continue the process: Now we work with 75. 75 is also divisible by 3 (7 + 5 = 12, which is divisible by 3). Dividing 75 by 3 gives us 25.
-
Identify the remaining prime factor: We are left with 25. 25 is not divisible by 3, but it is divisible by 5 (the next prime number). Dividing 25 by 5 gives us 5.
-
The final prime factor: We are left with 5, which is itself a prime number.
Therefore, the prime factorization of 225 is 3 x 3 x 5 x 5, which can be written more concisely as 3² x 5².
Different Methods for Prime Factorization
While the repeated division method is intuitive, other methods can also be employed to determine the prime factorization of a number. Let's explore a couple of them:
1. Factor Tree Method: This visual method uses a tree-like structure to represent the factorization process. You start with the number at the top and branch out, showing the factors at each step until you reach only prime numbers at the ends of the branches.
For 225, the factor tree would look like this:
225
/ \
3 75
/ \
3 25
/ \
5 5
This clearly shows the prime factors 3, 3, 5, and 5.
2. Using a Factorization Algorithm: For larger numbers, more sophisticated algorithms like trial division, Pollard's rho algorithm, or the general number field sieve are used. These algorithms are computationally intensive and often used in cryptography. While these aren't necessary for a number as small as 225, understanding their existence is crucial for appreciating the scale and complexity involved in factorizing much larger numbers.
The Significance of Prime Factorization
The prime factorization of a number might seem like a simple mathematical exercise, but it holds significant importance in various areas:
-
Cryptography: The security of many encryption algorithms relies on the difficulty of factoring large composite numbers into their prime factors. The RSA algorithm, widely used for secure online communication, is a prime example. The difficulty in factoring large numbers ensures the confidentiality of sensitive information.
-
Number Theory: Prime factorization is a fundamental concept in number theory, providing insights into the structure and properties of integers. It's used to prove theorems, solve Diophantine equations, and explore various number-theoretic relationships.
-
Modular Arithmetic: Understanding prime factorization is essential in modular arithmetic, which has applications in computer science, cryptography, and other fields.
-
Simplifying Fractions: Prime factorization helps simplify fractions. By expressing the numerator and denominator as a product of their prime factors, common factors can be canceled, resulting in a simplified fraction.
-
Least Common Multiple (LCM) and Greatest Common Divisor (GCD): Prime factorization makes finding the LCM and GCD of two or more numbers significantly easier.
Applications Beyond the Basics: Exploring the Implications of 225's Prime Factorization
The prime factorization of 225 (3² x 5²) reveals several interesting properties:
-
Perfect Square: Since 225 is expressed as the product of squares of prime numbers, it's a perfect square (15 x 15 = 225). This property has implications in geometry (e.g., constructing squares with side lengths related to 225) and other mathematical applications.
-
Divisors: Knowing the prime factorization allows us to quickly determine the number of divisors of 225. The number of divisors is calculated by adding 1 to each exponent in the prime factorization and multiplying the results: (2+1)(2+1) = 9. Therefore, 225 has nine divisors: 1, 3, 5, 9, 15, 25, 45, 75, and 225.
-
Relationship to other numbers: Understanding the prime factorization of 225 can help in exploring its relationships with other numbers. For instance, we can easily determine if 225 is divisible by other numbers simply by checking if those numbers contain the same prime factors (3 and 5).
Conclusion: The Enduring Importance of Prime Factorization
The prime factorization of 225, while seemingly simple at first glance, illustrates a core concept in number theory with wide-ranging implications. From the foundations of mathematics to the underpinnings of modern cryptography, the ability to decompose composite numbers into their prime factors is crucial. The methods presented here – repeated division, factor trees, and the mention of more advanced algorithms – provide a comprehensive approach to understanding and applying this fundamental principle. Mastering prime factorization opens doors to deeper explorations within mathematics and its many real-world applications. The seemingly simple number 225, therefore, serves as a perfect microcosm of a broader, powerful concept.
Latest Posts
Related Post
Thank you for visiting our website which covers about What Is Prime Factorization Of 225 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.