What Is Square Root Of 35
News Co
Apr 06, 2025 · 4 min read
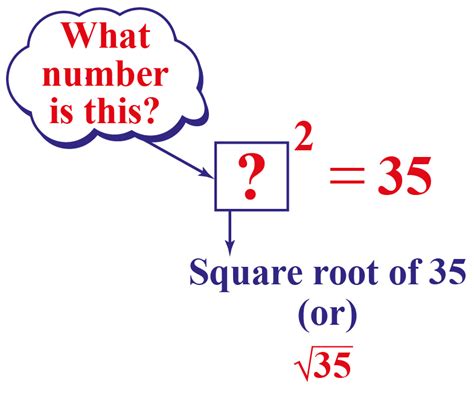
Table of Contents
What is the Square Root of 35? A Deep Dive into Irrational Numbers
The question, "What is the square root of 35?" seems simple enough. However, delving into this seemingly straightforward mathematical concept reveals a fascinating journey into the world of irrational numbers and their properties. This article will explore the square root of 35, examining its nature, how to approximate its value, its significance in various mathematical contexts, and its applications in real-world scenarios.
Understanding Square Roots
Before we tackle the specific case of the square root of 35, let's establish a fundamental understanding of square roots. The square root of a number 'x' is a value that, when multiplied by itself, equals 'x'. In mathematical notation, this is represented as √x. For example, the square root of 9 (√9) is 3, because 3 multiplied by itself (3 x 3 = 9) equals 9.
The Nature of the Square Root of 35
Unlike the square root of 9, which is a whole number, the square root of 35 (√35) is an irrational number. This means it cannot be expressed as a simple fraction (a ratio of two integers). Its decimal representation is non-terminating and non-repeating, meaning it continues infinitely without ever settling into a repeating pattern.
This characteristic distinguishes irrational numbers from rational numbers, which can be expressed as fractions. Examples of rational numbers include 1/2, 3/4, and even whole numbers like 5 (which can be expressed as 5/1).
The irrationality of √35 is a consequence of 35 not being a perfect square. A perfect square is a number that is the product of an integer multiplied by itself. For example, 9, 16, and 25 are perfect squares (3², 4², and 5² respectively). Since 35 is not the product of any integer multiplied by itself, its square root is irrational.
Approximating the Square Root of 35
While we cannot express √35 exactly as a fraction or a terminating decimal, we can approximate its value using various methods:
1. Using a Calculator
The simplest approach is to use a calculator. Most calculators have a square root function (√) that provides a decimal approximation. The calculator will give a result like 5.916079783... This is an approximation, as the actual value continues infinitely.
2. The Babylonian Method (or Heron's Method)
This iterative method provides increasingly accurate approximations. The process starts with an initial guess and then refines it through repeated calculations:
- Make an initial guess: Let's guess 6, as 6 x 6 = 36, which is close to 35.
- Improve the guess: Divide 35 by the initial guess (35/6 ≈ 5.833).
- Average: Average the initial guess and the result from step 2: (6 + 5.833)/2 ≈ 5.9165
- Repeat: Use the average as the new guess and repeat steps 2 and 3. With each iteration, the approximation becomes more accurate.
After several iterations, the Babylonian method provides a very close approximation to the square root of 35.
3. Using Linear Interpolation
This method uses the known square roots of numbers close to 35. We know √36 = 6 and √25 = 5. Since 35 lies between 25 and 36, we can linearly interpolate:
- The difference between 36 and 25 is 11.
- 35 is 10 units away from 25.
- Therefore, the square root of 35 is approximately 5 + (10/11) * (6-5) = 5.909
This method offers a quick but less precise approximation than the Babylonian method.
Significance in Mathematics
The square root of 35, despite being irrational, holds significance in various mathematical contexts:
- Geometry: It appears in calculations involving triangles and other geometric shapes. For example, if a right-angled triangle has legs of certain lengths, the hypotenuse might involve √35.
- Algebra: It's used in solving quadratic equations and other algebraic expressions.
- Number Theory: Its irrationality contributes to our understanding of the properties of different number systems.
- Calculus: It appears in various calculus problems, including integrals and derivatives.
Real-World Applications
While it may not be immediately apparent, the square root of 35, along with other irrational numbers, finds its way into real-world applications:
- Engineering: Calculations involving distances, areas, and volumes often involve irrational numbers. Civil engineers might use square roots to calculate the length of a diagonal support beam.
- Physics: Many physics equations involve square roots, particularly those related to motion, energy, and forces.
- Computer Graphics: The rendering of curves and shapes often relies on calculations involving irrational numbers like the square root of 35.
- Finance: Although less directly, irrational numbers play a role in financial models that involve complex calculations and statistical analysis.
Conclusion: Embracing the Irrational
The square root of 35, while not a neat whole number or simple fraction, is a perfectly valid and meaningful mathematical entity. Its irrationality highlights the richness and complexity of the number system. Understanding its nature, approximating its value, and recognizing its presence in various mathematical and real-world contexts allows us to appreciate the broader implications of this seemingly simple mathematical concept. The methods outlined above, from using a calculator to applying more sophisticated algorithms, demonstrate the diverse ways we can approach and understand irrational numbers like √35. Ultimately, embracing the irrational allows for a deeper understanding and appreciation of the elegance and power of mathematics.
Latest Posts
Related Post
Thank you for visiting our website which covers about What Is Square Root Of 35 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.