What Is The Measure Of Angle Abc
News Co
Apr 03, 2025 · 6 min read
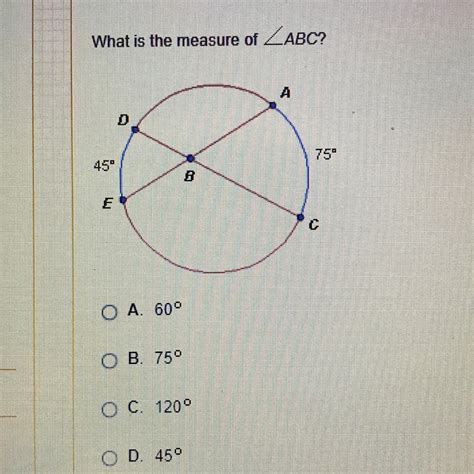
Table of Contents
What is the Measure of Angle ABC? A Comprehensive Guide to Angle Measurement
Determining the measure of angle ABC hinges on understanding fundamental geometry concepts and employing appropriate techniques. This comprehensive guide will explore various methods for calculating angle measures, focusing on angle ABC within different geometric contexts. We'll cover everything from basic angle properties to advanced trigonometric applications.
Understanding Angles and Their Measurement
Before we delve into calculating the measure of angle ABC, let's establish a solid foundation in angle terminology and measurement.
Defining Angles
An angle is formed by two rays that share a common endpoint, called the vertex. In the context of angle ABC, point B is the vertex, and rays BA and BC form the two sides of the angle. Angles are typically measured in degrees (°), although radians are also used in certain mathematical applications.
Types of Angles
Understanding different types of angles is crucial for solving problems involving angle measurement. Key angle types include:
- Acute Angle: An angle measuring less than 90°.
- Right Angle: An angle measuring exactly 90°. Represented by a small square at the vertex.
- Obtuse Angle: An angle measuring greater than 90° but less than 180°.
- Straight Angle: An angle measuring exactly 180°. Forms a straight line.
- Reflex Angle: An angle measuring greater than 180° but less than 360°.
Measuring Angles
Angles can be measured using a protractor, a tool specifically designed for angle measurement. To measure angle ABC using a protractor, place the protractor's center point on vertex B, align one ray (e.g., BA) with the 0° mark, and read the degree measure where the other ray (BC) intersects the protractor's scale.
Determining the Measure of Angle ABC in Different Contexts
The method for determining the measure of angle ABC depends heavily on the context – the type of geometric figure it's part of and the information provided. Let's explore several scenarios:
1. Angle ABC in a Triangle
If angle ABC is part of a triangle, we can utilize various properties of triangles to determine its measure.
a) Using the Sum of Angles in a Triangle
The sum of the interior angles in any triangle always equals 180°. If we know the measures of angles BAC and BCA, we can easily calculate the measure of angle ABC:
Angle ABC = 180° - Angle BAC - Angle BCA
b) Using Isosceles or Equilateral Triangles
If triangle ABC is an isosceles triangle, two of its angles are equal. Knowing the measure of one of these equal angles and the third angle allows us to calculate angle ABC. If triangle ABC is an equilateral triangle, all three angles are equal and each measures 60°.
c) Using Trigonometric Ratios (Sine, Cosine, Tangent)
In the case of a non-right-angled triangle, we can use the sine rule or cosine rule to determine the measure of angle ABC if the lengths of the sides of the triangle are known.
- Sine Rule: a/sin(A) = b/sin(B) = c/sin(C)
- Cosine Rule: a² = b² + c² - 2bc cos(A)
Where a, b, and c are the lengths of the sides opposite angles A, B, and C respectively.
2. Angle ABC in a Polygon
If angle ABC is part of a polygon (a closed figure with three or more sides), the calculation depends on the polygon's type and the known angles.
a) Using the Sum of Interior Angles of a Polygon
The sum of the interior angles of an n-sided polygon is given by the formula:
(n-2) * 180°
Once we know the sum of the interior angles and the measures of the other angles in the polygon, we can determine angle ABC.
b) Regular Polygons
If the polygon is a regular polygon (all sides and angles are equal), we can simply divide the sum of interior angles by the number of sides to find the measure of each angle, including angle ABC.
3. Angle ABC as a Part of Intersecting Lines
If angle ABC is formed by intersecting lines, we can use properties of vertically opposite angles and adjacent angles on a straight line to determine its measure.
- Vertically Opposite Angles: Vertically opposite angles formed by intersecting lines are always equal.
- Adjacent Angles on a Straight Line: Adjacent angles on a straight line add up to 180°.
Using these properties, if the measure of one of the related angles is known, the measure of angle ABC can be easily calculated.
4. Angle ABC in a Circle
If angle ABC is an inscribed angle in a circle (its vertex lies on the circle and its sides are chords), its measure is half the measure of the intercepted arc. If angle ABC is a central angle (its vertex is the center of the circle), its measure is equal to the measure of the intercepted arc.
Advanced Techniques and Applications
Beyond the basic methods discussed above, more advanced techniques can be employed to determine the measure of angle ABC, depending on the specific geometric configuration:
- Coordinate Geometry: If the coordinates of points A, B, and C are known, we can use vector methods or the dot product formula to determine the measure of angle ABC.
- Calculus: In situations involving curves and tangents, calculus techniques can be used to determine angles.
- Computer-Aided Design (CAD) Software: CAD software provides tools to precisely measure angles in complex geometric figures.
Practical Examples
Let's illustrate the calculation of angle ABC with a few examples:
Example 1: Triangle ABC
Suppose triangle ABC has angles BAC = 50° and BCA = 70°. To find angle ABC, we use the sum of angles in a triangle:
Angle ABC = 180° - 50° - 70° = 60°
Example 2: Intersecting Lines
Let's say two lines intersect, forming angles. If one of the angles is 110°, its vertically opposite angle will also be 110°. The adjacent angle will be 180° - 110° = 70°.
Example 3: Regular Pentagon
A regular pentagon has five equal angles. Using the formula for the sum of interior angles: (5-2) * 180° = 540°. Each angle in the regular pentagon measures 540° / 5 = 108°. If angle ABC is one of the angles in the pentagon, then angle ABC = 108°.
Conclusion
Determining the measure of angle ABC involves a multifaceted approach, relying on a thorough understanding of geometric principles and appropriate problem-solving techniques. From basic angle properties to advanced trigonometric and computational methods, the approach depends entirely on the given context and available information. By mastering these techniques, one can confidently tackle a wide range of angle measurement problems in various geometric settings. Remember to carefully analyze the given information, identify the relevant geometric properties, and choose the most suitable method to accurately calculate the measure of angle ABC.
Latest Posts
Related Post
Thank you for visiting our website which covers about What Is The Measure Of Angle Abc . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.