What Is The Mixed Number For 13/12
News Co
Mar 17, 2025 · 5 min read
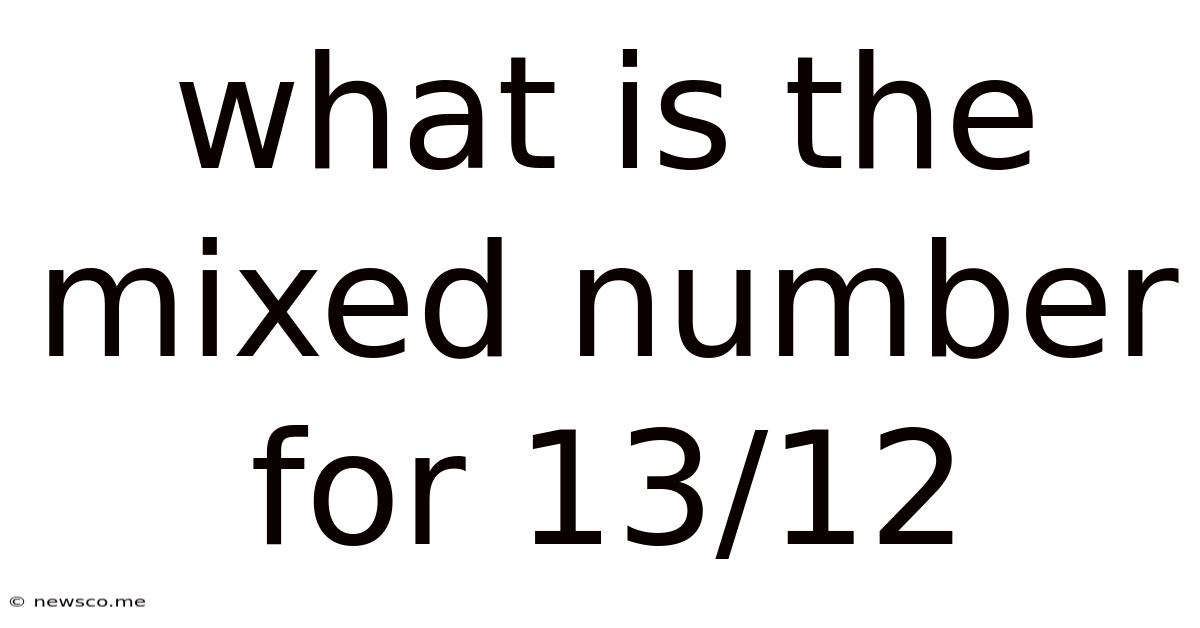
Table of Contents
What is the Mixed Number for 13/12? A Comprehensive Guide to Fractions and Mixed Numbers
Understanding fractions and mixed numbers is fundamental to mathematics. This comprehensive guide will not only answer the question, "What is the mixed number for 13/12?", but also delve into the underlying concepts, providing you with a solid foundation in fractional arithmetic. We'll explore various methods for converting improper fractions (like 13/12) to mixed numbers, and offer practical examples to solidify your understanding. This guide is designed for students, educators, and anyone looking to refresh their knowledge of this important mathematical topic.
Understanding Fractions and Mixed Numbers
Before diving into the conversion, let's clarify the terminology:
-
Fraction: A fraction represents a part of a whole. It's written as a numerator (top number) over a denominator (bottom number), e.g., 13/12. The numerator indicates the number of parts you have, and the denominator indicates the total number of equal parts the whole is divided into.
-
Improper Fraction: An improper fraction is a fraction where the numerator is greater than or equal to the denominator. 13/12 is an example of an improper fraction.
-
Mixed Number: A mixed number combines a whole number and a proper fraction. It represents a quantity that is greater than one. For example, 1 ¼ is a mixed number.
-
Proper Fraction: A proper fraction is a fraction where the numerator is less than the denominator, e.g., 1/4 or 3/8.
Converting 13/12 to a Mixed Number: The Step-by-Step Process
The core concept behind converting an improper fraction to a mixed number lies in dividing the numerator by the denominator. Here's how to convert 13/12:
-
Divide the numerator by the denominator: Divide 13 by 12.
13 ÷ 12 = 1 with a remainder of 1
-
The whole number part: The quotient (the result of the division) becomes the whole number part of the mixed number. In this case, the quotient is 1.
-
The fractional part: The remainder becomes the numerator of the fractional part, and the denominator remains the same. The remainder is 1, and the denominator is 12. Therefore, the fractional part is 1/12.
-
Combine the whole number and the fraction: Combine the whole number (1) and the fraction (1/12) to form the mixed number.
Therefore, the mixed number for 13/12 is 1 1/12.
Alternative Methods for Conversion
While the long division method is straightforward, other approaches can be equally effective, particularly for mental calculations or when working with simpler fractions.
Method 2: Visual Representation
Imagine you have 13 equal-sized slices of pizza. Since each pizza has 12 slices, you can make one whole pizza (12 slices) and have 1 slice remaining. This visually represents 1 1/12 pizzas.
Method 3: Understanding the Concept of "Units"
Think of the denominator (12) as representing units or groups. How many groups of 12 can you make from 13? You can make one complete group (12 units) with 1 unit left over. This leaves you with 1 whole and 1/12 remaining, confirming the mixed number 1 1/12.
Practical Applications of Mixed Numbers
Mixed numbers are widely used in everyday life and various fields:
-
Cooking and Baking: Recipes often call for mixed numbers of cups, teaspoons, or tablespoons. For example, a recipe might require 1 1/2 cups of flour.
-
Measurement: Many measurement systems utilize mixed numbers. You might measure the length of a piece of wood as 2 3/4 feet.
-
Construction and Engineering: Precise measurements in construction and engineering frequently involve mixed numbers to represent dimensions and quantities.
-
Data Analysis: In data analysis and statistics, mixed numbers can arise when presenting average values or summarizing data.
-
Time: Expressing time often involves mixed numbers. For example, 1 hour and 30 minutes can be written as 1 1/2 hours.
Further Exploration of Fractions and Mixed Numbers
Understanding the conversion between improper fractions and mixed numbers is just one aspect of working with fractions. Here are some related topics to further enhance your understanding:
-
Adding and Subtracting Fractions and Mixed Numbers: Mastering these operations requires a solid understanding of common denominators and the process of converting between improper fractions and mixed numbers.
-
Multiplying and Dividing Fractions and Mixed Numbers: These operations involve different techniques than addition and subtraction, requiring careful attention to the rules of fraction multiplication and division.
-
Simplifying Fractions: Reducing fractions to their simplest form is crucial for efficient calculations and clear presentation of results.
-
Comparing Fractions: Determining which fraction is greater or smaller is a fundamental skill in many mathematical applications.
-
Fractions and Decimals: Understanding the relationship between fractions and decimals is important for converting between these representations and solving various mathematical problems.
Real-World Examples Illustrating Mixed Numbers
Let's look at a few more examples to illustrate the practical application of mixed numbers:
Example 1: Sharing Pizza
If you have 17 slices of pizza and each pizza has 8 slices, how many pizzas do you have?
17 ÷ 8 = 2 with a remainder of 1. Therefore, you have 2 1/8 pizzas.
Example 2: Measuring Fabric
You need to cut 25 inches of fabric from a roll. If you need lengths of 12 inches, how many 12-inch pieces can you cut, and what is the leftover amount?
25 ÷ 12 = 2 with a remainder of 1. You can cut 2 pieces, with 1 inch remaining.
Example 3: Calculating Time
You worked for 150 minutes. How many hours and minutes did you work?
Since there are 60 minutes in an hour, 150 ÷ 60 = 2 with a remainder of 30. You worked 2 hours and 30 minutes, or 2 1/2 hours.
Conclusion: Mastering Fractions for Enhanced Mathematical Skills
This guide provided a comprehensive exploration of how to convert the improper fraction 13/12 into its mixed number equivalent, 1 1/12. We explored various methods for the conversion, demonstrating its practicality through real-world examples. By understanding the fundamentals of fractions and mixed numbers, you equip yourself with essential mathematical skills applicable across diverse fields. Continuing to explore related concepts, such as adding, subtracting, multiplying, and dividing fractions and mixed numbers, will further enhance your mathematical proficiency and problem-solving abilities. Remember, consistent practice is key to mastering these important mathematical concepts.
Latest Posts
Latest Posts
-
Find The Point On The Y Axis Which Is Equidistant From
May 09, 2025
-
Is 3 4 Bigger Than 7 8
May 09, 2025
-
Which Of These Is Not A Prime Number
May 09, 2025
-
What Is 30 Percent Off Of 80 Dollars
May 09, 2025
-
Are Alternate Exterior Angles Always Congruent
May 09, 2025
Related Post
Thank you for visiting our website which covers about What Is The Mixed Number For 13/12 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.