What Is The Percentage Of 5/3
News Co
May 08, 2025 · 5 min read
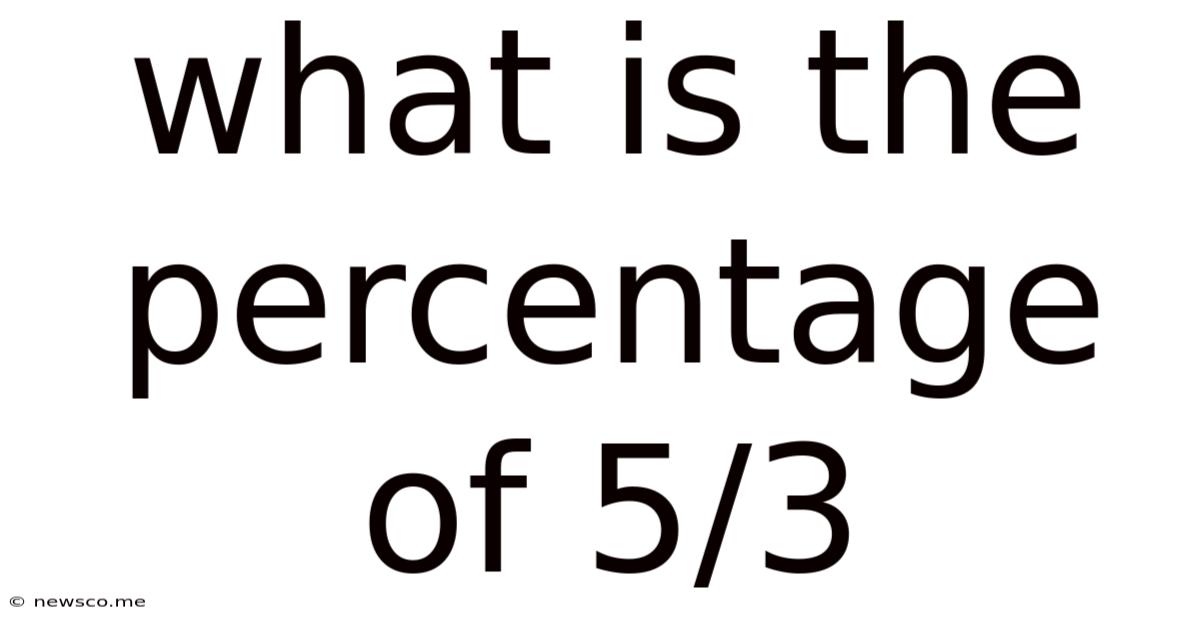
Table of Contents
What is the Percentage of 5/3? Understanding Fractions and Percentages
Understanding fractions and their percentage equivalents is a fundamental skill in mathematics with wide-ranging applications in everyday life, from calculating discounts and tips to analyzing data and understanding financial reports. This article delves deep into the conversion of the fraction 5/3 into a percentage, explaining the process step-by-step and exploring related concepts to build a solid understanding of this crucial mathematical concept.
From Fraction to Decimal: The First Step
Before we can express 5/3 as a percentage, we first need to convert it into a decimal. A fraction represents a part of a whole, where the numerator (top number) indicates the number of parts, and the denominator (bottom number) indicates the total number of parts that make up the whole. In the fraction 5/3, the numerator is 5 and the denominator is 3. This means we have 5 parts out of a total of 3 parts. Since the numerator is larger than the denominator, this fraction is what we call an improper fraction. It represents a value greater than 1.
To convert 5/3 into a decimal, we perform a simple division: we divide the numerator (5) by the denominator (3).
5 ÷ 3 = 1.666...
The result is a decimal number, 1.666..., where the '6' repeats infinitely. This is often represented as 1.6̅6̅ or 1.6 recurring. This recurring decimal indicates that the fraction 5/3 represents a value slightly larger than 1 and 2/3.
Converting Decimal to Percentage: The Crucial Step
Now that we have the decimal equivalent of 5/3 (1.666...), we can easily convert it to a percentage. A percentage is simply a fraction expressed as parts per hundred. To convert a decimal to a percentage, we multiply the decimal by 100 and add the percent symbol (%).
1.666... x 100 = 166.666...%
Therefore, 5/3 is equal to 166.666...%. The recurring decimal means that this percentage is an approximation. In practical applications, we often round this to a specific number of decimal places depending on the required level of accuracy. Commonly used approximations include:
- 166.67%: Rounded to two decimal places, this is a widely accepted approximation.
- 166.7%: Rounded to one decimal place.
- 167%: Rounded to the nearest whole number.
The choice of approximation depends on the context. For instance, in financial calculations, higher accuracy might be necessary, whereas in less precise contexts, rounding to a whole number might suffice.
Understanding the Significance of 5/3 and its Percentage Equivalent
The fraction 5/3 and its percentage equivalent, approximately 166.67%, have practical applications in numerous situations. Consider the following examples:
- Increase in Value: If a quantity increases by 5/3, it means it has increased by approximately 166.67% of its original value. For example, if an investment grows from $100 to $166.67, then it has increased by 5/3, or 166.67%.
- Scaling and Ratios: The ratio 5:3 can be expressed as 5/3. This ratio might represent various scenarios such as the proportion of ingredients in a recipe, the ratio of male to female employees in a company, or even the ratio of wins to losses in a sports game. Understanding its percentage equivalent helps to visualize the relative proportions more clearly.
- Data Analysis: In statistical analyses, the ratio 5/3 might appear in comparing different datasets or groups. Representing this ratio as a percentage provides a standardized way of comparing and interpreting the results.
Practical Applications and Real-World Scenarios
Let's illustrate the practical application of converting 5/3 to a percentage with some real-world scenarios:
Scenario 1: Business Growth
A company's revenue increased from $3 million to $5 million in a year. To calculate the percentage increase:
- Find the difference: $5 million - $3 million = $2 million
- Divide the difference by the original amount: $2 million / $3 million = 0.666...
- Multiply by 100 to convert to percentage: 0.666... x 100 = 66.67%
This shows a revenue increase of approximately 66.67%, not 166.67%. It's crucial to understand that this is different from the direct conversion of 5/3 to a percentage. The 166.67% represents the final amount as a percentage of the original amount, including the original amount itself.
Scenario 2: Recipe Scaling
A recipe calls for 3 cups of flour and 5 cups of sugar. The ratio of sugar to flour is 5/3, approximately 166.67%. This indicates that there's significantly more sugar than flour in the recipe. If you want to halve the recipe, you would divide both ingredients by 2. The ratio would remain 5/3 or approximately 166.67%.
Scenario 3: Data Interpretation
In a survey, 5 out of 3 participants preferred a particular brand. While this is not a realistic scenario, it serves to illustrate the concept. This is expressed as the fraction 5/3, meaning the preference was significantly greater than the number of participants. Converting this to a percentage (approximately 166.67%) helps visualize the overwhelming preference for that brand.
Beyond the Basics: Expanding Your Understanding
Understanding the conversion of fractions to percentages goes beyond simple arithmetic. It's a stepping stone to more complex mathematical concepts like:
- Ratios and Proportions: The ability to convert fractions to percentages enhances understanding and manipulation of ratios and proportions.
- Statistical Analysis: Percentages are crucial for representing and interpreting data in statistical analyses.
- Financial Calculations: Percentages are indispensable for calculating interest rates, discounts, profits, and losses.
- Algebra and Equation Solving: Understanding percentages facilitates solving algebraic equations involving fractions and proportions.
Conclusion: Mastering the Conversion
The conversion of 5/3 to a percentage, resulting in approximately 166.67%, is more than just a mathematical calculation. It represents a crucial skill that finds application in diverse fields. By understanding the underlying concepts of fractions, decimals, and percentages, and by practicing conversions, you equip yourself with a powerful tool for analyzing data, solving problems, and making informed decisions in various aspects of life. Remember to always consider the context when choosing the level of precision for your approximation. The ability to move fluidly between these different representations of numbers is an invaluable asset in mathematics and beyond.
Latest Posts
Related Post
Thank you for visiting our website which covers about What Is The Percentage Of 5/3 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.