What Is The Slope Of A Line That Is Perpendicular
News Co
Apr 06, 2025 · 7 min read
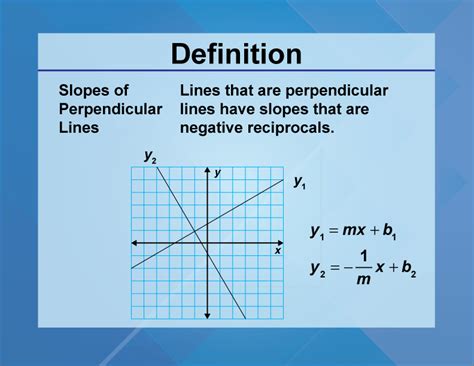
Table of Contents
What is the Slope of a Line That is Perpendicular?
Understanding the relationship between the slopes of perpendicular lines is fundamental in geometry and various applications, including calculus, physics, and computer graphics. This comprehensive guide delves into the concept of perpendicular lines and their slopes, providing a thorough explanation with illustrative examples and practical applications.
Understanding Slopes and Lines
Before we dive into perpendicular lines, let's refresh our understanding of the slope of a line. The slope (often represented by 'm') measures the steepness or inclination of a line. It's defined as the ratio of the vertical change (rise) to the horizontal change (run) between any two distinct points on the line. Mathematically, given two points (x₁, y₁) and (x₂, y₂), the slope is calculated as:
m = (y₂ - y₁) / (x₂ - x₁)
A positive slope indicates a line that rises from left to right, a negative slope indicates a line that falls from left to right, a slope of zero represents a horizontal line, and an undefined slope signifies a vertical line.
Defining Perpendicular Lines
Two lines are considered perpendicular if they intersect at a right angle (90 degrees). This seemingly simple definition holds profound implications for their slopes. Visualize two lines intersecting at a right angle – one line might be steep, while the other is relatively flat. This visual intuition hints at an inverse relationship between their slopes.
The Relationship Between Slopes of Perpendicular Lines
The key relationship between the slopes of two perpendicular lines is that they are negative reciprocals of each other. This means that if one line has a slope 'm', the slope of a line perpendicular to it will be '-1/m'.
Let's break this down:
- Negative: The sign is flipped. If 'm' is positive, '-1/m' will be negative, and vice versa.
- Reciprocal: The fraction is inverted. The numerator becomes the denominator, and the denominator becomes the numerator.
Example 1:
If a line has a slope of 2 (m = 2), the slope of a line perpendicular to it will be -1/2.
Example 2:
If a line has a slope of -3/4 (m = -3/4), the slope of a line perpendicular to it will be 4/3. Notice that the negative sign is removed because the reciprocal of a negative number is negative.
Example 3:
If a line has a slope of 0 (a horizontal line), then a line perpendicular to it will have an undefined slope (a vertical line). This is because the reciprocal of 0 is undefined.
Example 4:
If a line has an undefined slope (a vertical line), then a line perpendicular to it will have a slope of 0 (a horizontal line).
Proof of the Negative Reciprocal Relationship
We can formally prove the negative reciprocal relationship using the concept of right angles and the Pythagorean theorem. Consider two lines intersecting at a point (x, y). Let's denote the slopes of these lines as m₁ and m₂.
Suppose the lines intersect at a right angle. We can construct a right-angled triangle using points on both lines. The Pythagorean theorem states that in a right-angled triangle with legs of length 'a' and 'b', and hypotenuse of length 'c', a² + b² = c².
By applying this theorem to the right-angled triangle formed by the intersection of the perpendicular lines, and using the slope formula to express the lengths of the legs, we can derive the condition m₁ * m₂ = -1, which implies that m₂ = -1/m₁. This confirms the negative reciprocal relationship between the slopes. A detailed geometrical proof requires coordinate geometry and is beyond the scope of a concise blog post but can easily be found in advanced geometry textbooks.
Applications of Perpendicular Lines and Slopes
The concept of perpendicular lines and their slopes has far-reaching applications in numerous fields:
1. Geometry and Coordinate Geometry:
- Finding perpendicular bisectors: The perpendicular bisector of a line segment is a line that passes through the midpoint of the segment and is perpendicular to it. Knowing the slope of the segment allows us to determine the slope of its perpendicular bisector, enabling us to find its equation.
- Determining angles between lines: The angle between two lines can be calculated using their slopes. If the lines are perpendicular, the angle is 90 degrees, simplifying calculations.
- Constructing geometric figures: Perpendicular lines are fundamental to constructing various geometric figures such as squares, rectangles, and right-angled triangles.
2. Calculus:
- Finding tangents and normals: In calculus, the tangent to a curve at a point is a line that touches the curve at that point and has the same instantaneous slope. The normal line to the curve at that same point is perpendicular to the tangent. Therefore, knowing the slope of the tangent allows us to find the slope of the normal, crucial for solving optimization problems and other applications.
- Gradient descent algorithms in machine learning: Gradient descent uses the negative gradient of a function to iteratively find a local minimum. The gradient, which is a vector, is often visualized as a steepest descent line. Movement against the gradient toward the minimum is conceptually similar to moving perpendicular to the level curves.
3. Physics:
- Vectors and forces: In physics, vectors representing forces or velocities are often resolved into components that are parallel and perpendicular to a specific direction. Understanding perpendicular lines helps to analyze these components effectively.
- Optics: Reflection and refraction of light involve angles of incidence and reflection. The laws of reflection rely heavily on perpendicular lines.
4. Computer Graphics:
- Line intersections and collision detection: In computer graphics, detecting collisions between objects often involves checking for intersections between lines. Determining perpendicularity can accelerate these checks and optimize algorithms.
- Generating orthogonal grids: Creating grids in game development or CAD software often involves generating perpendicular lines to form a well-defined coordinate system.
- Transformations: Rotating objects involves understanding the relationships between the axes and rotation angles. Perpendicularity plays a crucial role here.
Solving Problems Related to Perpendicular Lines
Let's work through a few problems to solidify our understanding:
Problem 1:
Find the slope of a line perpendicular to the line passing through points A(2, 5) and B(4, 1).
Solution:
First, find the slope of line AB:
m_AB = (1 - 5) / (4 - 2) = -4 / 2 = -2
The slope of a line perpendicular to AB is the negative reciprocal of -2, which is 1/2.
Problem 2:
Line L₁ has a slope of 3/5. Line L₂ is perpendicular to L₁ and passes through the point (1, 2). Find the equation of Line L₂.
Solution:
The slope of L₂ is the negative reciprocal of 3/5, which is -5/3. Using the point-slope form of a line's equation (y - y₁ = m(x - x₁)), we have:
y - 2 = (-5/3)(x - 1)
Simplifying, we get:
y = (-5/3)x + (5/3) + 2
y = (-5/3)x + 11/3
Problem 3:
Determine if the lines y = 2x + 1 and y = -1/2x + 3 are perpendicular.
Solution:
The slope of the first line is 2. The slope of the second line is -1/2. Since these slopes are negative reciprocals of each other (2 * (-1/2) = -1), the lines are perpendicular.
Conclusion
The relationship between the slopes of perpendicular lines, being negative reciprocals, is a fundamental concept with vast applications across various disciplines. A solid understanding of this relationship is essential for solving geometric problems, working with derivatives in calculus, analyzing physical systems, and developing efficient algorithms in computer graphics and other fields. By mastering this core concept, you gain the ability to effectively model, analyze, and solve problems in a wide range of contexts. This knowledge provides a strong foundation for further exploration in advanced mathematics and related subjects.
Latest Posts
Latest Posts
-
What Is 3 5 Equivalent To In Fractions
Apr 08, 2025
-
How Can I Find Out Someones Age
Apr 08, 2025
-
What Are Common Multiples Of 3 And 5
Apr 08, 2025
-
What Percent Is 1 Of 6
Apr 08, 2025
-
Lowest Common Multiple Of 45 And 60
Apr 08, 2025
Related Post
Thank you for visiting our website which covers about What Is The Slope Of A Line That Is Perpendicular . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.