What Is The Square Root 4
News Co
Apr 03, 2025 · 5 min read
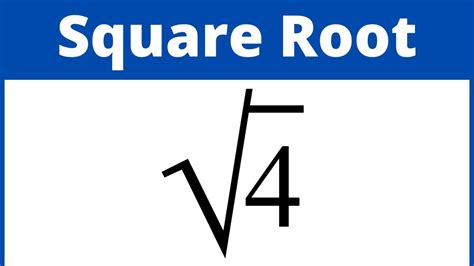
Table of Contents
What is the Square Root of 4? A Deep Dive into Square Roots and their Applications
The seemingly simple question, "What is the square root of 4?" opens the door to a fascinating exploration of fundamental mathematical concepts and their far-reaching applications. While the answer itself is straightforward – 2 – understanding the underlying principles behind square roots provides a solid foundation for more advanced mathematical pursuits. This article will delve deep into the concept of square roots, focusing specifically on the square root of 4, exploring its properties, and demonstrating its relevance in various fields.
Understanding Square Roots: A Foundational Concept
Before we delve into the specifics of the square root of 4, let's establish a firm understanding of what a square root actually is. In simple terms, the square root of a number is a value that, when multiplied by itself (squared), equals the original number. For example, the square root of 9 is 3 because 3 x 3 = 9. This relationship can be expressed mathematically as:
√x = y if and only if y² = x
Here, 'x' represents the number for which we are finding the square root, and 'y' represents the square root itself. The symbol '√' is the radical symbol, denoting the square root operation.
Calculating the Square Root of 4
Now, let's address the core question: What is the square root of 4? Using the definition above, we're looking for a number that, when multiplied by itself, equals 4. The answer is undeniably 2, as 2 x 2 = 4. Therefore:
√4 = 2
However, it's crucial to understand that this is only one of the square roots. The concept of negative numbers adds another layer of complexity. While (-2) x (-2) also equals 4, the principal square root (the one most commonly used) is the positive value. This distinction is particularly important in advanced mathematical contexts.
The Importance of the Principal Square Root
The concept of the principal square root is vital for consistency and avoiding ambiguity in mathematical calculations. Choosing a single principal square root (the positive one) allows us to define a unique function, ensuring that every non-negative number has only one square root. This is essential for the proper functioning of many mathematical formulas and equations. While both 2 and -2 satisfy the equation x² = 4, using the principal square root convention simplifies many calculations and prevents confusion.
Visualizing Square Roots: Geometric Interpretation
The square root of 4 can also be visualized geometrically. Imagine a square with an area of 4 square units. The length of each side of this square is the square root of its area. In this case, the side length is 2 units because 2 x 2 = 4. This geometric interpretation helps to solidify the connection between the concept of square roots and spatial dimensions.
Square Roots in Real-World Applications
The seemingly simple concept of square roots has wide-ranging applications in numerous fields. Some notable examples include:
1. Physics and Engineering
-
Calculating distances: The Pythagorean theorem, a cornerstone of geometry and physics, relies heavily on square roots. It states that in a right-angled triangle, the square of the hypotenuse (the longest side) is equal to the sum of the squares of the other two sides. Finding the length of the hypotenuse requires calculating the square root of the sum of the squares of the other two sides. This is fundamental to calculating distances and analyzing vectors in physics and engineering.
-
Wave propagation: Understanding wave behavior involves dealing with various forms of wave equations, often requiring the calculation of square roots to find parameters like wavelengths and frequencies.
-
Electrical engineering: Calculations involving impedance and reactance in electrical circuits frequently utilize square roots.
2. Computer Science
-
Graphics programming: Square roots are essential in 3D graphics and game development for calculating distances between points, normalizing vectors, and handling transformations.
-
Algorithms and data structures: Certain algorithms and data structures, such as the shortest path algorithms, rely on square root computations.
3. Statistics and Data Analysis
- Standard deviation: Calculating the standard deviation of a dataset, a crucial measure of data dispersion, involves finding the square root of the variance. This helps determine the spread and variability of the data.
4. Finance and Economics
- Financial modeling: Square roots are incorporated into various financial models, for example, in option pricing models, to estimate volatility and risk.
Advanced Concepts Related to Square Roots
Understanding the square root of 4 provides a stepping stone towards grasping more complex mathematical concepts, including:
-
Higher-order roots: Square roots are just one type of root. Cube roots (the number that, when cubed, equals the original number), fourth roots, and so on, are all extensions of the same fundamental idea.
-
Complex numbers: The concept of square roots extends into the realm of complex numbers, where the square root of a negative number can be defined using imaginary units.
-
Irrational numbers: While the square root of 4 is a rational number (2), many square roots are irrational, meaning they cannot be expressed as a simple fraction. For example, the square root of 2 is an irrational number.
Conclusion: The Significance of the Simple Square Root
The square root of 4, while appearing elementary, holds profound significance within the larger landscape of mathematics. Its simple calculation unveils the fundamental nature of square roots, showcasing their importance across diverse disciplines. From geometric visualizations to complex equations, this fundamental mathematical concept serves as a cornerstone for further exploration and understanding of more advanced mathematical concepts and their application to real-world problems. The ability to grasp this seemingly basic calculation serves as a crucial foundation for any aspiring mathematician, physicist, engineer, or computer scientist. It is a testament to the power of simple ideas in unlocking a deeper understanding of complex systems.
Latest Posts
Related Post
Thank you for visiting our website which covers about What Is The Square Root 4 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.