What Is The Sum Of Interior Angles In A Hexagon
News Co
Apr 05, 2025 · 5 min read
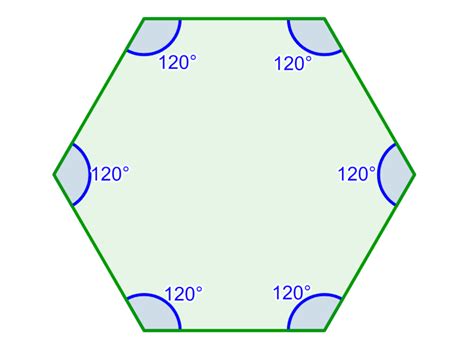
Table of Contents
What is the Sum of Interior Angles in a Hexagon? A Comprehensive Guide
The sum of interior angles in a hexagon, a six-sided polygon, is a fundamental concept in geometry. Understanding this concept is crucial for solving various geometric problems and grasping more advanced geometrical principles. This comprehensive guide will delve into the calculation of the sum of interior angles in a hexagon, explore related concepts, and provide practical examples to solidify your understanding.
Understanding Polygons and Interior Angles
Before we jump into hexagons, let's establish a foundational understanding of polygons and their interior angles. A polygon is a closed two-dimensional figure formed by connecting three or more straight line segments. These segments are called the sides of the polygon, and the points where the sides meet are called vertices.
The interior angles of a polygon are the angles formed inside the polygon at each vertex. The sum of these interior angles varies depending on the number of sides the polygon has.
Calculating the Sum of Interior Angles: The General Formula
There's a straightforward formula to calculate the sum of interior angles for any polygon, regardless of the number of sides. This formula is:
(n - 2) * 180°
Where 'n' represents the number of sides of the polygon.
This formula works because any polygon can be divided into triangles by drawing diagonals from one vertex to all other non-adjacent vertices. The number of triangles formed is always two less than the number of sides (n-2). Since the sum of angles in a triangle is always 180°, multiplying this by the number of triangles gives us the total sum of interior angles.
Applying the Formula to a Hexagon
A hexagon is a polygon with six sides (n=6). Applying the general formula, we can easily calculate the sum of its interior angles:
(6 - 2) * 180° = 4 * 180° = 720°
Therefore, the sum of the interior angles in any hexagon is 720°. This holds true for all hexagons, regardless of their shape or size – regular or irregular.
Regular vs. Irregular Hexagons
It's important to differentiate between regular and irregular hexagons:
-
Regular Hexagon: A regular hexagon has all six sides of equal length and all six interior angles of equal measure. In a regular hexagon, each interior angle measures 120° (720° / 6 = 120°).
-
Irregular Hexagon: An irregular hexagon has sides of varying lengths and angles of varying measures. While the sum of interior angles remains 720°, the individual angles will not all be equal.
Practical Applications and Examples
The knowledge of the sum of interior angles in a hexagon is crucial in various applications, including:
-
Architecture and Engineering: Designing structures with hexagonal elements, like honeycomb structures or certain types of tiling, requires understanding the angular relationships.
-
Computer Graphics and Game Development: Creating hexagonal grids or modeling hexagonal objects in 3D modeling software necessitates accurate calculations of interior angles.
-
Tessellations and Geometry Puzzles: Many geometric puzzles and tessellations utilize hexagonal shapes. Knowing the angle sums helps solve these puzzles and understand the patterns formed.
-
Cartography: Hexagonal grids are sometimes used in cartography for data representation, and understanding the angular properties is crucial for accurate mapping.
Let's consider some examples:
Example 1: Finding a Missing Angle
Suppose we have an irregular hexagon with five known angles: 110°, 125°, 130°, 140°, and 155°. To find the missing angle, we use the known sum of interior angles:
720° - (110° + 125° + 130° + 140° + 155°) = 720° - 660° = 60°
The missing angle is 60°.
Example 2: Regular Hexagon's Exterior Angle
In a regular hexagon, each interior angle measures 120°. The exterior angle at each vertex is supplementary to the interior angle (they add up to 180°). Therefore, each exterior angle in a regular hexagon is:
180° - 120° = 60°
The sum of exterior angles in any polygon, including a hexagon, is always 360°.
Beyond Hexagons: Extending the Knowledge
Understanding the sum of interior angles in a hexagon provides a stepping stone to understanding similar concepts for polygons with different numbers of sides. By using the general formula (n - 2) * 180°, you can calculate the sum of interior angles for any polygon, be it a pentagon, heptagon, octagon, or any other n-sided polygon.
Advanced Concepts and Related Topics
This understanding lays the groundwork for exploring more advanced geometrical concepts, such as:
-
Trigonometry: The interior angles of hexagons are often used in trigonometric calculations involving distances and angles within the hexagon.
-
Vector Geometry: Hexagons can be represented and manipulated using vectors, and understanding the angle sums is essential for calculations involving vector operations.
-
Solid Geometry: Three-dimensional shapes that incorporate hexagonal faces, like hexagonal prisms or pyramids, require knowledge of planar geometry, including the sum of interior angles of hexagons.
Conclusion: Mastering Hexagonal Geometry
The sum of interior angles in a hexagon, always 720°, is a fundamental geometric concept with diverse applications. Understanding this principle, along with the general formula for calculating interior angle sums in any polygon, provides a strong foundation for tackling more complex geometric problems and advancing your understanding of mathematical principles. By mastering these concepts, you can confidently tackle challenges in various fields, from architectural design to computer graphics and beyond. Remember the formula – (n-2) * 180° – and you'll be well-equipped to solve any problem involving the interior angles of polygons!
Latest Posts
Related Post
Thank you for visiting our website which covers about What Is The Sum Of Interior Angles In A Hexagon . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.