What Percent Is 2 Of 7
News Co
Mar 20, 2025 · 4 min read
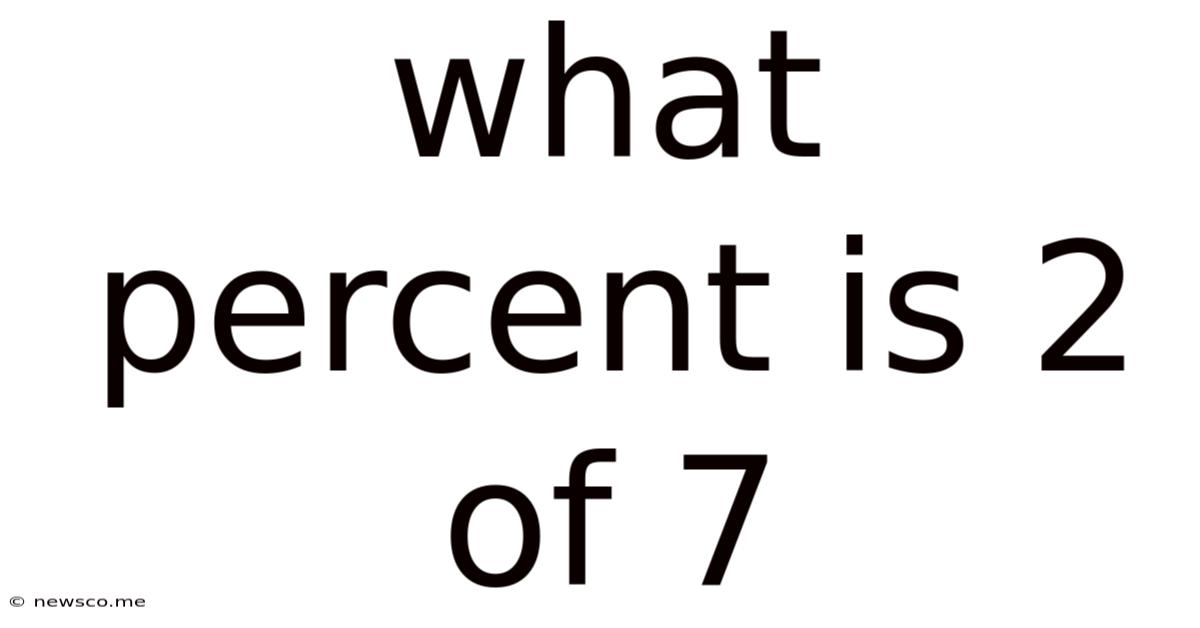
Table of Contents
What Percent is 2 of 7? A Deep Dive into Percentage Calculations
This seemingly simple question – "What percent is 2 of 7?" – opens the door to a broader understanding of percentages, their applications, and the various methods used to calculate them. While the answer itself is straightforward, exploring the underlying concepts and alternative approaches offers valuable insights for anyone seeking to improve their mathematical skills and problem-solving abilities.
Understanding Percentages: The Foundation
Before diving into the calculation, let's solidify our understanding of percentages. A percentage is simply a fraction expressed as a portion of 100. The symbol "%" represents "per hundred" or "out of 100." Therefore, 50% means 50 out of 100, which is equivalent to the fraction 50/100 or the decimal 0.5.
Percentages are used extensively in everyday life, from calculating discounts and sales tax to understanding financial reports and expressing statistical data. Mastering percentage calculations is a crucial skill for navigating various aspects of personal and professional life.
Calculating "What Percent is 2 of 7?" – The Direct Approach
The most direct method involves converting the fraction 2/7 into a percentage. This is achieved through the following steps:
-
Express the relationship as a fraction: The problem states "2 of 7," which translates directly into the fraction 2/7.
-
Convert the fraction to a decimal: Divide the numerator (2) by the denominator (7): 2 ÷ 7 ≈ 0.2857
-
Convert the decimal to a percentage: Multiply the decimal by 100: 0.2857 × 100 ≈ 28.57%
Therefore, 2 is approximately 28.57% of 7.
Alternative Methods: Exploring Different Perspectives
While the direct approach is efficient, exploring alternative methods reinforces understanding and provides flexibility in solving similar problems.
Method 2: Using Proportions
Proportions offer a powerful way to solve percentage problems. We can set up a proportion to find the percentage:
- x/100 = 2/7
Where 'x' represents the percentage we're trying to find. To solve for x:
-
Cross-multiply: 7x = 200
-
Solve for x: x = 200/7 ≈ 28.57
This confirms that 2 is approximately 28.57% of 7.
Method 3: Understanding the Concept of "Part" and "Whole"
In percentage problems, it's crucial to identify the "part" and the "whole." In this case:
- Part: 2
- Whole: 7
The percentage represents the ratio of the part to the whole, multiplied by 100. Therefore:
(Part / Whole) * 100% = (2/7) * 100% ≈ 28.57%
Practical Applications: Real-World Examples
Understanding percentage calculations extends far beyond simple mathematical exercises. Let's consider some real-world applications:
1. Sales and Discounts:
Imagine a store offering a discount. If an item originally costs $7 and is discounted by $2, the discount percentage can be calculated using the same method: ($2/$7) * 100% ≈ 28.57%.
2. Financial Analysis:
In financial analysis, percentages are critical for interpreting financial statements. For instance, calculating the profit margin involves determining the percentage of revenue that represents profit.
3. Statistical Analysis:
Percentages are extensively used in statistical data representation. For example, expressing the percentage of respondents who answered "yes" to a survey question requires calculating the ratio of "yes" responses to the total number of respondents, then multiplying by 100%.
4. Everyday Life Scenarios:
Numerous everyday scenarios involve percentages. Calculating tips in restaurants, determining the percentage of a task completed, or understanding interest rates on loans all rely on a solid grasp of percentage calculations.
Expanding the Understanding: Dealing with More Complex Scenarios
While "What percent is 2 of 7?" presents a relatively simple problem, the principles extend to more complex scenarios. Consider these examples:
1. Percentages with Decimals:
What percent is 2.5 of 7? The same methods apply: (2.5/7) * 100% ≈ 35.71%
2. Finding the Whole when the Percentage and Part are Known:
If 28.57% of a number is 2, what is the number? This requires reversing the percentage calculation. Let 'x' represent the whole:
(28.57/100) * x = 2
Solving for x gives you x ≈ 7
3. Percentage Change:
Calculating percentage change (increase or decrease) involves determining the difference between two values, dividing by the original value, and then multiplying by 100%. For instance, if a quantity increases from 5 to 7, the percentage increase is ((7-5)/5) * 100% = 40%.
Mastering Percentages: Tips and Tricks
- Practice Regularly: The key to mastering any mathematical concept is consistent practice. Solve various percentage problems, starting with simple ones and gradually increasing the complexity.
- Visual Aids: Diagrams, charts, and graphs can help visualize the relationship between the part and the whole, making the concept easier to grasp.
- Real-World Applications: Apply your knowledge to real-life situations to make the learning process more engaging and memorable.
- Use Online Resources: Numerous online resources, including calculators and tutorials, can provide additional support and practice exercises.
Conclusion: Beyond the Calculation
The question "What percent is 2 of 7?" serves as a springboard to a much deeper understanding of percentages and their widespread applications. Mastering percentage calculations is a valuable skill that empowers individuals to confidently navigate numerous personal, professional, and academic challenges. By understanding the underlying principles and applying various calculation methods, you can effectively tackle diverse percentage problems and interpret data with increased accuracy and confidence. Remember, consistent practice and a focus on applying the concept to real-world situations are key to solidifying your understanding.
Latest Posts
Latest Posts
-
Find The Point On The Y Axis Which Is Equidistant From
May 09, 2025
-
Is 3 4 Bigger Than 7 8
May 09, 2025
-
Which Of These Is Not A Prime Number
May 09, 2025
-
What Is 30 Percent Off Of 80 Dollars
May 09, 2025
-
Are Alternate Exterior Angles Always Congruent
May 09, 2025
Related Post
Thank you for visiting our website which covers about What Percent Is 2 Of 7 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.