What Percentage Is 20 Of 80
News Co
May 08, 2025 · 4 min read
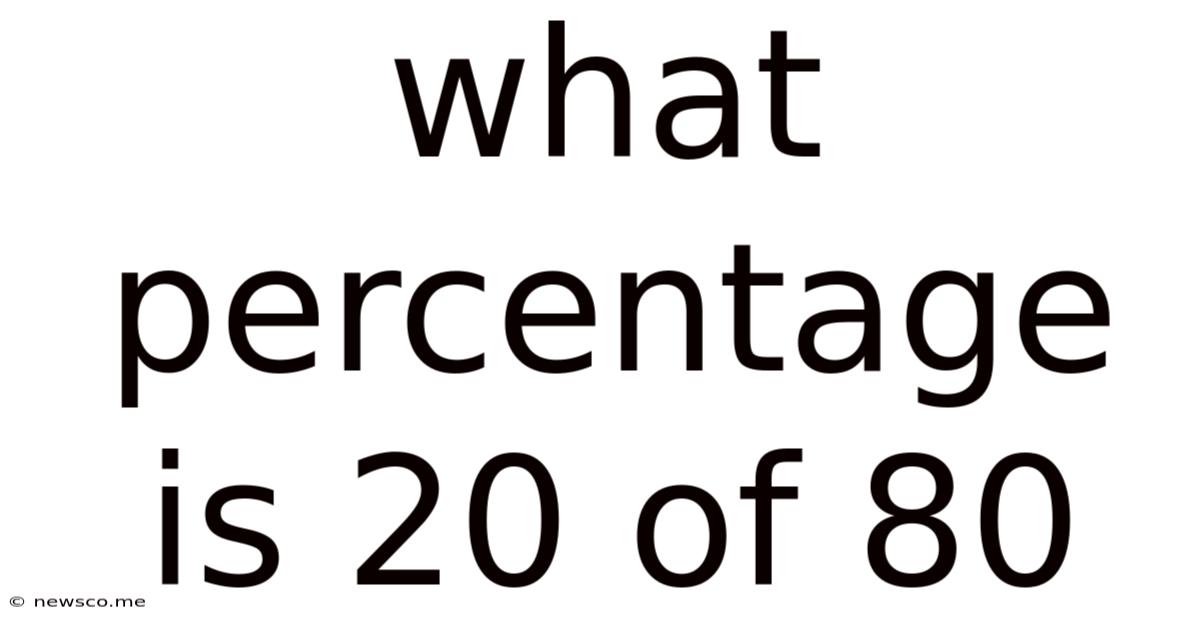
Table of Contents
What Percentage is 20 of 80? A Comprehensive Guide to Percentage Calculations
Determining what percentage 20 represents of 80 is a fundamental concept in mathematics with broad applications in various fields. This seemingly simple calculation forms the basis of understanding proportions, ratios, and more complex percentage-based problems. This comprehensive guide will not only answer the question directly but also delve into the underlying principles, providing you with the tools to tackle similar percentage calculations with ease.
Understanding Percentages
Before jumping into the calculation, let's solidify our understanding of percentages. A percentage is a fraction or ratio expressed as a number out of 100. The symbol "%" represents "per hundred" or "out of 100." For example, 50% means 50 out of 100, which is equivalent to ½ or 0.5 as a decimal.
Calculating the Percentage: 20 out of 80
To determine what percentage 20 is of 80, we can employ a straightforward method:
-
Set up a fraction: Represent the problem as a fraction. The part (20) is the numerator, and the whole (80) is the denominator: 20/80
-
Simplify the fraction (optional): Simplifying the fraction often makes the calculation easier. In this case, we can divide both the numerator and the denominator by 20: 20/80 = 1/4
-
Convert the fraction to a decimal: Divide the numerator by the denominator: 1 ÷ 4 = 0.25
-
Convert the decimal to a percentage: Multiply the decimal by 100 and add the percentage symbol (%): 0.25 x 100 = 25%
Therefore, 20 is 25% of 80.
Alternative Calculation Methods
While the above method is straightforward, there are other approaches to calculate percentages, especially useful for more complex problems.
Method 2: Using Proportions
We can set up a proportion to solve this:
- Let 'x' represent the unknown percentage.
- We can write the proportion as: x/100 = 20/80
To solve for 'x', we cross-multiply:
- 80x = 2000
- x = 2000/80
- x = 25
Therefore, x = 25%, confirming our previous result.
Method 3: Using the Percentage Formula
The general percentage formula is:
(Part / Whole) x 100 = Percentage
Substituting our values:
(20 / 80) x 100 = 25%
This formula provides a concise and readily applicable method for calculating percentages in various scenarios.
Real-World Applications of Percentage Calculations
Understanding percentage calculations is crucial in numerous real-world situations, including:
-
Finance: Calculating interest rates, discounts, tax amounts, profit margins, and investment returns all rely heavily on percentage calculations. For example, if a bank offers a 5% interest rate on a savings account, understanding percentages helps you determine the interest earned on your deposit.
-
Sales and Marketing: Determining sales growth, conversion rates, market share, and customer acquisition costs frequently involves percentage analysis. Tracking these metrics helps businesses understand their performance and make informed decisions. A 10% increase in sales compared to the previous year is a significant indicator of business growth.
-
Science and Statistics: Percentages are essential in representing data, expressing probabilities, and analyzing experimental results. For instance, a survey might report that 75% of respondents prefer a certain product.
-
Education: Grading systems often rely on percentages to express student performance. A score of 85% on an exam typically indicates a good understanding of the subject matter.
-
Everyday Life: Calculating tips in restaurants, discounts on sales, or understanding nutritional information on food labels all involve percentage calculations.
Advanced Percentage Problems and Solving Techniques
While the problem of "What percentage is 20 of 80?" is relatively simple, understanding the underlying principles allows you to tackle more complex percentage-related challenges.
Example 1: Finding the Whole when given the Percentage and Part
Let's say you received a 15% discount on a purchase, and the discount amount was $30. How much was the original price?
We can use the formula: (Part / Percentage) x 100 = Whole
Substituting: (30 / 15) x 100 = $200
The original price was $200.
Example 2: Finding the Percentage Increase or Decrease
Suppose your salary increased from $50,000 to $55,000. What is the percentage increase?
- Find the difference: $55,000 - $50,000 = $5,000
- Divide the difference by the original amount: $5,000 / $50,000 = 0.1
- Multiply by 100 to express as a percentage: 0.1 x 100 = 10%
Your salary increased by 10%.
Mastering Percentage Calculations: Tips and Tricks
-
Practice Regularly: The more you practice percentage calculations, the more comfortable and efficient you will become.
-
Understand the Fundamentals: A solid understanding of fractions, decimals, and ratios is essential for mastering percentage calculations.
-
Use Multiple Methods: Experiment with different calculation methods to find the one that best suits your problem-solving style and the complexity of the problem.
-
Check Your Work: Always double-check your calculations to ensure accuracy.
Conclusion
Determining that 20 is 25% of 80 is a fundamental yet versatile calculation. Understanding this and the broader principles of percentage calculations is essential for navigating various aspects of daily life, professional endeavors, and academic pursuits. By mastering these techniques and applying them consistently, you can confidently tackle a wide range of percentage-related problems and gain a deeper understanding of this critical mathematical concept. The ability to quickly and accurately calculate percentages is a valuable skill that will serve you well in numerous contexts. Continue to practice, explore different methods, and build your confidence in this crucial area of mathematics.
Latest Posts
Latest Posts
-
3 3 4 Divided By 2 In Fraction
May 08, 2025
-
Shapes That Have 2 Lines Of Symmetry
May 08, 2025
-
Which Graph Represents The Rational Function
May 08, 2025
-
How Many Angles Does A Obtuse Triangle Have
May 08, 2025
-
Cuanto Es 50 Grados En Fahrenheit
May 08, 2025
Related Post
Thank you for visiting our website which covers about What Percentage Is 20 Of 80 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.