What Ratio Is Equivalent To 5/4
News Co
May 08, 2025 · 5 min read
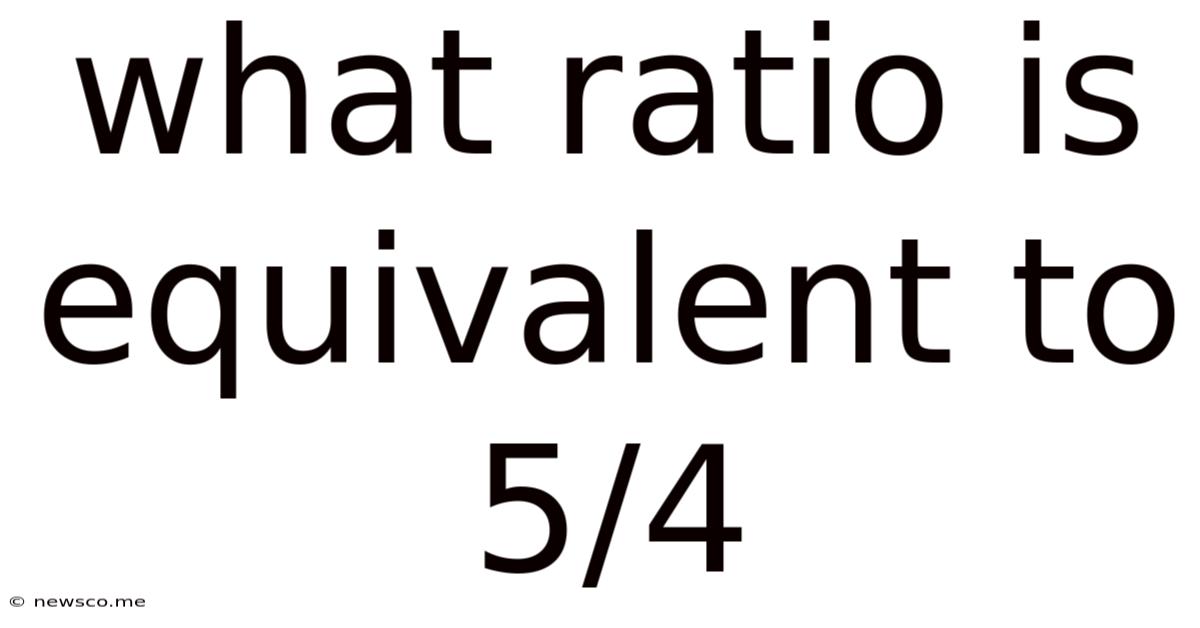
Table of Contents
What Ratio is Equivalent to 5/4? A Deep Dive into Ratio Equivalence
Understanding ratios and their equivalencies is fundamental to various fields, from cooking and construction to advanced mathematics and scientific research. This comprehensive guide explores the concept of ratio equivalence, focusing specifically on the ratio 5/4 and its numerous equivalents. We'll delve into the methods of finding equivalent ratios, practical applications, and how to represent these equivalencies visually and numerically.
Understanding Ratios
A ratio is a comparison of two or more quantities. It shows the relative sizes of the quantities. We can express a ratio in several ways:
- Using the colon symbol: 5:4
- Using the word "to": 5 to 4
- Using a fraction: 5/4
In the ratio 5/4, 5 is the antecedent and 4 is the consequent. This ratio indicates that for every 5 units of one quantity, there are 4 units of another.
Finding Equivalent Ratios: The Fundamental Principle
The key to finding equivalent ratios lies in the concept of proportionality. Two ratios are equivalent if one can be obtained from the other by multiplying or dividing both the antecedent and consequent by the same non-zero number. This is essentially scaling the ratio up or down.
Think of it like this: Imagine a recipe that calls for 5 cups of flour and 4 cups of sugar (a 5:4 ratio). If you want to double the recipe, you'd multiply both quantities by 2, resulting in 10 cups of flour and 8 cups of sugar (a 10:8 ratio). Both ratios, 5:4 and 10:8, are equivalent because they represent the same proportional relationship.
Methods for Finding Equivalent Ratios of 5/4
There are several methods to determine equivalent ratios for 5/4:
1. Multiplication: Scaling Up
The simplest method is to multiply both the numerator (5) and the denominator (4) by the same integer. Let's illustrate with a few examples:
- Multiply by 2: (5 * 2) / (4 * 2) = 10/8
- Multiply by 3: (5 * 3) / (4 * 3) = 15/12
- Multiply by 4: (5 * 4) / (4 * 4) = 20/16
- Multiply by 5: (5 * 5) / (4 * 5) = 25/20
- Multiply by 10: (5 * 10) / (4 * 10) = 50/40
All of these ratios – 10/8, 15/12, 20/16, 25/20, 50/40, and countless others generated by multiplying by any whole number – are equivalent to 5/4.
2. Division: Scaling Down (Simplification)
We can also find equivalent ratios by dividing both the numerator and denominator by a common factor. This process is also known as simplifying or reducing the fraction. While 5/4 is already in its simplest form (since 5 and 4 share no common factors other than 1), we can demonstrate this principle with a slightly different approach. Let's consider a ratio equivalent to 5/4, say 20/16.
- Dividing by 4: (20 / 4) / (16 / 4) = 5/4
This demonstrates that 20/16 simplifies to 5/4, proving their equivalence. This method is crucial for expressing ratios in their simplest form, making them easier to understand and compare.
3. Using Decimal Representation
Another way to understand equivalent ratios is through decimal representation. We can convert the fraction 5/4 into a decimal by performing the division: 5 ÷ 4 = 1.25. Any other ratio that converts to 1.25 is equivalent to 5/4. For example:
- 10/8 = 1.25
- 15/12 = 1.25
- 20/16 = 1.25
This method highlights the constant proportional relationship between the numerator and denominator across all equivalent ratios.
Visual Representation of Equivalent Ratios
Equivalent ratios can be visualized using various methods, including:
-
Bar Models: A bar model can visually represent the ratio 5:4 by dividing a rectangle into 9 equal parts, with 5 parts representing one quantity and 4 parts representing the other. Larger bars representing equivalent ratios (like 10:8 or 15:12) would simply extend the same proportional relationship.
-
Pie Charts: A pie chart can also effectively show the ratio. A circle divided into 9 equal slices, with 5 slices representing one quantity and 4 the other, clearly depicts the 5:4 ratio. Equivalent ratios would use larger circles with proportionately more slices, maintaining the 5:4 ratio across all sections.
Practical Applications of Equivalent Ratios
Understanding equivalent ratios is essential in numerous real-world applications:
-
Cooking: Adjusting recipes to serve more or fewer people requires finding equivalent ratios of ingredients.
-
Construction: Scaling blueprints and plans relies heavily on equivalent ratios to maintain accurate proportions.
-
Mapping: Maps utilize equivalent ratios to represent large distances on a smaller scale. The ratio of the map distance to the actual distance is maintained consistently.
-
Finance: Calculating interest rates, proportions of investments, and profit margins involves working with equivalent ratios.
-
Science: Many scientific concepts, such as proportions in chemical reactions or ratios in physics (like speed, distance, and time), heavily depend on equivalent ratios.
Advanced Concepts and Considerations
While basic multiplication and division are sufficient for finding many equivalent ratios, more complex scenarios might require additional mathematical tools. For instance:
-
Ratio and Proportion Problems: Many word problems involve setting up and solving proportions to find unknown quantities. This often involves cross-multiplication.
-
Proportional Reasoning: This is a higher-order thinking skill involving the ability to reason about proportions and use this reasoning to solve problems.
Conclusion
The ratio 5/4, and its countless equivalents, provides a rich example for understanding ratio equivalence. The methods outlined above – multiplication, division, and decimal representation – offer versatile approaches to identifying and working with equivalent ratios. Recognizing the importance of proportionality and mastering the techniques for finding equivalent ratios is critical for success in various academic and professional fields. By visualizing and understanding these concepts, you can confidently tackle problems involving ratios and proportions, regardless of their complexity. Remember, understanding equivalent ratios is not just about manipulating numbers; it's about grasping the underlying proportional relationships that govern the world around us.
Latest Posts
Latest Posts
-
Which Figures Are Polygons Select Each Correct Answer
May 08, 2025
-
Supplementary Angles Always Add Up To
May 08, 2025
-
What Is 15 Divided By 4
May 08, 2025
-
40 52 Rounded To The Nearest Whole Number
May 08, 2025
-
Find The Slope Given Two Points Worksheet
May 08, 2025
Related Post
Thank you for visiting our website which covers about What Ratio Is Equivalent To 5/4 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.