Area Of Composite Figures Worksheet Pdf
News Co
Apr 03, 2025 · 6 min read
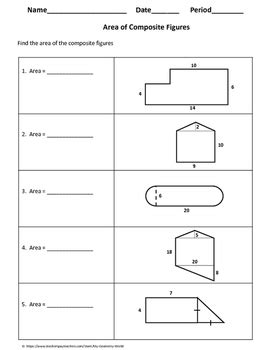
Table of Contents
Area of Composite Figures Worksheet PDF: A Comprehensive Guide
Finding the area of composite figures can be tricky, but it's a fundamental skill in geometry. This comprehensive guide will break down the process, provide practical examples, and offer resources to help you master calculating the area of composite shapes. We'll explore various methods, including using formulas for basic shapes and understanding how to decompose complex figures. This guide is perfect for students, teachers, and anyone looking to improve their geometry skills.
What are Composite Figures?
Composite figures, also known as composite shapes, are shapes formed by combining two or more basic geometric shapes like squares, rectangles, triangles, circles, trapezoids, and parallelograms. They don't have a single, readily available formula for calculating their area. Instead, you need to break them down into simpler shapes whose areas you can calculate.
Understanding the Composition: The key to success lies in recognizing the individual shapes that make up the composite figure. Look closely at the diagram. Can you identify squares, rectangles, triangles, semicircles, or other familiar shapes within the larger figure?
Example: Imagine a figure that looks like a house. It might consist of a rectangle forming the main body and a triangle forming the roof. To find the total area, you'd calculate the area of the rectangle and the area of the triangle separately and then add them together.
Strategies for Calculating Area of Composite Figures
There are several effective strategies for calculating the area of composite figures. Let's explore the most common ones:
1. Decomposition Method
This is the most prevalent approach. It involves breaking down the composite figure into smaller, manageable shapes whose areas you can easily calculate using standard geometric formulas.
Steps:
-
Identify the basic shapes: Carefully examine the composite figure and identify the individual shapes (rectangles, triangles, circles, etc.) that make it up.
-
Calculate individual areas: Use the appropriate formula for each shape to find its area. Remember the formulas:
- Rectangle: Area = length × width
- Square: Area = side × side
- Triangle: Area = (1/2) × base × height
- Circle: Area = π × radius²
- Trapezoid: Area = (1/2) × (base1 + base2) × height
- Parallelogram: Area = base × height
-
Sum the individual areas: Add the areas of all the individual shapes together to obtain the total area of the composite figure.
Example: Consider a figure composed of a rectangle (length = 8 cm, width = 5 cm) and a semicircle with a radius of 2.5 cm attached to one side of the rectangle.
- Area of rectangle: 8 cm × 5 cm = 40 cm²
- Area of semicircle: (1/2) × π × (2.5 cm)² ≈ 9.82 cm²
- Total area: 40 cm² + 9.82 cm² ≈ 49.82 cm²
2. Subtraction Method
Sometimes, it's easier to calculate the area of a larger, encompassing shape and then subtract the area of the smaller shapes that are not part of the composite figure.
Steps:
- Enclose the figure: Draw a larger, simple shape (like a rectangle or square) around the composite figure.
- Calculate the area of the encompassing shape: Find the area of the larger shape you've drawn.
- Calculate the area of the shapes to subtract: Identify the shapes that are outside the composite figure but within the encompassing shape. Calculate their areas.
- Subtract the areas: Subtract the areas of the shapes you identified in step 3 from the area of the encompassing shape to find the area of the composite figure.
Example: Imagine a square with a smaller square cut out of its center. To find the area of the remaining shape (the composite figure), you would:
- Find the area of the larger square.
- Find the area of the smaller, cut-out square.
- Subtract the area of the smaller square from the area of the larger square.
3. Using Coordinates (For Grid-Based Figures)**
If your composite figure is drawn on a grid, you can utilize the grid's coordinate system to calculate the area. This method often involves counting squares and parts of squares.
Steps:
- Count full squares: Count the number of complete squares within the composite figure.
- Estimate partial squares: For squares that are only partially within the figure, estimate their fractional contribution to the total area. This requires visual judgment and might introduce some error.
- Sum the areas: Add the number of full squares and the estimated areas of the partial squares. Each square has an area of 1 unit² (if each grid square is 1 unit by 1 unit).
Common Mistakes to Avoid
- Incorrectly identifying shapes: Carefully examine the figure to correctly identify the constituent shapes. A misidentification will lead to an incorrect area calculation.
- Forgetting units: Always include the appropriate square units (cm², m², in², etc.) in your final answer.
- Using the wrong formula: Double-check that you're using the correct formula for each shape's area.
- Rounding errors: When dealing with π or square roots, be mindful of rounding errors. Carry out calculations to a sufficient number of decimal places before rounding your final answer.
- Ignoring partial shapes: When using the grid method, accurately estimate the area of any partial squares.
Area of Composite Figures Worksheet PDF: Where to Find Practice Problems
While I cannot directly provide a PDF worksheet, you can easily find numerous free resources online by searching for "area of composite figures worksheet pdf" on a search engine. Many educational websites and online resources offer printable worksheets with varying difficulty levels, catering to different grade levels and skill sets. These worksheets usually provide diagrams of composite figures and require you to calculate their areas, often providing space for working out the solutions.
Advanced Applications and Extensions
Understanding area of composite figures is crucial for many real-world applications. Consider these examples:
- Construction and Design: Architects and engineers use these calculations for determining the surface area of buildings, the amount of material needed for construction projects, and more.
- Land Measurement: Surveyors use these principles to calculate land areas for property valuation and development.
- Art and Design: Artists and designers use these concepts for creating and scaling artwork, and calculating the amount of materials needed.
- Computer Graphics: These calculations are fundamental in computer graphics and game development, used in creating 2D and 3D shapes and textures.
Conclusion
Mastering the calculation of the area of composite figures is a valuable skill with broad applications. By carefully decomposing the figures into simpler shapes, using the appropriate formulas, and carefully executing the calculations, you can accurately determine the area of even complex shapes. Remember to practice regularly using worksheets and other resources, and don't hesitate to seek help or clarification when needed. Consistent practice will build your confidence and mastery in this crucial geometric concept. The resources available online offer a wealth of practice opportunities to solidify your understanding. With dedicated effort and the right approach, you can confidently tackle any problem involving the area of composite figures.
Latest Posts
Latest Posts
-
12 Weeks Is Equal To How Many Months
Apr 04, 2025
-
What Are The Factors Of 86
Apr 04, 2025
-
What Is The Factor Of 57
Apr 04, 2025
-
What Is The Greatest Common Factor Of 45 And 30
Apr 04, 2025
-
How Many Weeks In Four Months
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about Area Of Composite Figures Worksheet Pdf . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
