Factor 12y + 6 Using The Gcf
News Co
May 07, 2025 · 4 min read
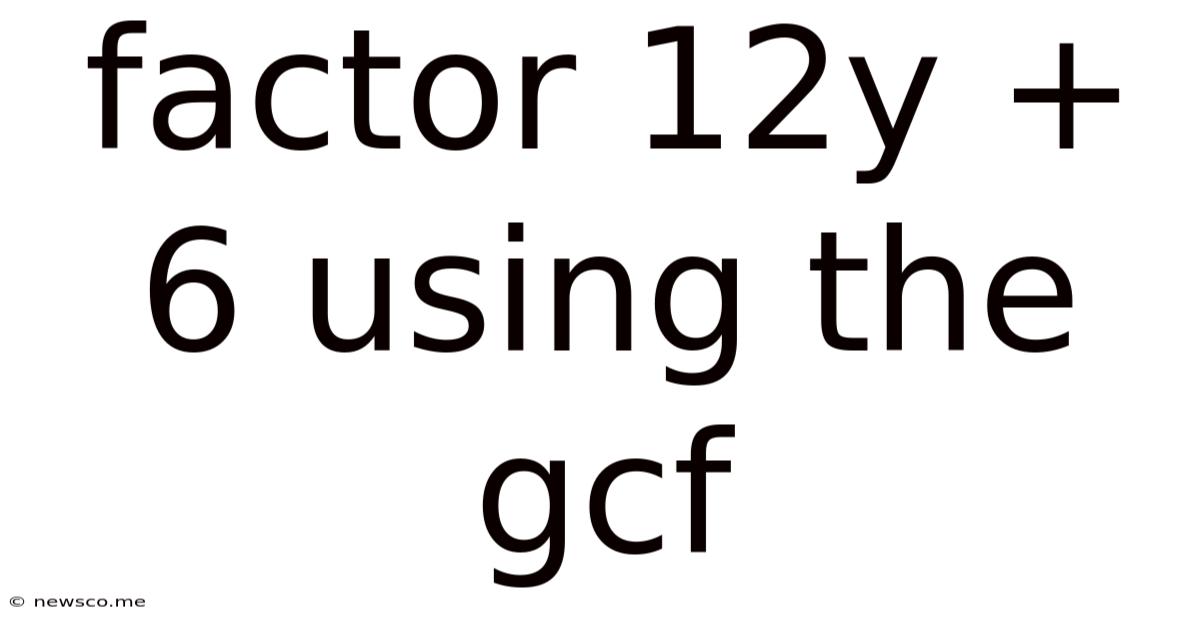
Table of Contents
Factoring 12y + 6 Using the Greatest Common Factor (GCF)
Factoring algebraic expressions is a fundamental skill in algebra. It involves breaking down a complex expression into simpler components, much like finding the prime factors of a number. This process is crucial for simplifying expressions, solving equations, and understanding more advanced algebraic concepts. One of the most basic factoring techniques involves identifying and extracting the greatest common factor (GCF). Let's explore this technique in detail using the expression 12y + 6 as our example.
Understanding the Greatest Common Factor (GCF)
Before we delve into factoring 12y + 6, let's clarify the concept of the greatest common factor. The GCF of two or more numbers or terms is the largest number or expression that divides evenly into all of them without leaving a remainder. Finding the GCF involves identifying the common factors and selecting the largest one.
For example, let's find the GCF of 12 and 6:
- Factors of 12: 1, 2, 3, 4, 6, 12
- Factors of 6: 1, 2, 3, 6
The common factors are 1, 2, 3, and 6. The largest of these is 6, so the GCF of 12 and 6 is 6.
Factoring 12y + 6: A Step-by-Step Guide
Now, let's apply this concept to factoring the algebraic expression 12y + 6.
Step 1: Identify the terms.
Our expression consists of two terms: 12y and 6.
Step 2: Find the GCF of the coefficients.
The coefficients are 12 and 6. As we determined earlier, the GCF of 12 and 6 is 6.
Step 3: Identify common variables (if any).
The first term, 12y, contains the variable 'y', while the second term, 6, does not. Therefore, there are no common variables.
Step 4: Extract the GCF.
Since the GCF is 6, we factor it out from both terms:
12y + 6 = 6(2y) + 6(1)
Notice that we've divided each term by the GCF (6). The results, 2y and 1, are placed within the parentheses.
Step 5: Write the factored expression.
Finally, we can write the factored expression as:
12y + 6 = 6(2y + 1)
This is the completely factored form of the expression 12y + 6 using the GCF. We've successfully broken down the original expression into its simplest components.
Verifying the Factorization
It's always a good practice to verify your factorization. To do this, we can expand the factored expression and see if we get back the original expression:
6(2y + 1) = 6 * 2y + 6 * 1 = 12y + 6
Since we've obtained the original expression, our factorization is correct.
Applying the GCF Factoring Technique to Other Expressions
The GCF factoring technique can be applied to various algebraic expressions. Let's consider a few more examples:
Example 1: Factoring 15x² + 25x
- Terms: 15x² and 25x
- GCF of coefficients: GCF(15, 25) = 5
- Common variable: x (the lowest power of x present in both terms is x¹)
- GCF: 5x
- Factoring: 15x² + 25x = 5x(3x + 5)
Example 2: Factoring 8a³b² - 12a²b³ + 4ab
- Terms: 8a³b², -12a²b³, 4ab
- GCF of coefficients: GCF(8, -12, 4) = 4
- Common variables: a (lowest power: a¹) and b (lowest power: b¹)
- GCF: 4ab
- Factoring: 8a³b² - 12a²b³ + 4ab = 4ab(2a²b - 3ab² + 1)
Example 3: Factoring 21m²n³ + 35m⁴n²
- Terms: 21m²n³, 35m⁴n²
- GCF of coefficients: GCF(21, 35) = 7
- Common variables: m² and n²
- GCF: 7m²n²
- Factoring: 21m²n³ + 35m⁴n² = 7m²n²(3n + 5m²)
Advanced Applications of GCF Factoring
While GCF factoring is a basic technique, it forms the foundation for more complex factoring methods. Often, after extracting the GCF, you might find that the remaining expression can be factored further using other techniques like difference of squares or trinomial factoring.
For example, consider the expression 2x² - 8. First, we extract the GCF, which is 2:
2x² - 8 = 2(x² - 4)
Now notice that (x² - 4) is a difference of squares (x² - 2²). This can be factored further:
2(x² - 4) = 2(x - 2)(x + 2)
Thus, the complete factorization of 2x² - 8 is 2(x - 2)(x + 2).
Importance of Factoring in Algebra
Factoring is a cornerstone of algebra, playing a crucial role in various algebraic operations:
- Simplifying Expressions: Factoring allows us to reduce complex expressions to simpler forms, making them easier to understand and manipulate.
- Solving Equations: Many algebraic equations require factoring to find solutions. For instance, quadratic equations are often solved by factoring.
- Understanding Functions: Factoring helps analyze the behavior of functions, particularly finding their roots (x-intercepts) and understanding their characteristics.
- Advanced Algebra: Factoring is essential for more advanced algebraic topics such as partial fraction decomposition, solving polynomial equations, and working with rational expressions.
Conclusion
Factoring algebraic expressions using the greatest common factor is a fundamental algebraic skill. By systematically identifying the GCF and extracting it from each term, we can effectively simplify expressions and lay the groundwork for more advanced factoring techniques. Mastering GCF factoring is crucial for success in algebra and beyond. Regular practice and a thorough understanding of the process will significantly improve your algebraic proficiency. Remember to always check your factorization by expanding the factored expression to ensure it matches the original expression. This verification step is essential to avoid errors and build confidence in your factoring abilities.
Latest Posts
Latest Posts
-
How Much Is 1700 Pounds In Us Dollars
May 08, 2025
-
How To Solve X 2 5
May 08, 2025
-
How Many Fluid Ounces Are In 1 L
May 08, 2025
-
V With A Line Through It
May 08, 2025
-
Are Isosceles Triangles Always Acute Triangles
May 08, 2025
Related Post
Thank you for visiting our website which covers about Factor 12y + 6 Using The Gcf . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.