First Derivative Test And Second Derivative Test
News Co
Apr 01, 2025 · 5 min read
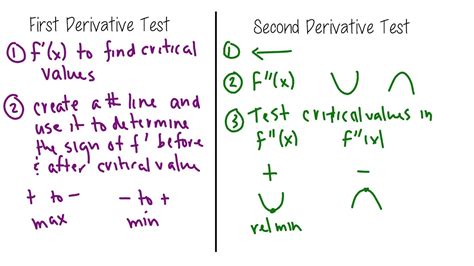
Table of Contents
First Derivative Test and Second Derivative Test: A Comprehensive Guide
Determining the characteristics of a function, such as its increasing and decreasing intervals, local maxima and minima, and concavity, is crucial in calculus. Two powerful tools for achieving this are the First Derivative Test and the Second Derivative Test. While both tests help analyze a function's behavior, they differ in their approach and application. This comprehensive guide will delve into both tests, providing clear explanations, practical examples, and insights into their limitations.
Understanding the First Derivative Test
The First Derivative Test utilizes the sign of the first derivative, f'(x), to identify critical points and classify them as local maxima, local minima, or neither. A critical point occurs where the derivative is zero (f'(x) = 0) or undefined. The test hinges on the principle that:
- f'(x) > 0: The function f(x) is increasing.
- f'(x) < 0: The function f(x) is decreasing.
The sign change of the first derivative around a critical point indicates the nature of that point.
Steps in Applying the First Derivative Test:
- Find the first derivative: Calculate f'(x).
- Find critical points: Solve f'(x) = 0 or identify where f'(x) is undefined.
- Test intervals: Determine the sign of f'(x) in intervals created by the critical points. This often involves choosing a test point within each interval and evaluating f'(x) at that point. A positive result indicates an increasing interval, while a negative result indicates a decreasing interval.
- Classify critical points: Analyze the sign changes of f'(x) around each critical point:
- Local Maximum: If f'(x) changes from positive to negative.
- Local Minimum: If f'(x) changes from negative to positive.
- Neither: If there is no sign change.
Example: Applying the First Derivative Test
Let's consider the function f(x) = x³ - 3x + 2.
-
First Derivative: f'(x) = 3x² - 3
-
Critical Points: Set f'(x) = 0: 3x² - 3 = 0 => x² = 1 => x = ±1.
-
Test Intervals: We have three intervals: (-∞, -1), (-1, 1), and (1, ∞).
- Interval (-∞, -1): Let's choose x = -2. f'(-2) = 3(-2)² - 3 = 9 > 0. The function is increasing.
- Interval (-1, 1): Let's choose x = 0. f'(0) = -3 < 0. The function is decreasing.
- Interval (1, ∞): Let's choose x = 2. f'(2) = 9 > 0. The function is increasing.
-
Classification:
- At x = -1, f'(x) changes from positive to negative. Therefore, x = -1 is a local maximum.
- At x = 1, f'(x) changes from negative to positive. Therefore, x = 1 is a local minimum.
Understanding the Second Derivative Test
The Second Derivative Test provides an alternative method for classifying critical points. It uses the sign of the second derivative, f''(x), at a critical point. The test is based on the concept of concavity:
- f''(x) > 0: The function is concave up (like a U).
- f''(x) < 0: The function is concave down (like an upside-down U).
Steps in Applying the Second Derivative Test:
- Find the first derivative: Calculate f'(x).
- Find critical points: Solve f'(x) = 0.
- Find the second derivative: Calculate f''(x).
- Evaluate the second derivative at critical points: Substitute each critical point into f''(x).
- Local Minimum: If f''(x) > 0.
- Local Maximum: If f''(x) < 0.
- Inconclusive: If f''(x) = 0. The Second Derivative Test fails; you must use the First Derivative Test.
Example: Applying the Second Derivative Test
Let's use the same function as before: f(x) = x³ - 3x + 2.
-
First Derivative: f'(x) = 3x² - 3
-
Critical Points: x = ±1 (as determined earlier).
-
Second Derivative: f''(x) = 6x
-
Evaluation:
- At x = -1: f''(-1) = -6 < 0. Therefore, x = -1 is a local maximum.
- At x = 1: f''(1) = 6 > 0. Therefore, x = 1 is a local minimum.
Comparing the First and Second Derivative Tests
Both tests serve the same purpose – classifying critical points. However, they have distinct advantages and disadvantages:
First Derivative Test:
- Advantages: Always works (even when the second derivative test is inconclusive). Provides a complete picture of increasing/decreasing intervals.
- Disadvantages: Requires more steps; involves testing intervals which can be more computationally intensive.
Second Derivative Test:
- Advantages: Often simpler and quicker than the First Derivative Test, especially if the second derivative is easy to compute.
- Disadvantages: Can be inconclusive (when f''(x) = 0), forcing the use of the First Derivative Test. Doesn't provide information about increasing/decreasing intervals.
Advanced Applications and Considerations
The First and Second Derivative Tests are fundamental tools with broader applications beyond simply finding local extrema. They play crucial roles in:
- Optimization problems: Finding maximum or minimum values within constraints.
- Curve sketching: Understanding the shape and behavior of a function for accurate graphing.
- Analyzing physical phenomena: Modeling and interpreting the behavior of systems described by mathematical functions.
Dealing with Inconclusive Cases
As mentioned, the Second Derivative Test is inconclusive when f''(x) = 0 at a critical point. In such scenarios, the First Derivative Test is essential. Furthermore, even when the second derivative test yields a result, it's often prudent to verify the findings with the First Derivative Test for complete assurance.
Functions with Undefined Derivatives
Both tests require the existence of the derivatives. For functions with points where the derivative is undefined, the First Derivative Test is still applicable by carefully analyzing the behavior of the function around those points. However, the Second Derivative Test may not be directly usable.
Higher-Order Derivatives
While we focus on the first and second derivatives, higher-order derivatives can provide additional information about a function's behavior, such as inflection points (where concavity changes).
Conclusion
The First and Second Derivative Tests are indispensable tools in calculus for understanding the behavior of functions. While the Second Derivative Test offers a potentially faster approach, the First Derivative Test provides a more robust and comprehensive analysis, particularly when dealing with inconclusive cases or functions with undefined derivatives. Mastering both tests equips you with a powerful arsenal for tackling diverse calculus problems and gaining a deeper understanding of function analysis. Remember to choose the most appropriate test based on the specific problem and the nature of the function. Using both tests in tandem often provides the most complete and reliable results.
Latest Posts
Latest Posts
-
Is 41 Prime Or Composite Number
Apr 02, 2025
-
What Is The Gcf Of 48 And 54
Apr 02, 2025
-
8 Of 20 Is What Percent
Apr 02, 2025
-
The Volume Of The Pyramid Abcd
Apr 02, 2025
-
Lcm Of 8 6 And 3
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about First Derivative Test And Second Derivative Test . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
