How Many Cubic Units Is A Box 3x3x2
News Co
May 08, 2025 · 5 min read
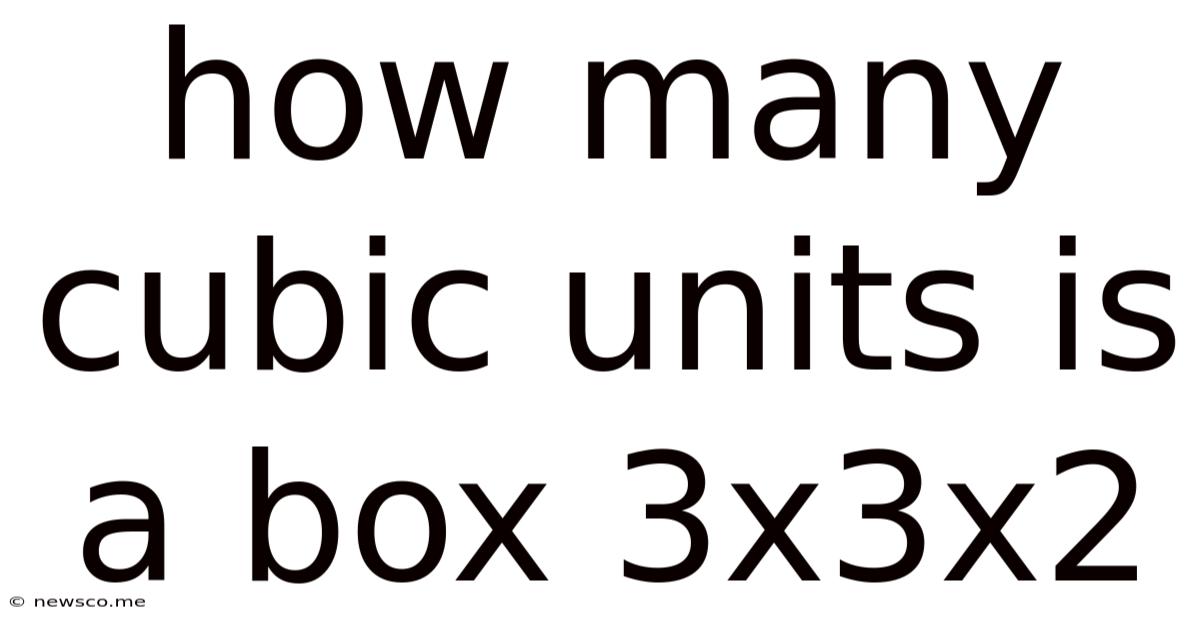
Table of Contents
How Many Cubic Units is a Box 3x3x2? A Deep Dive into Volume Calculation
This seemingly simple question, "How many cubic units is a box 3x3x2?", opens the door to a fascinating exploration of volume calculation, its applications, and even some surprising connections to other mathematical concepts. While the answer itself is straightforward, understanding the underlying principles is crucial for anyone working with three-dimensional shapes, whether in geometry class, engineering projects, or everyday life.
Let's delve into the specifics, addressing not only the immediate answer but also the broader context of volume calculation and its practical implications.
Understanding Volume: The Foundation
Volume, in its simplest form, measures the amount of three-dimensional space occupied by an object. It's expressed in cubic units (cubic centimeters, cubic meters, cubic feet, etc.), reflecting the three-dimensional nature of the measurement. Think of filling the box with tiny cubes—each one representing a single cubic unit. The total number of these cubes needed to completely fill the box gives you its volume.
Calculating the Volume of a Rectangular Prism (Box)
A box, in mathematical terms, is typically referred to as a rectangular prism. This is a three-dimensional shape with six rectangular faces, where opposite faces are parallel and congruent. Calculating its volume is remarkably simple:
Volume = Length x Width x Height
In our case, we have a box with:
- Length = 3 units
- Width = 3 units
- Height = 2 units
Therefore, the volume is:
Volume = 3 units x 3 units x 2 units = 18 cubic units
So, the answer to our initial question is 18 cubic units.
Beyond the Simple Calculation: Practical Applications
While the calculation itself is elementary, the concept of volume has far-reaching applications in numerous fields:
-
Engineering and Construction: Calculating the volume of materials is crucial for estimating quantities needed for construction projects (concrete, bricks, etc.), designing pipelines and reservoirs, and determining the capacity of containers. Understanding volume is fundamental in structural engineering, where the load-bearing capacity of a building is directly linked to its volume and the materials used.
-
Packaging and Logistics: Businesses rely heavily on accurate volume calculations for efficient packaging and shipping. Optimizing the size and shape of packaging minimizes waste and shipping costs, directly impacting profitability. Accurate volume calculations are essential for determining the shipping volume and associated fees.
-
Manufacturing and Production: In manufacturing, understanding volume is crucial for producing the correct quantity of goods, determining the capacity of machinery, and managing inventory. Accurate volume measurements ensure that production lines operate smoothly and meet demands.
-
Medicine and Healthcare: Volume calculations are essential in many medical applications, such as determining the dosage of medications, calculating blood volume, and analyzing the capacity of organs.
-
Environmental Science: Volume calculations are crucial in environmental studies, such as determining the volume of pollutants in water bodies, measuring the capacity of reservoirs, and analyzing the impact of deforestation on water storage.
Extending the Concept: Irregular Shapes and Complex Volumes
While calculating the volume of a rectangular prism is straightforward, many real-world objects have irregular shapes. Calculating their volume requires more sophisticated techniques. These methods often involve:
-
Water Displacement: Submerging an object in water and measuring the displaced water's volume provides an indirect measure of the object's volume. This method is commonly used for irregularly shaped objects.
-
Integration (Calculus): For more complex shapes, integral calculus provides a powerful tool for calculating volumes. By breaking down the object into infinitely small slices and summing their volumes, extremely accurate estimations can be achieved.
-
Numerical Methods: Computer-aided design (CAD) software and numerical methods allow for precise volume calculations of intricate shapes, often used in engineering and design applications.
Connecting to Other Mathematical Concepts
The concept of volume is deeply interconnected with other mathematical ideas:
-
Surface Area: While related, surface area (the total area of the object's outer surfaces) is distinct from volume. A larger surface area doesn't necessarily mean a larger volume, and vice versa. Understanding both concepts is important for a complete understanding of three-dimensional shapes.
-
Density: Density relates mass and volume. It's defined as mass per unit volume (e.g., grams per cubic centimeter). Knowing the volume and density allows for calculating the mass of an object.
-
Geometry and Trigonometry: Understanding various geometric shapes and principles of trigonometry is often necessary for calculating the volumes of complex objects.
Beyond Cubic Units: Different Units of Measurement
The choice of unit for volume depends on the scale of the object being measured. Common units include:
- Cubic Centimeters (cm³): Often used for smaller objects.
- Cubic Meters (m³): Used for larger objects or spaces.
- Cubic Feet (ft³): Common in many engineering and construction applications.
- Cubic Inches (in³): Used for smaller, more precise measurements.
- Liters (L) and Milliliters (mL): Commonly used for liquid volumes, with 1 liter equal to 1000 cubic centimeters. Gallons are used in the United States.
Error Analysis and Precision
In practical applications, it's crucial to consider potential sources of error in volume calculations. These errors can arise from:
- Measurement Inaccuracies: Imperfect measurements of length, width, and height will lead to errors in the calculated volume.
- Shape Irregularities: Real-world objects may not be perfectly rectangular prisms, leading to discrepancies between the calculated and actual volume.
- Rounding Errors: Rounding off numbers during calculations can accumulate errors, especially when dealing with many calculations.
Conclusion: The Importance of Understanding Volume
The seemingly simple problem of calculating the volume of a 3x3x2 box reveals a wealth of mathematical concepts and practical applications. While the answer, 18 cubic units, is readily obtained, a deeper understanding of volume calculation, its diverse applications, and its connections to other mathematical concepts is essential for anyone working with three-dimensional shapes and quantities. From engineering designs to everyday tasks, the ability to accurately determine and utilize volume is a fundamental skill with far-reaching implications. The understanding of volume calculation expands beyond simple geometry; it becomes a cornerstone in various fields, impacting efficiency, accuracy, and problem-solving capabilities across various disciplines.
Latest Posts
Latest Posts
-
Can Sample Variance Be Equal To Population Variance
May 09, 2025
-
Events That Cannot Occur At The Same Time Are Called
May 09, 2025
-
What Is 10 4 As A Decimal
May 09, 2025
-
What Does Area Under The Curve Mean In Statistics
May 09, 2025
-
8 1 4 As A Fraction
May 09, 2025
Related Post
Thank you for visiting our website which covers about How Many Cubic Units Is A Box 3x3x2 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.