Is 4 A Factor Of 18
News Co
Apr 07, 2025 · 5 min read
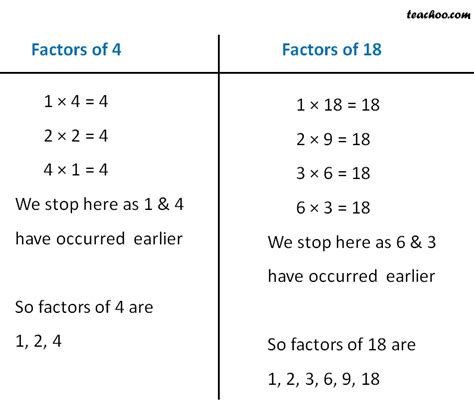
Table of Contents
Is 4 a Factor of 18? A Deep Dive into Factors, Multiples, and Divisibility
The question, "Is 4 a factor of 18?" might seem simple at first glance. However, understanding the underlying concepts of factors, multiples, and divisibility allows us to not only answer this specific question definitively but also build a strong foundation in number theory. This exploration will delve into these concepts, providing a comprehensive understanding of factors and how to determine whether one number is a factor of another. We'll even touch upon practical applications of this knowledge.
Understanding Factors and Multiples
Before we tackle the central question, let's define some key terms:
Factors: A factor of a number is a whole number that divides evenly into that number without leaving a remainder. In simpler terms, if you can divide a number by a factor, the result is a whole number. For example, the factors of 12 are 1, 2, 3, 4, 6, and 12. Notice that each of these numbers divides 12 without leaving a remainder.
Multiples: A multiple of a number is the result of multiplying that number by any whole number. For instance, the multiples of 3 are 3, 6, 9, 12, 15, and so on. Each of these numbers is obtained by multiplying 3 by a whole number (3 x 1, 3 x 2, 3 x 3, and so on).
Divisibility: Divisibility refers to the property of one number being completely divisible by another without leaving a remainder. We often use divisibility rules to quickly determine if a number is divisible by another. For example, a number is divisible by 2 if it's even (ends in 0, 2, 4, 6, or 8), and a number is divisible by 3 if the sum of its digits is divisible by 3.
Determining if 4 is a Factor of 18
Now, let's address the core question: Is 4 a factor of 18?
To determine if 4 is a factor of 18, we need to see if 18 can be divided by 4 without leaving a remainder. Let's perform the division:
18 ÷ 4 = 4 with a remainder of 2.
Since the division results in a remainder, 4 is not a factor of 18. This means that 4 does not divide evenly into 18.
Exploring the Factors of 18
Let's find all the factors of 18 to further illustrate the concept:
We can systematically check each whole number from 1 up to 18 to see which ones divide 18 evenly:
- 18 ÷ 1 = 18
- 18 ÷ 2 = 9
- 18 ÷ 3 = 6
- 18 ÷ 6 = 3
- 18 ÷ 9 = 2
- 18 ÷ 18 = 1
Therefore, the factors of 18 are 1, 2, 3, 6, 9, and 18. Notice that 4 is not included in this list.
Prime Factorization and Factors
Understanding prime factorization can also help us determine factors. Prime factorization is the process of expressing a number as the product of its prime factors (numbers divisible only by 1 and themselves).
The prime factorization of 18 is 2 x 3 x 3 or 2 x 3². Since 4 (which is 2²) is not present in the prime factorization of 18, it cannot be a factor.
Practical Applications of Factors and Divisibility
The concepts of factors and divisibility are not just theoretical; they have many practical applications in various fields:
-
Mathematics: Factors and multiples are fundamental in arithmetic, algebra, and number theory. They are essential for simplifying fractions, finding common denominators, and solving various mathematical problems.
-
Computer Science: In computer programming, divisibility checks are frequently used in algorithms and data structures. For example, determining if a number is even or odd is a basic divisibility test (divisible by 2).
-
Engineering: Engineers use divisibility and factors in designing structures, calculating measurements, and optimizing systems. For example, determining the number of units needed to build a structure or calculating the required material based on even division.
-
Everyday Life: We unconsciously use the concept of factors and divisibility in everyday scenarios. For example, dividing a pizza equally among friends (dividing the number of slices by the number of friends) or arranging items in rows and columns (finding factors to evenly distribute items).
Advanced Concepts: Greatest Common Factor (GCF) and Least Common Multiple (LCM)
Understanding factors also lays the groundwork for more advanced concepts like the Greatest Common Factor (GCF) and the Least Common Multiple (LCM).
-
Greatest Common Factor (GCF): The GCF of two or more numbers is the largest number that divides evenly into all of them. Finding the GCF is crucial in simplifying fractions and solving problems involving ratios and proportions.
-
Least Common Multiple (LCM): The LCM of two or more numbers is the smallest number that is a multiple of all of them. The LCM is essential in adding or subtracting fractions with different denominators.
Both GCF and LCM calculations rely heavily on understanding factors and prime factorization.
Conclusion: Why 4 is not a Factor of 18
In conclusion, the answer to the question "Is 4 a factor of 18?" is definitively no. We demonstrated this through direct division, which yielded a remainder, and by examining the prime factorization of 18, which doesn't contain 4 as a factor. Understanding the concepts of factors, multiples, divisibility, and related advanced concepts like GCF and LCM is crucial for various mathematical applications and real-world problem-solving. This exploration has not only answered the initial question but also broadened our understanding of fundamental number theory. The ability to identify factors efficiently and confidently is a valuable skill in mathematics and beyond. Remember to practice these concepts, and you'll build a strong foundation in number theory.
Latest Posts
Latest Posts
-
What Is The Next Number In The Sequence 931 1 3
Apr 08, 2025
-
How Do You Spell 60 In Words
Apr 08, 2025
-
Multiplication And Division Worksheets Grade 5 Pdf
Apr 08, 2025
-
What Is 3 To The 3 Power
Apr 08, 2025
-
Find The Centroid Of The Region Bounded By
Apr 08, 2025
Related Post
Thank you for visiting our website which covers about Is 4 A Factor Of 18 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.