Is 6 A Multiple Of 3
News Co
May 08, 2025 · 5 min read
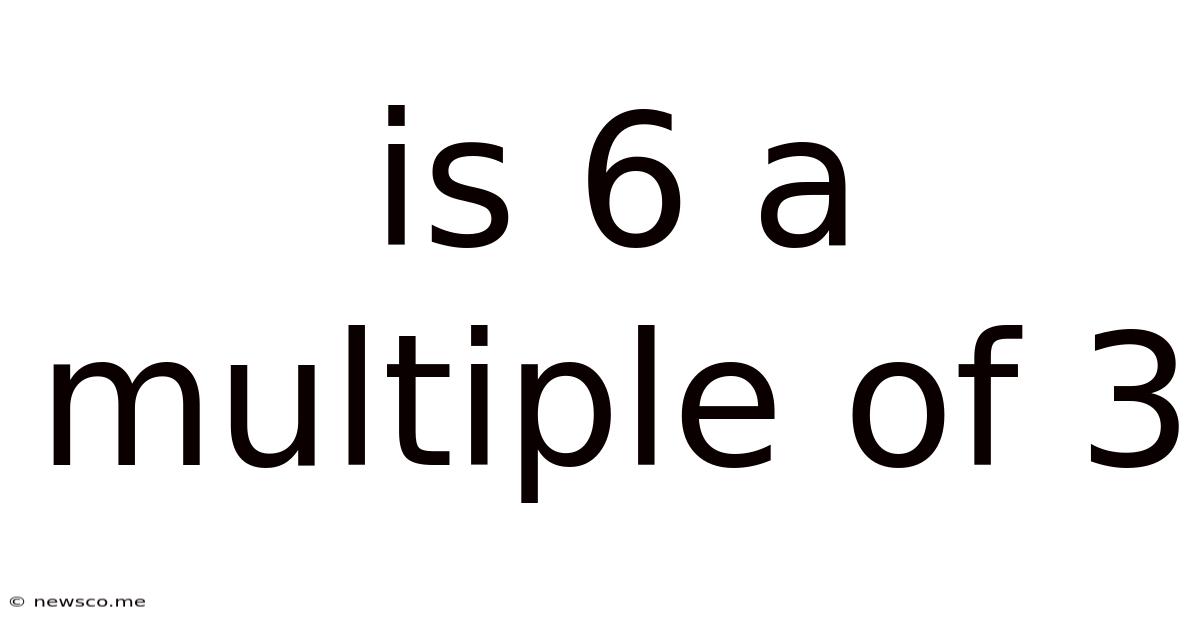
Table of Contents
Is 6 a Multiple of 3? A Deep Dive into Divisibility and Factors
The question, "Is 6 a multiple of 3?" might seem trivial at first glance. For many, the answer is immediately apparent. However, exploring this seemingly simple question allows us to delve into fundamental concepts in mathematics, specifically divisibility rules, factors, multiples, and their significance in various mathematical operations and applications. This article will not only answer the question definitively but will also provide a comprehensive understanding of the underlying principles.
Understanding Multiples and Divisibility
Before we address the specific question, let's define key terms:
Multiple: A multiple of a number is the product of that number and any integer (a whole number). For example, multiples of 3 are 3 (3 x 1), 6 (3 x 2), 9 (3 x 3), 12 (3 x 4), and so on. Essentially, multiples are the results of repeated addition of a number.
Divisibility: Divisibility refers to whether one number can be divided by another number without leaving a remainder. If a number is divisible by another, it means the second number is a factor of the first. For instance, 6 is divisible by 3 because 6 ÷ 3 = 2 with no remainder.
Factor: A factor is a number that divides another number without leaving a remainder. In other words, factors are numbers that can be multiplied together to produce a given number. The factors of 6 are 1, 2, 3, and 6 because 1 x 6 = 6 and 2 x 3 = 6.
Answering the Question: Is 6 a Multiple of 3?
Yes, 6 is definitively a multiple of 3. This is because 6 can be obtained by multiplying 3 by an integer: 3 x 2 = 6. Conversely, 3 is a factor of 6, and 6 is divisible by 3. This simple fact demonstrates a fundamental relationship between multiples, divisibility, and factors.
The Significance of Divisibility Rules
Understanding divisibility rules can significantly speed up calculations and simplify problem-solving. Divisibility rules are shortcuts that help determine if a number is divisible by another without performing the actual division.
For the number 3, the divisibility rule states: A number is divisible by 3 if the sum of its digits is divisible by 3.
Let's apply this rule to the number 6:
- The sum of the digits of 6 is 6 (since there's only one digit).
- 6 is divisible by 3 (6 ÷ 3 = 2).
- Therefore, 6 is divisible by 3, confirming that 6 is a multiple of 3.
This rule applies to larger numbers as well. For example, consider the number 123:
- The sum of the digits is 1 + 2 + 3 = 6.
- 6 is divisible by 3.
- Therefore, 123 is divisible by 3, making 123 a multiple of 3.
Applications of Multiples and Divisibility
The concepts of multiples and divisibility are not merely abstract mathematical ideas; they have wide-ranging applications in various fields:
1. Number Theory and Algebra:
Divisibility plays a crucial role in number theory, forming the basis for concepts like prime numbers, greatest common divisors (GCD), and least common multiples (LCM). These concepts are fundamental in simplifying fractions, solving equations, and understanding the structure of numbers.
2. Real-World Applications:
- Measurement and Units: Divisibility is essential in converting units of measurement. For example, converting inches to feet (12 inches = 1 foot) relies on the divisibility of 12 by 3.
- Scheduling and Planning: Multiples are used in creating schedules and organizing events. For instance, if a particular task takes 3 hours, scheduling multiple instances of this task will require using multiples of 3 (3 hours, 6 hours, 9 hours, etc.).
- Pattern Recognition: Recognizing patterns and sequences often involves understanding multiples. This is applicable in areas like data analysis, cryptography, and computer science.
- Geometry and Area Calculations: Calculating areas of shapes often involves using multiples and factors. For instance, finding the area of a rectangle with dimensions 3 and 6 requires understanding the relationship between 3 and 6.
3. Computer Science:
Multiples and divisibility are extensively used in algorithms and data structures. Hashing techniques, for example, rely on modular arithmetic, which is based on divisibility and remainders.
Beyond the Basics: Exploring Prime Factorization
Prime factorization is a powerful tool for understanding the structure of numbers and is directly related to divisibility and multiples. Prime factorization involves expressing a number as a product of its prime factors (numbers divisible only by 1 and themselves).
Let's look at the prime factorization of 6:
6 = 2 x 3
This factorization shows that 6 is composed of the prime factors 2 and 3. This reveals that 6 is divisible by 2 and 3, and that both 2 and 3 are factors of 6. The prime factorization method offers a systematic way to determine all the factors of a number.
Common Misconceptions about Multiples
While the concept of multiples is relatively straightforward, some common misconceptions can arise:
- Confusing Multiples and Factors: Students sometimes confuse multiples and factors. Remember, multiples are the results of multiplication, while factors are numbers that divide evenly into a given number.
- Assuming Only Positive Multiples: Multiples can be positive or negative. For instance, -6 is also a multiple of 3 because 3 x (-2) = -6.
- Limited Understanding of Divisibility Rules: While divisibility rules are helpful shortcuts, it's important to understand their limitations and not rely solely on them.
Conclusion: The Importance of Foundational Mathematical Concepts
The seemingly simple question, "Is 6 a multiple of 3?" serves as a springboard to explore fundamental mathematical concepts like multiples, divisibility, factors, and prime factorization. These concepts are not merely abstract ideas but are essential tools used in various fields, highlighting their practical significance beyond the classroom. A thorough understanding of these concepts is crucial for success in mathematics and its diverse applications in the real world. Mastering these foundational principles will empower you to tackle more complex mathematical problems and appreciate the elegant structure of numbers. Furthermore, practicing these concepts strengthens problem-solving skills and enhances mathematical intuition. Remember to always revisit and reinforce these basic ideas as they form the bedrock for advanced mathematical exploration.
Latest Posts
Latest Posts
-
An Equation That States Two Ratios Are Equivalent
May 08, 2025
-
How Is 2 3 Not Always Equivalent To 4 6
May 08, 2025
-
Identify Which Of The Following Statements Is Correct
May 08, 2025
-
How To Find 3 4 Of A Number
May 08, 2025
-
Which Graph Shows An Odd Function
May 08, 2025
Related Post
Thank you for visiting our website which covers about Is 6 A Multiple Of 3 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.