What Is 1 3 Of 12000
News Co
May 04, 2025 · 4 min read
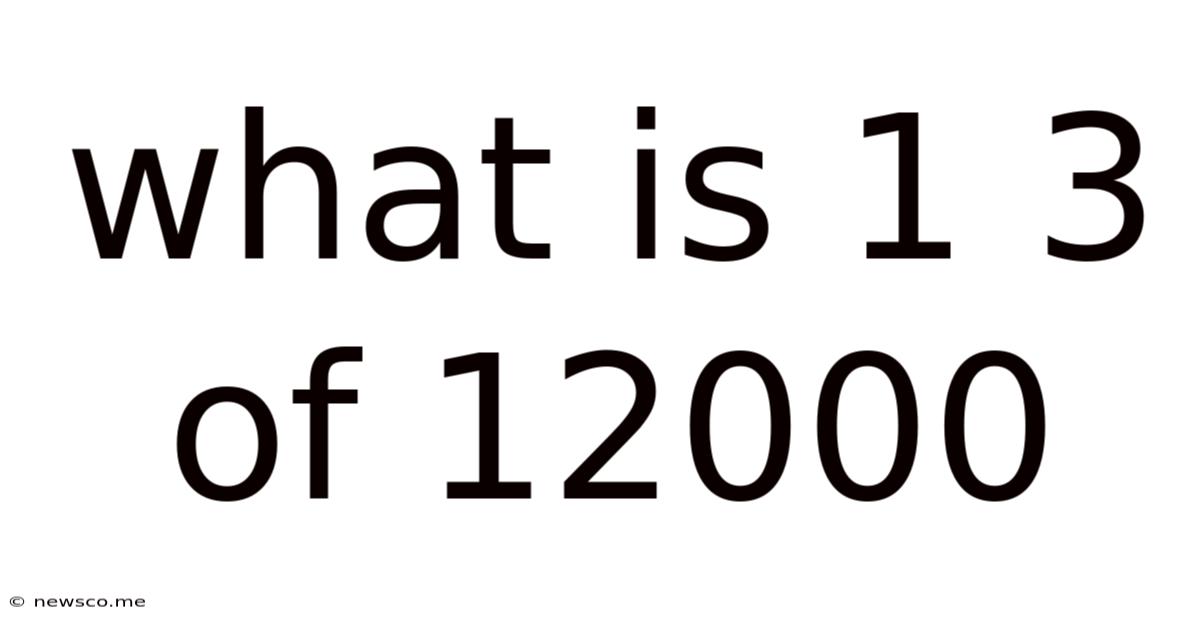
Table of Contents
What is 1/3 of 12000? A Deep Dive into Fractions and Their Applications
Finding a fraction of a number is a fundamental mathematical operation with widespread applications in everyday life, from calculating discounts and splitting bills to understanding proportions in various fields like finance, cooking, and construction. This article will explore the question, "What is 1/3 of 12000?", providing a comprehensive explanation of the solution, its underlying principles, and numerous real-world examples to solidify your understanding.
Understanding Fractions: A Refresher
Before we delve into the calculation, let's briefly revisit the concept of fractions. A fraction represents a part of a whole. It consists of two numbers: the numerator (the top number) and the denominator (the bottom number). The numerator indicates how many parts we have, and the denominator indicates how many equal parts the whole is divided into.
For instance, in the fraction 1/3, 1 is the numerator, and 3 is the denominator. This means we have 1 part out of a total of 3 equal parts.
Calculating 1/3 of 12000
To find 1/3 of 12000, we perform a simple multiplication:
(1/3) * 12000
This can be solved in two ways:
Method 1: Direct Multiplication
We can multiply the numerator (1) by 12000 and then divide the result by the denominator (3):
(1 * 12000) / 3 = 12000 / 3 = 4000
Therefore, 1/3 of 12000 is 4000.
Method 2: Simplification Before Multiplication
Sometimes, simplification can make the calculation easier. We can simplify the fraction before multiplying. Since 12000 is divisible by 3, we can do this:
12000 / 3 = 4000
Then, we multiply the simplified result by the numerator (1):
4000 * 1 = 4000
Both methods yield the same answer: 4000.
Real-World Applications: Where Fractions Matter
The ability to calculate fractions is essential in many real-world scenarios. Here are some examples demonstrating the practical application of finding 1/3 of a quantity:
1. Financial Planning and Budgeting:
- Investment Returns: If you invested $12,000 and earned a 1/3 return on your investment, you would have made a profit of $4,000.
- Expense Allocation: Imagine you have $12,000 for home renovations and plan to allocate 1/3 to kitchen upgrades. This means you would budget $4,000 for the kitchen.
- Debt Management: If you owe $12,000 and manage to pay off 1/3 of the debt, you would have reduced your debt by $4,000.
2. Cooking and Baking:
- Recipe Adjustments: A recipe calls for 12,000 grams of flour, but you only want to make 1/3 of the recipe. You would need 4,000 grams of flour.
- Ingredient Proportions: If a recipe requires ingredients in a ratio of 1:3 and you start with 4,000 grams of one ingredient, you would need 12,000 grams of the other.
3. Construction and Engineering:
- Material Calculations: If a construction project requires 12,000 bricks and only 1/3 of the project is completed, 4,000 bricks have been used.
- Land Division: If you own a 12,000 square-foot plot of land and want to sell 1/3 of it, you would sell 4,000 square feet.
4. Data Analysis and Statistics:
- Sample Size: If you have a dataset of 12,000 observations and you want to analyze a random sample representing 1/3 of the data, you would select a sample size of 4,000.
- Percentage Calculations: Calculating 1/3 of a total value allows for easy conversion to percentages. 1/3 represents 33.33% (approximately).
Beyond 1/3: Working with Other Fractions
The principles discussed above can be applied to any fraction. For example, to find 2/3 of 12000, you would:
- Find 1/3 of 12000 (which is 4000, as we have established).
- Multiply the result by the numerator of the fraction: 4000 * 2 = 8000
Therefore, 2/3 of 12000 is 8000.
Mastering Fractions: A Key to Mathematical Proficiency
The ability to work comfortably with fractions is crucial for success in various academic disciplines and everyday situations. Regular practice and understanding the underlying principles are essential for mastering this fundamental mathematical skill. This includes understanding equivalent fractions, simplifying fractions, adding, subtracting, multiplying, and dividing fractions.
Expanding Your Knowledge: Further Exploration
To deepen your understanding of fractions and their applications, consider exploring resources that cover topics such as:
- Ratio and Proportion: Understanding ratios helps you relate different quantities and solve problems involving proportional relationships.
- Percentages: Converting fractions to percentages and vice versa is a valuable skill for real-world applications.
- Decimals: Understanding the relationship between fractions and decimals further enhances your numerical fluency.
- Algebra: Applying fractions in algebraic equations and expressions is a key element of more advanced mathematics.
By mastering the concept of fractions and their applications, you equip yourself with a powerful tool for problem-solving in numerous areas of life, from personal finance to professional endeavors. The simple question of "What is 1/3 of 12000?" opens the door to a deeper understanding of a fundamental mathematical concept with far-reaching implications. Remember, consistent practice and exploration are key to developing a strong grasp of this valuable skill.
Latest Posts
Latest Posts
-
How Many Days Has It Been Since February 12
May 04, 2025
-
What Is The Interior Angle Of A Regular Decagon
May 04, 2025
-
7 Pi Over 6 In Degrees
May 04, 2025
-
How To Take The Integral Of An Absolute Value
May 04, 2025
-
What Is The Parent Function Of A Quadratic Function
May 04, 2025
Related Post
Thank you for visiting our website which covers about What Is 1 3 Of 12000 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.