What Is The Prime Factors Of 225
News Co
Apr 07, 2025 · 5 min read
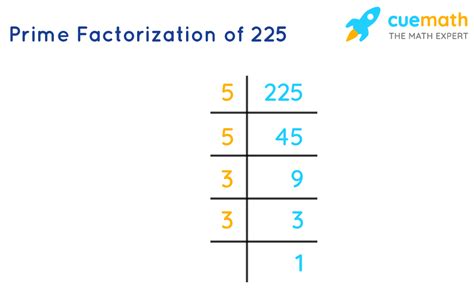
Table of Contents
What are the Prime Factors of 225? A Deep Dive into Prime Factorization
Finding the prime factors of a number might seem like a simple mathematical exercise, but understanding the process unlocks a deeper appreciation of number theory and its applications in various fields. This article will delve into the fascinating world of prime factorization, focusing specifically on the number 225. We'll explore not only the answer but also the underlying principles and methods used to arrive at the solution. We'll even touch upon the broader implications of prime factorization in areas like cryptography and computer science.
Understanding Prime Numbers and Prime Factorization
Before we tackle the prime factors of 225, let's establish a firm understanding of the key concepts involved.
What are Prime Numbers?
A prime number is a natural number greater than 1 that has no positive divisors other than 1 and itself. This means it's only divisible without a remainder by 1 and itself. The first few prime numbers are 2, 3, 5, 7, 11, 13, and so on. Note that 1 is not considered a prime number.
What is Prime Factorization?
Prime factorization (also known as prime decomposition) is the process of finding the prime numbers that, when multiplied together, equal the original number. Every composite number (a number that is not prime) can be expressed as a unique product of prime numbers. This uniqueness is guaranteed by the Fundamental Theorem of Arithmetic.
Finding the Prime Factors of 225: A Step-by-Step Approach
Now, let's determine the prime factors of 225. We'll use a common method called the factor tree.
-
Start with the smallest prime number: The smallest prime number is 2. However, 225 is an odd number, so it's not divisible by 2.
-
Try the next prime number: The next prime number is 3. Is 225 divisible by 3? Yes! 225 / 3 = 75.
-
Continue factoring: Now we have 3 x 75. 75 is also divisible by 3 (75 / 3 = 25).
-
Keep going until you reach prime numbers: We now have 3 x 3 x 25. 25 is not divisible by 3, but it is divisible by 5 (25 / 5 = 5).
-
Final result: We've reached only prime numbers: 3, 3, and 5, and 5. Therefore, the prime factorization of 225 is 3 x 3 x 5 x 5, which can also be written as 3² x 5².
Therefore, the prime factors of 225 are 3 and 5.
Alternative Methods for Prime Factorization
While the factor tree is a visually intuitive method, other approaches exist for finding prime factors.
Division Method
This method involves repeatedly dividing the number by the smallest prime number that divides it evenly until you are left with 1.
- Start with 225.
- Divide by 3: 225 / 3 = 75
- Divide by 3 again: 75 / 3 = 25
- Divide by 5: 25 / 5 = 5
- Divide by 5: 5 / 5 = 1
The prime factors are the numbers used in the divisions: 3, 3, 5, and 5.
Using Exponents
Once you've found the prime factors, it's common practice to express the factorization using exponents. This makes the representation more concise. In the case of 225, the prime factorization is 3² x 5². This clearly shows that 3 appears twice and 5 appears twice as factors.
The Significance of Prime Factorization
Prime factorization isn't just a mathematical curiosity; it has far-reaching applications in several fields:
Cryptography
Prime numbers play a crucial role in modern cryptography, particularly in public-key cryptography systems like RSA. The security of these systems relies on the difficulty of factoring very large numbers into their prime components. The larger the numbers, the more computationally intensive it becomes to find the prime factors, ensuring the confidentiality of sensitive information.
Computer Science
Prime factorization algorithms are important in computer science for various applications, including:
- Hashing: Prime numbers are often used in hash table algorithms to minimize collisions.
- Random Number Generation: Prime numbers are essential in generating pseudo-random numbers.
- Network Security: Prime numbers are used in various network security protocols.
Number Theory
Prime factorization is fundamental to number theory, a branch of mathematics that studies the properties of integers. Many important theorems and conjectures in number theory rely on the properties of prime numbers and their factorizations.
Beyond 225: Exploring Other Numbers
Understanding the prime factorization of 225 provides a solid foundation for tackling other numbers. Let's briefly look at some examples:
- 100: The prime factorization of 100 is 2² x 5².
- 150: The prime factorization of 150 is 2 x 3 x 5².
- 360: The prime factorization of 360 is 2³ x 3² x 5.
By practicing with different numbers, you'll become more proficient in identifying prime factors and appreciating the elegance of prime decomposition.
Conclusion: The Importance of Prime Factors
The prime factors of 225, 3 and 5, represent more than just a mathematical solution. They are building blocks of a number system with profound implications across various fields. Mastering prime factorization not only strengthens your mathematical skills but also provides insights into the fundamental principles underlying cryptography, computer science, and number theory. The seemingly simple process of finding the prime factors of a number opens doors to a deeper understanding of the structure and beauty of mathematics. Remember, practice makes perfect; the more you work with prime numbers and their factorizations, the more confident and adept you'll become.
Latest Posts
Latest Posts
-
What Number Is 10 Of 20
Apr 08, 2025
-
What Is 10 To The 23rd Power
Apr 08, 2025
-
How To Find The Nth Degree Polynomial Function
Apr 08, 2025
-
How Many Acres In A Square Mile Of Land
Apr 08, 2025
-
12 7 As A Mixed Number
Apr 08, 2025
Related Post
Thank you for visiting our website which covers about What Is The Prime Factors Of 225 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.