Area Of Composite Figures Worksheet Answers
News Co
Apr 05, 2025 · 5 min read
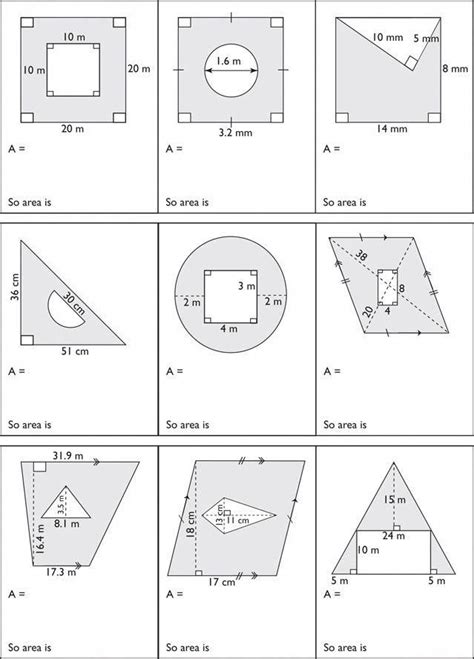
Table of Contents
Area of Composite Figures Worksheet Answers: A Comprehensive Guide
Finding the area of composite figures can be tricky, but mastering this skill is crucial for success in geometry and beyond. Composite figures are shapes formed by combining two or more basic geometric shapes like rectangles, triangles, circles, and semi-circles. This comprehensive guide will walk you through various methods to calculate the area of composite figures, provide examples, and offer strategies to tackle worksheet problems effectively. We'll even delve into common mistakes to avoid and provide tips for improving your problem-solving skills. Let's dive in!
Understanding Composite Figures
Before we tackle the calculations, it's essential to understand what composite figures are. They are essentially geometric puzzles, where simpler shapes are joined together to create a more complex one. Think of them as a collection of rectangles, squares, triangles, circles, or any combination thereof, all pieced together. This seemingly complex shape is actually just a sum of its simpler parts. The key to solving area problems involving composite figures is to decompose the figure into its constituent shapes.
Identifying Component Shapes
The first step in solving any composite figure area problem is to correctly identify the individual shapes that make up the larger figure. This requires careful observation and sometimes a bit of creativity. Look for familiar shapes within the composite figure. Can you see a rectangle, a triangle, a semicircle, or perhaps a combination of these? Drawing lines to separate the composite figure into its individual components often helps visualize the individual shapes.
Methods for Calculating Area
Once you've identified the component shapes, you can calculate their individual areas using the appropriate formulas. Let's review the area formulas for some common shapes:
1. Rectangle:
- Formula: Area = length × width
2. Square:
- Formula: Area = side × side (or side²)
3. Triangle:
- Formula: Area = (1/2) × base × height
4. Circle:
- Formula: Area = π × radius² (remember π ≈ 3.14159)
5. Semicircle:
- Formula: Area = (1/2) × π × radius²
Solving Area of Composite Figures: Step-by-Step Examples
Now let's apply these formulas to solve some example problems. Remember, the key is to break down the composite figure into its simpler parts.
Example 1: A Figure Composed of a Rectangle and a Triangle
Imagine a figure that looks like a house. The base is a rectangle with a length of 10 cm and a width of 5 cm. A triangle sits on top of the rectangle. The triangle has a base of 10 cm and a height of 4 cm.
-
Decompose: We have a rectangle and a triangle.
-
Calculate individual areas:
- Rectangle: Area = 10 cm × 5 cm = 50 cm²
- Triangle: Area = (1/2) × 10 cm × 4 cm = 20 cm²
-
Combine: Total Area = Area of Rectangle + Area of Triangle = 50 cm² + 20 cm² = 70 cm²
Example 2: A Figure Composed of a Rectangle and a Semicircle
Consider a figure shaped like a rectangle with a semicircle attached to one of its sides. The rectangle has a length of 8 cm and a width of 6 cm. The semicircle has a diameter of 6 cm (radius of 3 cm).
-
Decompose: We have a rectangle and a semicircle.
-
Calculate individual areas:
- Rectangle: Area = 8 cm × 6 cm = 48 cm²
- Semicircle: Area = (1/2) × π × (3 cm)² ≈ (1/2) × π × 9 cm² ≈ 14.14 cm²
-
Combine: Total Area = Area of Rectangle + Area of Semicircle ≈ 48 cm² + 14.14 cm² ≈ 62.14 cm²
Example 3: A More Complex Composite Figure
Let's tackle a more challenging example. Imagine a figure that's a combination of a large rectangle with a smaller rectangle cut out from the center. The large rectangle measures 12 cm by 8 cm, and the smaller rectangle cut out measures 4 cm by 3 cm.
-
Decompose: We have a large rectangle and a smaller rectangle (to be subtracted).
-
Calculate individual areas:
- Large Rectangle: Area = 12 cm × 8 cm = 96 cm²
- Small Rectangle: Area = 4 cm × 3 cm = 12 cm²
-
Combine (Subtraction): Total Area = Area of Large Rectangle - Area of Small Rectangle = 96 cm² - 12 cm² = 84 cm²
Common Mistakes to Avoid
Several common mistakes can lead to incorrect answers when calculating the area of composite figures. Here are some to watch out for:
- Incorrectly identifying shapes: Carefully examine the figure and ensure you correctly identify all the component shapes before proceeding with calculations.
- Using incorrect formulas: Double-check that you're using the appropriate area formula for each shape.
- Mixing up units: Maintain consistency in units throughout your calculations. If you start with centimeters, stick with centimeters.
- Forgetting to subtract areas: When a shape is cut out from another, remember to subtract the area of the smaller shape from the area of the larger shape.
- Rounding errors: Try to avoid rounding until the very end of your calculations to minimize errors.
Tips for Success
- Draw clear diagrams: Sketching the composite figure and clearly labeling its dimensions can significantly improve your understanding and reduce errors.
- Break down complex figures: Divide the figure into simpler shapes and solve each individually.
- Check your work: Always review your calculations to ensure accuracy. Compare your answer to your diagram to verify it makes sense.
- Practice regularly: The more you practice solving problems, the more confident and efficient you'll become.
Advanced Composite Figures and Problem Solving Strategies
As you progress, you'll encounter more complex composite figures involving irregular shapes. For these, you might need to utilize more advanced techniques, such as:
- Coordinate Geometry: Using coordinate points to define the vertices of shapes can help in determining lengths and areas, especially for irregular polygons.
- Approximation Methods: For very irregular shapes, approximation techniques like dividing the shape into smaller, simpler shapes, might be necessary.
Conclusion
Calculating the area of composite figures is a fundamental skill in geometry. By carefully identifying component shapes, applying the correct area formulas, and paying attention to detail, you can successfully solve even the most challenging problems. Remember to practice regularly, review your work, and don't be afraid to break down complex figures into manageable parts. With consistent effort, you’ll master this skill and confidently tackle any area of composite figures worksheet. Remember that practice is key! The more you work with these problems, the easier they will become. Good luck!
Latest Posts
Latest Posts
-
Whats The Square Root Of 27
Apr 05, 2025
-
How Much 70 Fahrenheit In Celsius
Apr 05, 2025
-
Sin And Cos And Tan Rules
Apr 05, 2025
-
What Is The Roman Numeral For 59
Apr 05, 2025
-
Express The Fractions 3 4 7 16 And 5 8 With The Lcd
Apr 05, 2025
Related Post
Thank you for visiting our website which covers about Area Of Composite Figures Worksheet Answers . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
