Common Multiples Of 17 And 13
News Co
Apr 04, 2025 · 5 min read
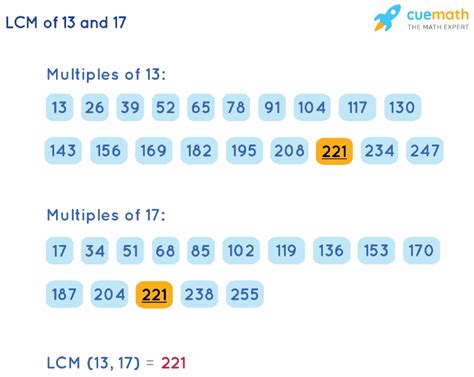
Table of Contents
Unveiling the Mysteries of Common Multiples: A Deep Dive into the Multiples of 17 and 13
Finding common multiples, especially for seemingly unrelated numbers like 17 and 13, might seem daunting at first. But with a systematic approach and a touch of mathematical understanding, we can unravel the intricacies of these common multiples and even develop strategies for finding them efficiently for any pair of numbers. This comprehensive guide will not only reveal the common multiples of 17 and 13 but also equip you with the tools to tackle similar problems confidently.
Understanding Multiples and Common Multiples
Before we delve into the specifics of 17 and 13, let's establish a solid foundation. A multiple of a number is the product of that number and any integer (whole number). For instance, multiples of 5 include 5 (5 x 1), 10 (5 x 2), 15 (5 x 3), and so on. These extend infinitely in both positive and negative directions.
A common multiple, as the name suggests, is a multiple shared by two or more numbers. For example, 12 is a common multiple of 3 and 4 because 3 x 4 = 12 and 4 x 3 = 12. Finding common multiples is a crucial concept in various areas of mathematics, from simplifying fractions to solving complex algebraic equations.
Finding the Least Common Multiple (LCM) of 17 and 13
The least common multiple (LCM) is the smallest positive common multiple of two or more numbers. Finding the LCM is often the first step in working with common multiples. Since 17 and 13 are both prime numbers (divisible only by 1 and themselves), finding their LCM is surprisingly straightforward.
Method 1: Prime Factorization
Prime factorization involves expressing a number as a product of its prime factors. Since 17 and 13 are already prime, their prime factorizations are simply 17 and 13, respectively. To find the LCM, we take the highest power of each prime factor present in the numbers. In this case:
- Prime factors of 17: 17¹
- Prime factors of 13: 13¹
Therefore, the LCM(17, 13) = 17 x 13 = 221.
Method 2: Using the Formula for Two Numbers
For two numbers, a and b, the LCM can be calculated using the formula:
LCM(a, b) = (a x b) / GCD(a, b)
where GCD(a, b) represents the greatest common divisor of a and b. Since 17 and 13 are prime and share no common factors other than 1, their GCD is 1. Therefore:
LCM(17, 13) = (17 x 13) / 1 = 221
This confirms our earlier result. The least common multiple of 17 and 13 is 221.
Generating Other Common Multiples of 17 and 13
The LCM is just the beginning. There are infinitely many other common multiples of 17 and 13. To find these, simply multiply the LCM by any positive integer.
- 221 x 1 = 221
- 221 x 2 = 442
- 221 x 3 = 663
- 221 x 4 = 884
- 221 x 5 = 1105
- and so on...
This sequence continues indefinitely. Each number in this sequence is a common multiple of both 17 and 13. You can verify this by dividing each number by 17 and 13; you'll always get an integer result.
Real-World Applications of Finding Common Multiples
The concept of common multiples, particularly the LCM, isn't confined to abstract mathematical exercises. It finds practical applications in various fields:
-
Scheduling: Imagine two buses that operate on different routes, one arriving at a stop every 17 minutes and the other every 13 minutes. The LCM (221 minutes) would tell you when both buses arrive at the stop simultaneously.
-
Project Management: If two tasks require 17 and 13 units of time, respectively, the LCM helps determine when both tasks could be completed at the same time, enabling efficient resource allocation.
-
Music Theory: Musical intervals and harmonies often rely on relationships between frequencies, where common multiples play a significant role in creating consonant sounds.
-
Manufacturing and Production: In manufacturing processes where machines operate at different cycles, common multiples can be utilized to optimize production schedules and minimize downtime.
Extending the Concept: Finding Common Multiples of More Than Two Numbers
The principles discussed above can be extended to find common multiples of more than two numbers. For instance, let's consider finding the LCM of 17, 13, and 5.
Method: Prime Factorization
-
Prime factorize each number:
- 17 = 17
- 13 = 13
- 5 = 5
-
Identify the highest power of each prime factor: 17¹, 13¹, and 5¹
-
Multiply these highest powers together: LCM(17, 13, 5) = 17 x 13 x 5 = 1105
Beyond the Basics: Exploring Advanced Concepts
While finding the LCM of relatively small numbers like 17 and 13 is relatively straightforward, the challenge increases when dealing with larger numbers or a greater number of numbers. For these scenarios, more advanced techniques such as the Euclidean algorithm for finding the GCD (which is crucial for the LCM calculation) become essential.
Conclusion: Mastering the Art of Common Multiples
Understanding common multiples, particularly the LCM, is a fundamental skill in mathematics with far-reaching practical applications. This detailed exploration of the common multiples of 17 and 13, combined with the explanation of different methods, equips you with the knowledge to tackle various problems involving common multiples. Remember, mastering this concept opens doors to understanding more complex mathematical concepts and their real-world applications, enriching your problem-solving abilities across diverse fields. Whether it's scheduling, project management, or even understanding musical harmony, the principles of common multiples are invaluable tools. So, embrace the power of these mathematical concepts and unlock their potential in your world.
Latest Posts
Latest Posts
-
What Are All The Factors For 40
Apr 05, 2025
-
Highest Common Factor Of 15 And 18
Apr 05, 2025
-
What Is The Square Root Of 512
Apr 05, 2025
-
What Is The Highest Common Factor Of 28 And 42
Apr 05, 2025
-
What Is 3 Out Of 5 As A Percentage
Apr 05, 2025
Related Post
Thank you for visiting our website which covers about Common Multiples Of 17 And 13 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
